n个数进栈，随机出栈，不同顺序数 catalan number

最新推荐文章于 2021-07-14 16:20:39 发布

life_liver

最新推荐文章于 2021-07-14 16:20:39 发布

阅读量1.9k

点赞数 1

文章标签：随机出栈catalan number

有n个数随机进栈，并且随机出栈，问有多少种不同的出栈顺序？

以下解答转自：http://blog.csdn.net/zyearn/article/details/7758716?reload

我们把n个元素的出栈个数的记为f(n), 那么对于1,2,3, 我们很容易得出

f(1) = 1 //即 1

f(2) = 2 //即 12、21

f(3) = 5 //即 123、132、213、321、231

然后我们来考虑f(4), 我们给4个元素编号为a,b,c,d, 那么考虑：元素a只可能出现在1号位置，2号位置，3号位置和4号位置(很容易理解，一共就4个位置，比如abcd,元素a就在1号位置)。

分析：

1) 如果元素a在1号位置，那么只可能a进栈，马上出栈，此时还剩元素b、c、d等待操作，就是子问题f(3)；

2) 如果元素a在2号位置，那么一定有一个元素比a先出栈，即有f(1)种可能顺序（只能是b），还剩c、d，即

f（2)，根据乘法原理，一共的顺序个数为f(1) * f(2)；

3) 如果元素a在3号位置，那么一定有两个元素比1先出栈，即有f(2)种可能顺序（只能是b、c），还剩d，即f(1)，

根据乘法原理，一共的顺序个数为f(2) * f(1)；

4) 如果元素a在4号位置，那么一定是a先进栈，最后出栈，那么元素b、c、d的出栈顺序即是此小问题的解，即

f(3)；

结合所有情况，即f(4) = f(3) + f(2) * f(1) + f(1) * f(2) + f(3);

为了规整化，我们定义f(0) = 1；于是f(4)可以重新写为：

f(4) = f(0)*f(3) + f(1)*f(2) + f(2) * f(1) + f(3)*f(0)

然后我们推广到n，推广思路和n=4时完全一样，于是我们可以得到：

f(n) = f(0)*f(n-1) + f(1)*f(n-2) + ... + f(n-1)*f(0)

即

但这只是一个递推公式（若编程实现，需要维护一个一维数组，时间复杂度为O(n^2)）。怎么把它转化为通项公式呢，复杂度仅为O(1)?

于是上网搜索一下，原来真的有这么一个公式：C(2n,n)/(n+1) （C(2n,n)表示2n里取n），并且有个名字叫Catalan数。附上wiki的链接，写得太详细了：http://en.wikipedia.org/wiki/Catalan_number，这里面有好多适合catalan数的例子，可以阅读下，等以后类似可以使用的catalan数的问题是可直接用catalan数得出结果，而不用去在逻辑方面推导。

现在的问题就是：怎么从上述的递推公式求出C(2n,n)/(n+1) ? 有兴趣的朋友欢迎留言讨论！在没有自己推导之前可以先记住这个结论：若

$C_0 = 1 \quad \text{and} \quad C_{n+1}=\sum_{i=0}^n C_i\,C_{n-i}\quad\text{for }n\ge 0.$

则有

。 $C_n = \frac{1}{n+1}{2n\choose n}$

下面列举几个从wiki上看到的适合catalan数的问题：

1、 C _n is the number of Dyck words of length 2 n. A Dyck word is a string consisting of n X's and n Y's such that no initial segment of the string has more Y's than X's (see also Dyck language). For example, the following are the Dyck words of length 6:

XXXYYY XYXXYY XYXYXY XXYYXY XXYXYY.

2、Re-interpreting the symbol X as an open parenthesis and Y as a close parenthesis, C_n counts the number of expressions containing n pairs of parentheses which are correctly matched:

((())) ()(()) ()()() (())() (()())

3、 C _n is the number of different ways n + 1 factors can be completely parenthesized (or the number of ways of associating n applications of a binary operator). For n = 3, for example, we have the following five different parenthesizations of four factors:

$((ab)c)d \quad (a(bc))d \quad(ab)(cd) \quad a((bc)d) \quad a(b(cd))$

4、Successive applications of a binary operator can be represented in terms of a full binary tree. (A rooted binary tree is full if every vertex has either two children or no children.) It follows that C _n is the number of full binary trees with n + 1 leaves:

5、 C_n is the number of non-isomorphic ordered trees with n+1 vertices. (An ordered tree is a rooted tree in which the children of each vertex are given a fixed left-to-right order.）

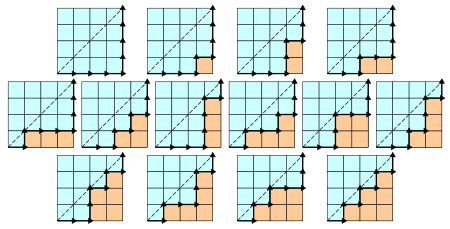
6、 C _n is the number of monotonic paths along the edges of a grid with n × n square cells, which do not pass above the diagonal. A monotonic path is one which starts in the lower left corner, finishes in the upper right corner, and consists entirely of edges pointing rightwards or upwards. Counting such paths is equivalent to counting Dyck words: X stands for "move right" and Y stands for "move up".

The following diagrams show the case n = 4:

A succinct representation is listing the Catalan elements by column height:

[0,0,0,0][0,0,0,1][0,0,0,2][0,0,1,1]

[0,1,1,1] [0,0,1,2] [0,0,0,3] [0,1,1,2][0,0,2,2][0,0,1,3]

[0,0,2,3][0,1,1,3] [0,1,2,2][0,1,2,3]

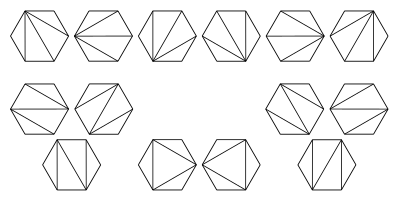
7、C_n is the number of different ways a convex polygon with n + 2 sides can be cut into triangles by connecting vertices with straight lines (a form of Polygon triangulation). The following hexagons illustrate the case n = 4: