二叉树还原

已经前序遍历  与 中序遍历 的 结果，还原出二叉树：

    private static String[] pre = {"G", "D", "A", "F", "E", "M", "H", "Z"};
    private static String[] in = {"A", "D", "E", "F", "G", "H", "M", "Z"};
    private static String[] post = {"A", "E", "F", "D", "H", "Z", "M", "G"};

public static Node getRootPreIn(String[] pre, String[] in) {
        if (pre == null || in == null) {
            return null;
        }
        Node root = new Node(pre[0]);
        if (root == null) {
            return null;
        }
        int len = pre.length;
        if (len == 0) {
            return null;
        }
        if (len == 1) {
            root.left = null;
            root.right = null;
            return root;
        }
        int index = getIndex(in, root.valStr);
        if (index <= 0) {
            //中序遍历结果，根节点是第一个，则说明没有左子树
            root.left = null;
        } else {
            String[] preChild = new String[index];
            String[] inChild = new String[index];
            System.arraycopy(pre, 1, preChild, 0, index);
            System.arraycopy(in, 0, inChild, 0, index);
            root.left = getRootPreIn(preChild, inChild);
        }

        if ((len - (index + 1)) <= 0) {
            //中序遍历结果，根节点是最后一个，则说明没有右子树
            root.right = null;
        } else {
            //index+1 ---> 左子树与根节点加一起的长度
            //len-(index+1) ---> 右子树的长度
            String[] preChild = new String[len - (index + 1)];
            String[] inChild = new String[len - (index + 1)];
            System.arraycopy(pre, index + 1, preChild, 0, len - (index + 1));
            System.arraycopy(in, index + 1, inChild, 0, len - (index + 1));
            root.right = getRootPreIn(preChild, inChild);
        }
        return root;
    }

    public static int getIndex(String[] array, String element) {
        if (array == null || TextUtils.isEmpty(element)) {
            return -1;
        }
        for (int i = 0; i < array.length; i++) {
            if (element.equalsIgnoreCase(array[i])) {
                return i;
            }
        }
        return -1;
    }

    public static class Node {
        int valInt = -1;
        String valStr;
        Node left;
        Node right;

        public Node(int self) {
            this.valInt = self;
        }

        public Node() {
        }

        public Node(String self) {
            this.valStr = self;
        }

        public String getValStr() {
            return valStr;
        }

        public void setValStr(String valStr) {
            this.valStr = valStr;
        }

        public Node getRight() {
            return right;
        }

        public void setRight(Node right) {
            this.right = right;
        }

        public int getValInt() {
            return valInt;
        }

        public void setValInt(int valInt) {
            this.valInt = valInt;
        }

        public Node getLeft() {
            return left;
        }

        public void setLeft(Node left) {
            this.left = left;
        }
    }

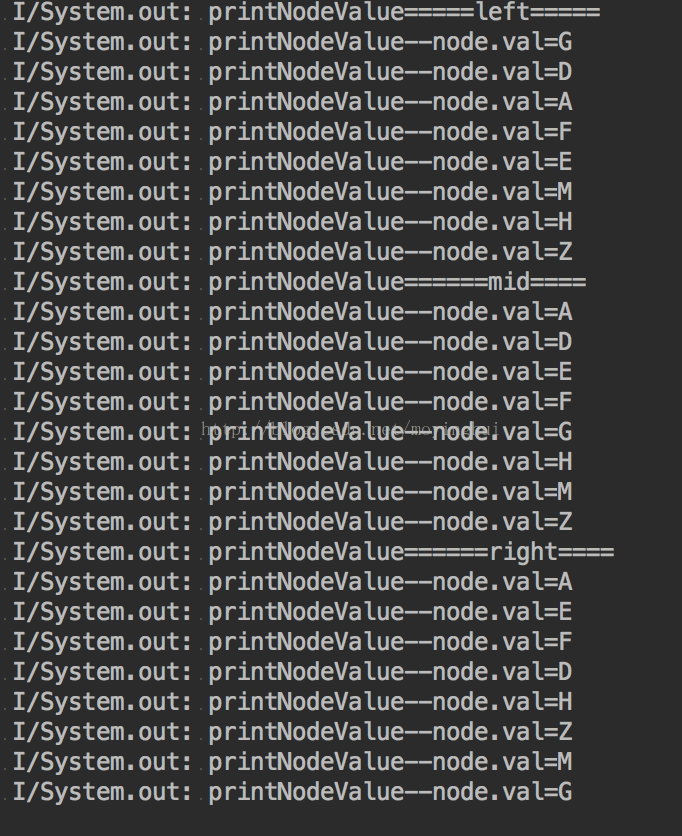
    public static void print(Node root) {
        System.out.println("printNodeValue=====pre=====");
        leftTraversal(root);
        System.out.println("printNodeValue======in====");
        midTraversal(root);
        System.out.println("printNodeValue======post====");
        rightTraversal(root);
    }

    public static Node getRoot() {
        Node root= getRootPreIn(pre, in);
        //return getRoot(pre, in);
        print(root);
        return  root;
    }

1.前序遍历的第一个节点一定是二叉树根节点；

2.中序遍历的结果中，根节点左侧的数据一定是 根节点左子树相关数据，右侧一定是根节点右子树相关数据；

    private static String[] pre = {"G", "D", "A", "F", "E", "M", "H", "Z"};
    private static String[] in = {"A", "D", "E", "F", "G", "H", "M", "Z"};

所以可以看出来，ADEF 是左子树上的数据，HMZ 是右子树上的数据；

G节点左孩子应该是D，以D为根节点的 前序遍历应该是 DAFE，中序遍历应该是 ADEF；

G节点右孩子应该是M，以M为根节点的 前序遍历赢还是 MHZ，中序遍历应该是HMZ；

然后递归。。。。。。