hdu 4568 Hunter (旅行商问题)

Hunter

Time Limit: 2000/1000 MS (Java/Others)    Memory Limit: 32768/32768 K (Java/Others)

Total Submission(s): 692    Accepted Submission(s): 190

Problem Description

　　One day, a hunter named James went to a mysterious area to find the treasures. James wanted to research the area and brought all treasures that he could.
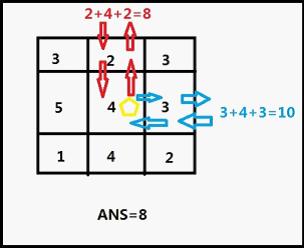
　　The area can be represented as a N*M rectangle. Any points of the rectangle is a number means the cost of research it,-1 means James can't cross it, James can start at any place out of the rectangle, and explore point next by next. He will move in the rectangle and bring out all treasures he can take. Of course, he will end at any border to go out of rectangle(James will research every point at anytime he cross because he can't remember whether the point are researched or not).
　　Now give you a map of the area, you must calculate the least cost that James bring out all treasures he can take(one point up to only one treasure).Also, if nothing James can get, please output 0.

 

Input

　　The input consists of T test cases. The number of test cases T is given in the first line of the input. Each test case begins with a line containing 2 integers N M , (1<=N,M<=200), that represents the rectangle. Each of the following N lines contains M numbers(0~9),represent the cost of each point. Next is K(1<=K<=13),and next K lines, each line contains 2 integers x y means the position of the treasures, x means row and start from 0, y means column start from 0 too.

 

Output

　　For each test case, you should output only a number means the minimum cost.

 

Sample Input

  
  

   
   2
3 3
3 2 3
5 4 3
1 4 2
1
1 1
3 3
3 2 3
5 4 3
1 4 2
2
1 1
2 2
  
  

 

Sample Output

  
  

   
   8
11
  
  

 

Source

2013 ACM-ICPC长沙赛区全国邀请赛——题目重现

思路：

蛮裸的旅行商问题，将珠宝抽象出来，bfs预处理珠宝间的距离，然后状压dp求答案就够了。

代码：

#include <iostream>
#include <cstdio>
#include <cstring>
#include <algorithm>
#include <cmath>
#include <string>
#include <map>
#include <stack>
#include <vector>
#include <set>
#include <queue>
//#pragma comment (linker,"/STACK:102400000,102400000")
#define maxn 205
#define MAXN 100005
#define mod 1000000000
#define INF 0x3f3f3f3f
using namespace std;

int n,m,ans,k,cnt,est;
int dp[1<<14][14];
int u[14],v[14];
int id[maxn][maxn],dist[14][14];
bool vis[maxn][maxn];
int mp[maxn][maxn];
int dx[]={-1,1,0,0};
int dy[]={0,0,-1,1};
struct Node
{
    int x,y,step;
    bool operator <(const Node&x1) const
    {
        return step>x1.step;
    }
}cur,now;
priority_queue<Node>q;

void bfs(int bx,int by,int xx)
{
    int i,j,nx,ny,tx,ty,flag=0;
    memset(vis,0,sizeof(vis));
    while(!q.empty()) q.pop();
    cur.x=bx;
    cur.y=by;
    cur.step=0;
    vis[bx][by]=1;
    q.push(cur);
    while(!q.empty())
    {
        now=q.top();
        q.pop();
        nx=now.x;
        ny=now.y;
        if(id[nx][ny]!=-1) dist[xx][id[nx][ny]]=now.step;
        for(i=0;i<4;i++)
        {
            tx=nx+dx[i];
            ty=ny+dy[i];
            if(tx<1||tx>n||ty<1||ty>m)
            {
                if(!flag) flag=1,dp[1<<xx][xx]=now.step+mp[u[xx]][v[xx]];
                continue ;
            }
            if(vis[tx][ty]||mp[tx][ty]==-1) continue ;
            cur.x=tx;
            cur.y=ty;
            cur.step=now.step+mp[tx][ty];
            vis[tx][ty]=1;
            q.push(cur);
        }
    }
}
void solve()
{
    int i,j,p,ma,st;
    ma=1<<k;
    for(i=0;i<ma;i++)
    {
        for(j=0;j<k;j++)
        {
            if(dp[i][j]>=INF) continue ;
            for(p=0;p<k;p++)
            {
                if(p==j||(i&(1<<p))) continue ;
                st=i|(1<<p);
                dp[st][p]=min(dp[st][p],dp[i][j]+dist[j][p]);
            }
        }
    }
    ans=INF;
    for(i=0;i<k;i++)
    {
        ans=min(ans,dp[est][i]+dp[1<<i][i]-mp[u[i]][v[i]]);
    }
    if(ans>=INF) ans=0;
}
int main()
{
    int i,j,t;
    scanf("%d",&t);
    while(t--)
    {
        scanf("%d%d",&n,&m);
        for(i=1;i<=n;i++)
        {
            for(j=1;j<=m;j++)
            {
                scanf("%d",&mp[i][j]);
            }
        }
        memset(id,-1,sizeof(id));
        memset(dp,0x3f,sizeof(dp));
        scanf("%d",&k);
        for(i=0;i<k;i++)
        {
            scanf("%d%d",&u[i],&v[i]);
            u[i]++,v[i]++;
            id[u[i]][v[i]]=i;
        }
        est=(1<<k)-1;
        for(i=0;i<k;i++)
        {
            if(mp[u[i]][v[i]]==-1)  // 汗 去掉也能A
            {
                est=est&(~(1<<i));
                continue ;
            }
            bfs(u[i],v[i],i);
            if(dp[1<<i][i]==INF) est=est&(~(1<<i));  // 汗 去掉也能A  没有设track
        }
        solve();
        printf("%d\n",ans);
    }
    return 0;
}