透视变换(Perspective Transformation)是将图片投影到一个新的视平面(Viewing Plane),也称作投影映射(Projective Mapping)。通用的变换公式为:
u,v是原始图片左边,对应得到变换后的图片坐标x,y,其中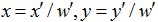
变换矩阵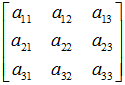
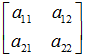
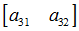
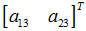
重写之前的变换公式可以得到:
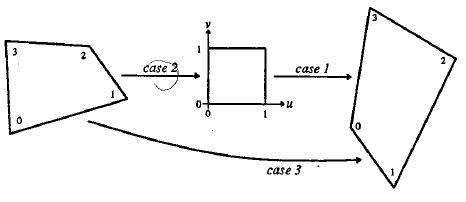
所以,已知变换对应的几个点就可以求取变换公式。反之,特定的变换公式也能新的变换后的图片。简单的看一个正方形到四边形的变换:
变换的4组对应点可以表示成:
根据变换公式得到:
定义几个辅助变量:
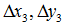
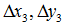
求解出的变换矩阵就可以将一个正方形变换到四边形。反之,四边形变换到正方形也是一样的。于是,我们通过两次变换:四边形变换到正方形+正方形变换到四边形就可以将任意一个四边形变换到另一个四边形。
看一段代码:
- PerspectiveTransform::PerspectiveTransform(float inA11, float inA21,
- float inA31, float inA12,
- float inA22, float inA32,
- float inA13, float inA23,
- float inA33) :
- a11(inA11), a12(inA12), a13(inA13), a21(inA21), a22(inA22), a23(inA23),
- a31(inA31), a32(inA32), a33(inA33) {}
- PerspectiveTransform PerspectiveTransform::quadrilateralToQuadrilateral(float x0, float y0, float x1, float y1,
- float x2, float y2, float x3, float y3, float x0p, float y0p, float x1p, float y1p, float x2p, float y2p,
- float x3p, float y3p) {
- PerspectiveTransform qToS = PerspectiveTransform::quadrilateralToSquare(x0, y0, x1, y1, x2, y2, x3, y3);
- PerspectiveTransform sToQ =
- PerspectiveTransform::squareToQuadrilateral(x0p, y0p, x1p, y1p, x2p, y2p, x3p, y3p);
- return sToQ.times(qToS);
- }
- PerspectiveTransform PerspectiveTransform::squareToQuadrilateral(float x0, float y0, float x1, float y1, float x2,
- float y2, float x3, float y3) {
- float dx3 = x0 - x1 + x2 - x3;
- float dy3 = y0 - y1 + y2 - y3;
- if (dx3 == 0.0f && dy3 == 0.0f) {
- PerspectiveTransform result(PerspectiveTransform(x1 - x0, x2 - x1, x0, y1 - y0, y2 - y1, y0, 0.0f,
- 0.0f, 1.0f));
- return result;
- } else {
- float dx1 = x1 - x2;
- float dx2 = x3 - x2;
- float dy1 = y1 - y2;
- float dy2 = y3 - y2;
- float denominator = dx1 * dy2 - dx2 * dy1;
- float a13 = (dx3 * dy2 - dx2 * dy3) / denominator;
- float a23 = (dx1 * dy3 - dx3 * dy1) / denominator;
- PerspectiveTransform result(PerspectiveTransform(x1 - x0 + a13 * x1, x3 - x0 + a23 * x3, x0, y1 - y0
- + a13 * y1, y3 - y0 + a23 * y3, y0, a13, a23, 1.0f));
- return result;
- }
- }
- PerspectiveTransform PerspectiveTransform::quadrilateralToSquare(float x0, float y0, float x1, float y1, float x2,
- float y2, float x3, float y3) {
- // Here, the adjoint serves as the inverse:
- return squareToQuadrilateral(x0, y0, x1, y1, x2, y2, x3, y3).buildAdjoint();
- }
- PerspectiveTransform PerspectiveTransform::buildAdjoint() {
- // Adjoint is the transpose of the cofactor matrix:
- PerspectiveTransform result(PerspectiveTransform(a22 * a33 - a23 * a32, a23 * a31 - a21 * a33, a21 * a32
- - a22 * a31, a13 * a32 - a12 * a33, a11 * a33 - a13 * a31, a12 * a31 - a11 * a32, a12 * a23 - a13 * a22,
- a13 * a21 - a11 * a23, a11 * a22 - a12 * a21));
- return result;
- }
- PerspectiveTransform PerspectiveTransform::times(PerspectiveTransform other) {
- PerspectiveTransform result(PerspectiveTransform(a11 * other.a11 + a21 * other.a12 + a31 * other.a13,
- a11 * other.a21 + a21 * other.a22 + a31 * other.a23, a11 * other.a31 + a21 * other.a32 + a31
- * other.a33, a12 * other.a11 + a22 * other.a12 + a32 * other.a13, a12 * other.a21 + a22
- * other.a22 + a32 * other.a23, a12 * other.a31 + a22 * other.a32 + a32 * other.a33, a13
- * other.a11 + a23 * other.a12 + a33 * other.a13, a13 * other.a21 + a23 * other.a22 + a33
- * other.a23, a13 * other.a31 + a23 * other.a32 + a33 * other.a33));
- return result;
- }
- void PerspectiveTransform::transformPoints(vector<float> &points) {
- int max = points.size();
- for (int i = 0; i < max; i += 2) {
- float x = points[i];
- float y = points[i + 1];
- float denominator = a13 * x + a23 * y + a33;
- points[i] = (a11 * x + a21 * y + a31) / denominator;
- points[i + 1] = (a12 * x + a22 * y + a32) / denominator;
- }
- }
- int main(){
- Mat img=imread("boy.png");
- int img_height = img.rows;
- int img_width = img.cols;
- Mat img_trans = Mat::zeros(img_height,img_width,CV_8UC3);
- PerspectiveTransform tansform = PerspectiveTransform::quadrilateralToQuadrilateral(
- 0,0,
- img_width-1,0,
- 0,img_height-1,
- img_width-1,img_height-1,
- 150,250, // top left
- 771,0, // top right
- 0,1023,// bottom left
- 650,1023
- );
- vector<float> ponits;
- for(int i=0;i<img_height;i++){
- for(int j=0;j<img_width;j++){
- ponits.push_back(j);
- ponits.push_back(i);
- }
- }
- tansform.transformPoints(ponits);
- for(int i=0;i<img_height;i++){
- uchar* t= img_trans.ptr<uchar>(i);
- for (int j=0;j<img_width;j++){
- int tmp = i*img_width+j;
- int x = ponits[tmp*2];
- int y = ponits[tmp*2+1];
- if(x<0||x>(img_width-1)||y<0||y>(img_height-1))
- continue;
- uchar* p = img.ptr<uchar>(y);
- t[j*3] = p[x*3];
- t[j*3+1] = p[x*3+1];
- t[j*3+2] = p[x*3+2];
- }
- }
- imwrite("trans.png",img_trans);
- return 0;
- }

另外在OpenCV中也实现了基础的透视变换操作,有关函数使用请见下一篇:
【OpenCV】透视变换 Perspective Transformation(续)











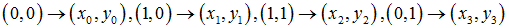
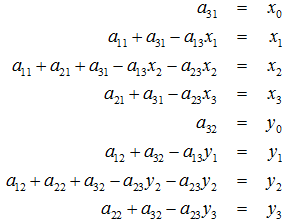
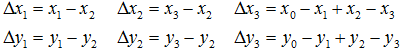
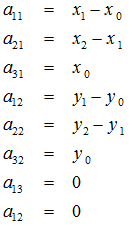
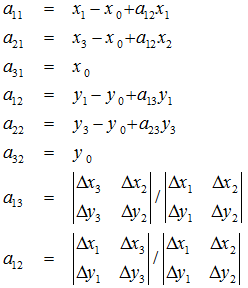














 12万+
12万+

 被折叠的 条评论
为什么被折叠?
被折叠的 条评论
为什么被折叠?








