回文子序列_计算回文子序列的总数

回文子序列

Problem statement:

问题陈述：

Given a string str, find total number of possible palindromic sub-sequences. A sub-sequence does not need to be consecutive, but for any x_ix_j i<j must be valid in the parent string too. Like "icl" is a subsequence of "includehelp" while "ple" is not.

给定字符串str ，找到可能的回文子序列的总数。 子序列不必是连续的，但是对于任何x _i x _j i <j在父字符串中也必须有效。 像“ icl”一样，是“ includehelp”的子序列，而“ ple”则不是。

Input:

输入：

The first line of input contains an integer T, denoting the no of test cases then T test cases follow. Each test case contains a string str.

输入的第一行包含一个整数T ，表示测试用例的数量，然后是T个测试用例。 每个测试用例都包含一个字符串str 。

Output:

输出：

For each test case output will be an integer denoting the total count of palindromic subsequence which could be formed from the string str.

对于每个测试用例，输出将是一个整数，表示回文子序列的总数，该总数可以由字符串str形成。

Constraints:

限制条件：

1 <= T <= 100
1 <= length of string str <= 300

Example:

例：

Input:
Test case: 2

First test case:
Input string:
"aaaa"

Output:
Total count of palindromic subsequences is: 15

Second test case:
Input string:
"abaaba"

Output:
Total count of palindromic subsequences is: 31

Explanation:

说明：

Test case 1:

测试用例1：

Input: "aaaa"

输入：“ aaaa”

The valid palindromic subsequences are shown below,

有效回文子序列如下所示，

Marked cells are character taken in subsequence:

标记的单元格是子序列中的字符：

Count=1

计数= 1

Count=2

计数= 2

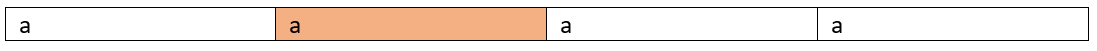
Count=3

计数= 3

Count=4

计数= 4

Count=5

计数= 5

Count=6

计数= 6

Count=7

计数= 7

Count=8

计数= 8

Count=9

计数= 9

Count=10

数= 10

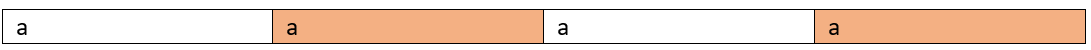
Count=11

数= 11

So on...
Total 15 palindromic sub-sequences
Actually in this case since all the character is same each and every subsequence is palindrome here.
For the second test case
Few sub-sequences can be
"a"
"b"
"a"
"aba"
So on
Total 31 such palindromic subsequences

等等...
总共15个回文子序列
实际上，在这种情况下，由于所有字符都是相同的，每个子序列在这里都是回文。
对于第二个测试用例
很少有子序列可以是
“一个”
“ b”
“一个”
“阿巴”
依此类推
总共31个这样的回文序列

Solution approach

解决方法

This can be solved by using DP bottom up approach,

这可以通过使用DP自下而上的方法来解决，

1. Initialize dp[n][n] where n be the string length to 0

   初始化dp [n] [n] ，其中n为0的字符串长度

2. Fill up the base case, Base case is that each single character is a palindrome itself. And for length of two, i.e, if adjacent characters are found to be equal then dp[i][i+1]=3, else if characters are different then dp[i][i+1]=2

   填满基本情况，基本情况是每个单个字符本身都是回文。 对于两个长度，即，如果发现相邻字符相等，则dp [i] [i + 1] = 3 ；否则，如果字符不同，则dp [i] [i + 1] = 2

   To understand this lets think of a string like "acaa"

   要理解这一点，可以考虑一个字符串，例如“ acaa”

   Here

   这里

   dp[0][1]=2 because there's only two palindrome possible because of "a" and "c".

   dp [0] [1] = 2是因为“ a”和“ c”仅可能存在两个回文。

   Whereas for

   鉴于

   dp[2][3] value will be 3 as possible subsequences are "a", "a", "aa".

   dp [2] [3]的值将为3，因为可能的子序列为“ a”，“ a”，“ aa”。

   for i=0 to n
       // for single length characters
       dp[i][i]=1; 
       if(i==n-1)
           break;        
       if(s[i]==s[i+1])
           dp[i][i+1]=3;
       else
           dp[i][i+1]=2;
   end for
   
   

3. Compute for higher lengths,

   计算更长的长度，

   for len=3 to n
       for start=0 to n-len
           int end=start+len-1;
           // start and end is matching
           if(s[end]==s[start])
               // 1+subsequence from semaining part
               dp[start][end]=1+dp[start+1][end]+dp[start][end-1];
           else
               dp[start][end]=dp[start+1][end]+dp[start][end-1]-dp[start+1][end-1];
           end if
       end for
   end for
   
   

4. Final result is stored in dp[0][n-1];

   最终结果存储在dp [0] [n-1]中；

So for higher lengths if starting and ending index is the same then we recur for the remaining characters, since we have the sub-problem result stored so we computed that. In case start and end index character are different then we have added dp[start+1][end] and dp[start][end-1] that's similar to recur for leaving starting index and recur for leaving end index. But it would compute dp[start+1][end-1] twice and that why we have deducted that.

因此，对于更大的长度，如果开始索引和结束索引相同，那么我们将重复其余字符，因为我们存储了子问题结果，因此我们对其进行了计算。 如果起始索引和终止索引的字符不同，则我们添加了dp [start + 1] [end]和dp [start] [end-1] ，类似于recur离开起始索引和recur离开结束索引。 但是它将两次计算dp [start + 1] [end-1] ，这就是为什么我们要减去它。

For proper understanding you can compute the table by hand for the string "aaaa" to understand how it's working.

为了正确理解，您可以手动计算字符串“ aaaa”的表以了解其工作方式。

C++ Implementation:

C ++实现：

#include <bits/stdc++.h>
using namespace std;

int countPS(string s)
{

    int n = s.length();
    int dp[n][n];
    
    memset(dp, 0, sizeof(dp));

    for (int i = 0; i < n; i++) {
        dp[i][i] = 1;
        if (i == n - 1)
            break;

        if (s[i] == s[i + 1])
            dp[i][i + 1] = 3;
        else
            dp[i][i + 1] = 2;
    }

    for (int len = 3; len <= n; len++) {
        for (int start = 0; start <= n - len; start++) {
            int end = start + len - 1;
            if (s[end] == s[start]) {
                dp[start][end] = 1 + dp[start + 1][end] + dp[start][end - 1];
            }
            else {
                dp[start][end] = dp[start + 1][end] + dp[start][end - 1] - dp[start + 1][end - 1];
            }
        }
    }

    return dp[0][n - 1];
}

int main()
{
    int t;
    cout << "Enter number of testcases\n";
    cin >> t;
    
    while (t--) {
        string str;
        cout << "Enter the input string\n";
        cin >> str;
    
        cout << "Total Number of palindromic Subsequences are: " << countPS(str) << endl;
    }
    
    return 0;
}

Output:

输出：

Enter number of testcases
2
Enter the input string
aaaa
Total Number of palindromic Subsequences are: 15
Enter the input string
abaaba
Total Number of palindromic Subsequences are: 31

翻译自: https://www.includehelp.com/icp/count-total-number-of-palindromic-subsequences.aspx

回文子序列