大顶堆

1. 概述: 最大堆(大顶堆)

一句话 heap：一种数据结构，完全二叉树（若二叉树高 h，则除过最底层 h 层，其他 1~h-1 层都是满的；并且最底层从左到右不能有空隙）。有最大堆和最小堆两种，最大堆即根节点的键值比其他所有节点键值都大；最小堆即根节点的键值比其他所有节点键值都小。在实现上，它没有选择一般的二叉树数据结构（即一个节点包含指向两个孩子的指针），使用的是数组；heap 最为常用的操作是上溯和下溯，在“维持堆”和“堆排序”中经常用到。

最小堆和最大堆思路如出一辙，只讨论最大堆。对于一个大顶堆，如下结论成立:

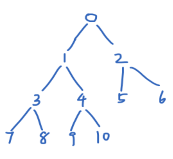
1. 数组从 index为 1 的地方开始存放第一个节点：

• 第 i 个节点的父节点的 index 为 ( i/2 )
• 第 i 个节点的 left 孩子节点的 index 为 ( 2* i )
• 第 i 个节点的 right 孩子节点的 index 为 ( 2* i + 1 )

这里根节点就规定是[1]，那么最后一个 非叶子 节点就是[last/2]

1. 当然，从 index为 0 的地方开始存放第一个节点，稍微复杂一点，我们下面的代码就是使用了这个。

• 第 i 个节点的父节点的 index 为 ( i-1 ) / 2
• 第 i 个节点的 left 孩子节点的 index 为 2* i + 1
• 第 i 个节点的 right 孩子节点的 index 为 2* i + 2

根节点就是[0]，那么最后一个 非叶子 节点就是[ ( last - 1 ) / 2 ] (也就是最后一个节点的父亲)

2. 实现

maxheap.h

#ifndef __MAX_HEAP
#define __MAX_HEAP

void shiftDown(int *a, int pos, int last);
void shiftDown2(int *a, int pos, int last);
void ShiftUp(int *a, int pos);

//============================================================
void maxHeapBuild(int *a, int size);
void maxHeapSort(int *a, int size);

//============================================================
#define PRIOR_QUEUE_BASE_SIZE        50
#define PRIOR_QUEUE_MAX_SIZE        100
#define PRIOR_QUEUE_INCREASE_SIZE    10
typedef struct
{
    int *a;
    int totalSize;
    int validSize;    //validSize 其实也是第一个空闲的位置
    //mutex SHOULD be used
}PriorQueue;

void createPriorQueue(PriorQueue **ppPriorQueue);
void destroyPriorQueue(PriorQueue *pPriorQueue);
void enPriorQueue(PriorQueue *pPriorQueue,int prior);
int dePriorQueue(PriorQueue *pPriorQueue);
void showQueue(PriorQueue *pPriorQueue);

#endif

maxheap.c

#include <stdio.h>
#include <string.h>
#include <stdlib.h>
#include "maxHeap.h"

//一般在操作堆顶后,用此做 shiftDown 调整
//eg.1 删除堆顶: 交换堆顶和最后元素(其实根本不用交换,直接用last覆盖堆顶),then size--
//eg.2 新添一个节点在pos位置(或者更改堆顶点),如果这个pos节点
//     比较小(和左右子节点比),那么此节点会一直 沉到它应该呆的位置
void shiftDown(int *a, int pos, int last)    //last 是最后一个节点的 index
{
    int left = 2*pos+1;        //pos 的左孩子的 index
    int right = 2*pos+2;    //pos 的右孩子的 index
    int max = pos;
    int tmp;

    if(pos <= (last-1)/2)    //叶子节点没必要调整
    {
        if(left <= last && a[left] > a[max])
            max = left;

        if(right <= last && a[right] > a[max])
            max = right;    //now,max已经是父子三人中最大的那个元素的 index 了

        if(max != pos)        //max 是某个子节点的 index
        {
            //swap(a[pos], a[max]);
            tmp = a[pos];
            a[pos] = a[max];
            a[max] = tmp;
            shiftDown(a, max, last);
        }
    }
}

//heapShiftDown 之非递归实现(迭代实现)
void shiftDown2(int *a, int pos, int last)
{
    int left;        //pos 的左孩子的 index
    int right;        //pos 的右孩子的 index
    int max = pos;        //先假设 pos 最大
    int tmp;
    int flag = 0;    //为1表示已经满足最大堆约束

    while(pos <= (last-1)/2 && (flag==0))    //叶子节点没必要调整
    {
        left = 2*pos+1;        //pos 的左孩子的 index
        right = 2*pos+2;    //pos 的右孩子的 index
        //max = pos;            //先假设 pos 最大

        if(left <= last && a[left] > a[max])
            max = left;

        if(right <= last && a[right] > a[max])
            max = right;    //now,max已经是父子三人中最大的那个元素的 index 了

        if(max != pos)        //max 是某个子节点的 index
        {
            //swap(a[pos], a[max]);
            tmp = a[pos];
            a[pos] = a[max];
            a[max] = tmp;
            //
            pos = max;
        }
        else
        {
            flag = 1;
        }
    }
}

//当改动了heap的最后一个元素的时候需要调用此 ShiftUp 调整一下
void ShiftUp(int *a, int pos)
{
    int flag = 0;
    int tmp;

    while((pos > 0) && (flag==0))    //如果已经是堆顶了(pos == 0),就不要再调整了
    {
        if(a[pos] > a[(pos-1)/2])
        {
            //swap(a[pos], a[(pos-1)/2]);
            tmp = a[pos];
            a[pos] = a[(pos-1)/2];
            a[(pos-1)/2] = tmp;
            //
        }
        else
        {
            flag = 1;
        }
        pos = (pos-1)/2;
    }
}

//============================= 堆排序 ================================================

//将一个数组搞成一个最大堆(并不严格排序!),a[0]不管他了???
void maxHeapBuild(int *a, int size)
{
    int i;
    //last=size-1,last的parent就是 (last-1)/2 = size/2 -1
    for(i = size/2 - 1; i>=0; i--)    //从所有有孩子的节点往上遍历(相当于挨个插入节点了)
    {
        shiftDown2(a,i,size-1);
    }
}

//将一个数组搞成最大堆,然后利用最大堆属性
//慢慢将数组搞成严格排序
void maxHeapSort(int *a, int size)
{
    int i;
    int tmp;
    maxHeapBuild(a, size);
    for(i = size-1; i>=0; i--)
    {
        //swap(a[i], a[1]);    //交换堆顶 a[0] 和最后一个元素(即 将最大值放在了最后)
        tmp = a[i];
        a[i] = a[0];
        a[0] = tmp;
        //最大值放在最后,同时最后的那个值也放在了堆顶,肯定需要重新调整为 maxHeap,
        //(最后的那个元素已经不把他当作堆中一员了)
        shiftDown(a,0,i-1);
    }
}



//============================= 优先级队列 ==============================================
void createPriorQueue(PriorQueue **ppPriorQueue)
{
    PriorQueue *p = NULL;
    if(!ppPriorQueue)
    {
        printf("createPriorQueue:invalid param..\n");
        return;
    }
    p = (PriorQueue *)malloc(sizeof(PriorQueue));
    if(!p)
    {
        printf("createPriorQueue:no avail mem for PriorQueue struct..\n");
        return;
    }
    p->a = (int *)malloc(PRIOR_QUEUE_BASE_SIZE*sizeof(int));    //sizeof(int)忘掉写了,会导致free失败?
    if(!(p->a))
    {
        printf("createPriorQueue:no avail mem for PriorQueue array..\n");
        return;
    }
    p->totalSize = PRIOR_QUEUE_BASE_SIZE;
    p->validSize = 0;
    *ppPriorQueue = p;
}

void destroyPriorQueue(PriorQueue *pPriorQueue)
{
    if(!pPriorQueue)
    {
        printf("destroyPriorQueue:invalid param..\n");
        return;
    }
    if(pPriorQueue->a)
        free(pPriorQueue->a);
    free(pPriorQueue);
}

void enPriorQueue(PriorQueue *pPriorQueue,int prior)
{
    int *pTmp = NULL;
    if(!pPriorQueue)
    {
        printf("enPriorQueue:invalid param..\n");
        return;
    }
    if(pPriorQueue->validSize >= pPriorQueue->totalSize)
    {
        if(pPriorQueue->totalSize + PRIOR_QUEUE_INCREASE_SIZE > PRIOR_QUEUE_MAX_SIZE)
        {
            printf("enPriorQueue:exceed max size, will not alloc mem for heap any more\n");
            return;
        }
        pTmp = pPriorQueue->a;
        pPriorQueue->a = (int *)malloc((pPriorQueue->totalSize+PRIOR_QUEUE_INCREASE_SIZE)*sizeof(int));
        if(!pPriorQueue->a)
        {
            printf("enPriorQueue:no avail mem for PriorQueue array..\n");
            return;
        }
        memcpy(pPriorQueue->a,pTmp,pPriorQueue->totalSize);
        pPriorQueue->totalSize += PRIOR_QUEUE_INCREASE_SIZE;
        printf("enPriorQueue:increase heap size success..\n");
        free(pTmp);
        pTmp = NULL;
    }
    pPriorQueue->a[pPriorQueue->validSize] = prior;
    ShiftUp(pPriorQueue->a, pPriorQueue->validSize);
    pPriorQueue->validSize++;
}

int dePriorQueue(PriorQueue *pPriorQueue)
{
    int prior;
    if(!pPriorQueue)
    {
        printf("dePriorQueue:invalid param..\n");
        return -1;
    }
    if(pPriorQueue->validSize == 0)
    {
        printf("dePriorQueue:prior queue is empty..\n");
        return -1;
    }
    prior = pPriorQueue->a[0];
    pPriorQueue->validSize--;
    pPriorQueue->a[0] = pPriorQueue->a[pPriorQueue->validSize];    //直接用最后一个覆盖[0]
    shiftDown2(pPriorQueue->a, 0, pPriorQueue->validSize-1);    //原来的那个最后一个已经不算再内了
    return prior;
}

//for debug
void showQueue(PriorQueue *pPriorQueue)
{
    int i;
    if(!pPriorQueue)
        return;
    for(i=0;i<pPriorQueue->validSize;i++)
    {
        printf("%d ,",pPriorQueue->a[i]);
    }
    printf("\n");
}

test.c

#include <stdio.h>
#include <stdlib.h>
#include <string.h>
#include "maxHeap.h"

#define MAX_HEAP_NUM 10000

int maxHeap_test()
{
    int prior;
    PriorQueue *pPQ = NULL;
    int i = 0;
    int *abc = malloc(MAX_HEAP_NUM*sizeof(int));
    srand(0); //生成随机种子

    printf("\n====================随机生成 %d 个数==============================\n",MAX_HEAP_NUM);
    for(i=0;i<MAX_HEAP_NUM;i++)
    {
        abc[i] = rand()%(10*MAX_HEAP_NUM);
        printf("%d ",abc[i]);
    }
    printf("\n");
    printf("\n==================== 优先级队列 ==============================\n");
    
    createPriorQueue(&pPQ);

    printf("PQ");
    for(i=0;i<MAX_HEAP_NUM/10;i++)
    {
        printf("<== %d ",abc[i]);
        enPriorQueue(pPQ,abc[i]);
    }
    printf("\n");

    for(i=0;i<25;i++)
    {
        prior = dePriorQueue(pPQ);
        printf("==> %d \n",prior);
        //showQueue(pPQ);
    }

    destroyPriorQueue(pPQ);
    pPQ = NULL;

    printf("\n==================== 堆排序 ==============================\n");
    maxHeapSort(abc, MAX_HEAP_NUM);
    for(i=0;i<MAX_HEAP_NUM;i++)
    {
        printf("%d ",abc[i]);
    }
    printf("\n");
    
    return 0;
}

3. 后话

• 堆排序 O(N*logN)：

• 求一个数组中（N个元素）中最小的K个数：

1. 快速排序，然后取前K个数 O(N*logN)+Q(K)
2. 维护一个含有K个数的最大堆（刚开始即为初始的K个数），然后后面（N-K）个数依次与heap[1]（大顶）比较，小于它则入围（直接覆盖heap[1]），更新大顶堆。否则不更新 N*O(logK) (只要K比N小，就比上面的有优势，最差也是和上面一样)
3. 快排 Partition 找到第k小的数，再遍历一遍 O()

• 利用最大堆实现优先级队列（数组方式实现的哦）：

1. 入队就是插入元素到堆。a[size++] = new，then shiftUp()
2. 出队当然出优先级最高的了，即将堆顶出队。a[0] = a[size-1]，then shiftDown()
   这种方式实现的是一个不稳定的优先级队列，即当两个相同值（这个值就被认为是 prior）被插入时，shift后并不保证他们之间的相对顺序不改变。

网上有个人说了一个实现稳定优先级队列的方法：
最大堆实现的优先级队列只保存不同的prior，同时prior还作为key被存入一个hashmap，冲突时采用链接法将节点链接起来。当插入节点时，之前没有相同的prior才入队。当冲突链上所有节点都被删除时，才出队