代码随想录算法训练营Day20 | 235. 二叉搜索树的最近公共祖先、701.二叉搜索树中的插入操作、450.删除二叉搜索树中的节点

目录

235. 二叉搜索树的最近公共祖先

701.二叉搜索树中的插入操作

450.删除二叉搜索树中的节点

235. 二叉搜索树的最近公共祖先

题目

235. 二叉搜索树的最近公共祖先 - 力扣（LeetCode）

给定一个二叉搜索树, 找到该树中两个指定节点的最近公共祖先。

百度百科中最近公共祖先的定义为：“对于有根树 T 的两个结点 p、q，最近公共祖先表示为一个结点 x，满足 x 是 p、q 的祖先且 x 的深度尽可能大（一个节点也可以是它自己的祖先）。”

例如，给定如下二叉搜索树: root = [6,2,8,0,4,7,9,null,null,3,5]

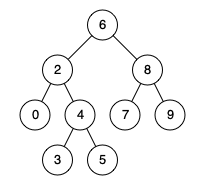
示例1：

输入: root = [6,2,8,0,4,7,9,null,null,3,5], p = 2, q = 8
输出: 6 
解释: 节点 2 和节点 8 的最近公共祖先是 6。

示例2：

输入: root = [6,2,8,0,4,7,9,null,null,3,5], p = 2, q = 4
输出: 2
解释: 节点 2 和节点 4 的最近公共祖先是 2, 因为根据定义最近公共祖先节点可以为节点本身。

说明:

• 所有节点的值都是唯一的。
• p、q 为不同节点且均存在于给定的二叉搜索树中。

思路

代码随想录：235.二叉搜索树的最近公共祖先

视频讲解：235. 二叉搜索树的最近公共祖先

类似于236. 二叉树的最近公共祖先 - 力扣（LeetCode），区别是本题为二叉搜索树，可以利用其特性进行解题。

从根节点向下遍历，有三种情况：

1. 当前节点的值小于q.val和p.val，说明目标节点在当前节点的右子树中。
2. 当前节点的值大于q.val和p.val，说明目标节点在当前节点的左子树中。
3. 当前节点的值在q.val和p.val之间，当前节点即为最近公共祖先。

本题使用递归法和迭代法都比较简单。

题解

递归法：

class Solution {
    public TreeNode lowestCommonAncestor(TreeNode root, TreeNode p, TreeNode q) {
        if (root.val < p.val && root.val < q.val)
            return lowestCommonAncestor(root.right, p, q);
        if (root.val > p.val && root.val > q.val)
            return lowestCommonAncestor(root.left, p, q);
        return root;
    }
}

迭代法：

class Solution {
    public TreeNode lowestCommonAncestor(TreeNode root, TreeNode p, TreeNode q) {
        TreeNode cur = root;
        while (cur != null) {
            if (cur.val > p.val && cur.val > q.val) {
                cur = cur.left;
            } else if (cur.val < p.val && cur.val < q.val) {
                cur = cur.right;
            } else {
                return cur;
            }
        }
        return cur;
    }
}

701.二叉搜索树中的插入操作

题目

701. 二叉搜索树中的插入操作 - 力扣（LeetCode）

给定二叉搜索树（BST）的根节点 root 和要插入树中的值 value ，将值插入二叉搜索树。 返回插入后二叉搜索树的根节点。 输入数据 保证 ，新值和原始二叉搜索树中的任意节点值都不同。

注意，可能存在多种有效的插入方式，只要树在插入后仍保持为二叉搜索树即可。 你可以返回 任意有效的结果 。

示例1：

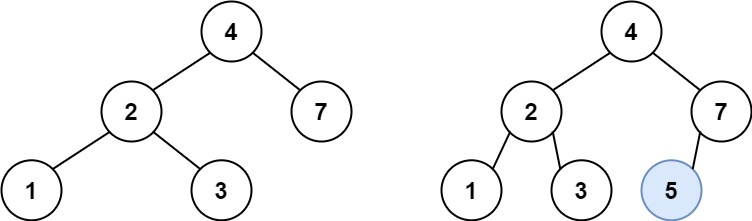
输入：root = [4,2,7,1,3], val = 5
输出：[4,2,7,1,3,5]

解释：另一个满足题目要求可以通过的树是：

示例2：

输入：root = [40,20,60,10,30,50,70], val = 25
输出：[40,20,60,10,30,50,70,null,null,25]

示例3：

输入：root = [4,2,7,1,3,null,null,null,null,null,null], val = 5
输出：[4,2,7,1,3,5]

提示：

• 树中的节点数将在 [0, 104]的范围内。
• -108 <= Node.val <= 108
• 所有值 Node.val 是 独一无二 的。
• -108 <= val <= 108
• 保证 val 在原始BST中不存在。

思路

代码随想录：701.二叉搜索树中的插入操作

视频讲解：701.二叉搜索树中的插入操作

根据二叉搜索树的特性向下遍历直到叶子节点即可。

题解

递归法：

class Solution {
    public TreeNode insertIntoBST(TreeNode root, int val) {
        TreeNode node = new TreeNode();
        if (root == null) {
            node.val = val;
            return node;
        }
        if (root.val < val) {
            node = insertIntoBST(root.right, val);
            if (root.right == null)
                root.right = node;
        } else if (root.val > val) {
            node = insertIntoBST(root.left, val);
            if (root.left == null)
                root.left = node;
        }
        return root;
    }
}

优化：

class Solution {
    public TreeNode insertIntoBST(TreeNode root, int val) {
        if (root == null) {
            TreeNode node = new TreeNode(val);
            return node;
        }
        if (root.val < val) {
            root.right = insertIntoBST(root.right, val);
        } else if (root.val > val) {
            root.left = insertIntoBST(root.left, val);
        }
        return root;
    }
}

迭代法：

class Solution {
    public TreeNode insertIntoBST(TreeNode root, int val) {
        if (root == null)
            return new TreeNode(val);
        TreeNode cur = root;
        TreeNode pre = root;
        while (cur != null) {
            pre = cur;
            if (cur.val < val) {
                cur = cur.right;
            } else if (cur.val > val) {
                cur = cur.left;
            }
        }
        if (pre.val < val) {
            pre.right = new TreeNode(val);
        } else {
            pre.left = new TreeNode(val);
        }
        return root;
    }
}

450.删除二叉搜索树中的节点

题目

450. 删除二叉搜索树中的节点 - 力扣（LeetCode）

给定一个二叉搜索树的根节点 root 和一个值 key，删除二叉搜索树中的 key 对应的节点，并保证二叉搜索树的性质不变。返回二叉搜索树（有可能被更新）的根节点的引用。

一般来说，删除节点可分为两个步骤：

1. 首先找到需要删除的节点；
2. 如果找到了，删除它。

示例1：

输入：root = [5,3,6,2,4,null,7], key = 3
输出：[5,4,6,2,null,null,7]

解释：给定需要删除的节点值是 3，所以我们首先找到 3 这个节点，然后删除它。
一个正确的答案是 [5,4,6,2,null,null,7], 如下图所示。
另一个正确答案是 [5,2,6,null,4,null,7]。

示例2：

输入: root = [5,3,6,2,4,null,7], key = 0
输出: [5,3,6,2,4,null,7]
解释: 二叉树不包含值为 0 的节点

示例3：

输入: root = [], key = 0
输出: []

提示:

• 节点数的范围 [0, 104].
• -105 <= Node.val <= 105
• 节点值唯一
• root 是合法的二叉搜索树
• -105 <= key <= 105

思路

代码随想录：450.删除二叉搜索树中的节点

视频讲解：LeetCode：450.删除二叉搜索树中的节点

删除节点有四种情况：

1. 没有找到目标节点
2. 目标节点为叶子节点，直接删除，返回null
3. 目标节点的左右孩子有一个为空，删除后返回不为空的孩子为根节点
4. 目标节点的左右孩子都不为空，删除后将左孩子放到右子树里面最左侧的叶子节点之下，返回右孩子为根节点

情况四如下图：

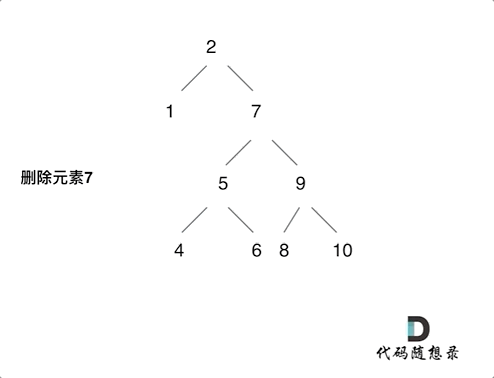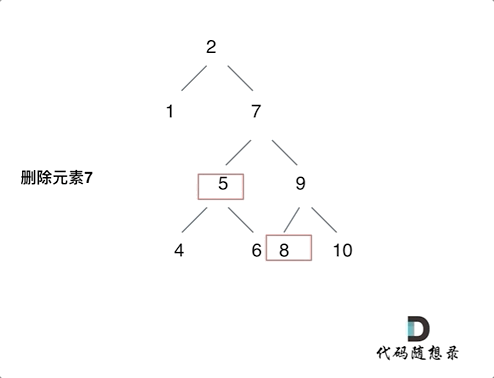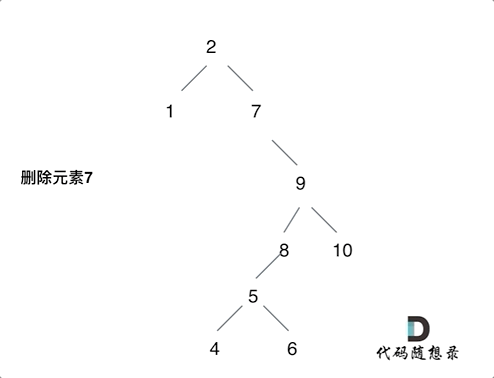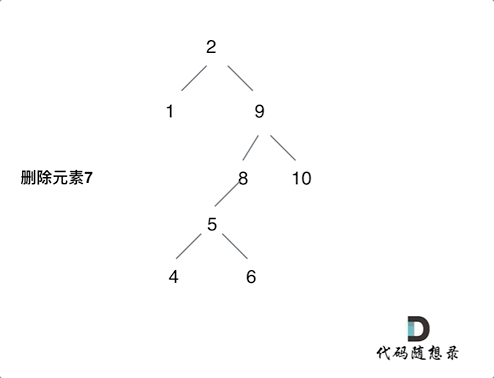
题解

递归法：

class Solution {
    public TreeNode deleteNode(TreeNode root, int key) {
        if (root == null)
            return null;
        if (root.val == key) {
            if (root.left == null && root.right == null)
                return null;
            if (root.left == null && root.right != null)
                return root.right;
            if (root.left != null && root.right == null)
                return root.left;
            if (root.left != null && root.right != null) {
                TreeNode cur = root.right;
                while (cur.left != null) {
                    cur = cur.left;
                }
                cur.left = root.left;
                return root.right;
            }
        }
        if (root.val < key) {
            root.right = deleteNode(root.right, key);
        } else if (root.val > key) {
            root.left = deleteNode(root.left, key);
        }
        return root;
    }
}