BZOJ传送门 Codevs传送门
2301: [HAOI2011]Problem b
Time Limit: 50 Sec Memory Limit: 256 MB
Submit: 2723 Solved: 1200
[Submit][Status][Discuss]
Description
对于给出的n个询问,每次求有多少个数对(x,y),满足a≤x≤b,c≤y≤d,且gcd(x,y) = k,gcd(x,y)函数为x和y的最大公约数。
Input
第一行一个整数n,接下来n行每行五个整数,分别表示a、b、c、d、k
Output
共n行,每行一个整数表示满足要求的数对(x,y)的个数
Sample Input
2
2 5 1 5 1
1 5 1 5 2
Sample Output
14
3
HINT
100%的数据满足:1≤n≤50000,1≤a≤b≤50000,1≤c≤d≤50000,1≤k≤50000
Source
最近也懒得写题解了,基本上数论全部懵逼,题基本照着打直接给跪了
自己写的式子,不好见谅哈,如有不对请指出!
#include<cstdio>
#include<cstring>
#include<cstdlib>
#include<algorithm>
#include<iostream>
using namespace std;
#define N 50005
#define MAXN 1024*512
bool v[N];int mu[N],p[N];long long sum[N];
int cnt,n,t,a,b,c,d,k;long long ans;
void GetPrime(){
mu[1]=1;
for(int i=2;i<N;i++){
if(!v[i]){mu[i]=-1;p[cnt++]=i;}
for(int j=0;j<cnt&&i*p[j]<N;j++){
v[i*p[j]]=true;
if(i%p[j]) mu[i*p[j]]=-mu[i];
else{mu[i*p[j]]=0;break;}
}
}
for(int i=1;i<N;i++) sum[i]=sum[i-1]+mu[i];
}
long long getans(int n,int m){
long long res=0;if(n>m) swap(n,m);
for(int i=1,j;i<=n;i=j+1){
j=min(n/(n/i),m/(m/i));
res+=(sum[j]-sum[i-1])*(n/i)*(m/i);
}
return res;
}
char buf[MAXN],*ps=buf,*pe=ps+1;
inline void rnext(){//读优
if(++ps==pe)
pe=(ps=buf)+fread(buf,1,sizeof(buf),stdin);
}
inline int in(){
do{rnext();}while(!isdigit(*ps));
int ans=0;
do{ans=(ans<<1)+(ans<<3)+*ps-48;rnext();
}while(isdigit(*ps));
return ans;
}
/*
int in(){
int x=0;char ch=getchar();
while(ch>'9'||ch<'0') ch=getchar();
while(ch>='0'&&ch<='9') x=x*10+ch-'0',ch=getchar();
return x;
}*/
int main(){
GetPrime();
t=in();
while(t--){
a=in(),b=in(),c=in(),d=in(),k=in();
ans=getans(b/k,d/k);ans-=getans((a-1)/k,d/k);
ans-=getans(b/k,(c-1)/k);ans+=getans((a-1)/k,(c-1)/k);
printf("%lld\n",ans);
}
}










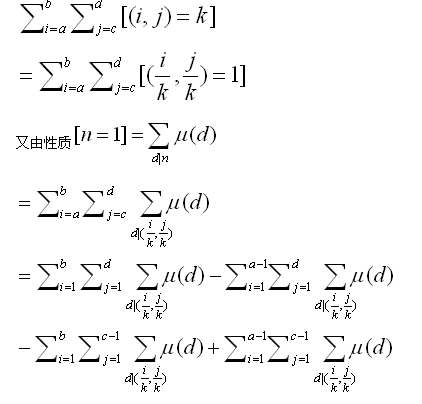














 396
396

 被折叠的 条评论
为什么被折叠?
被折叠的 条评论
为什么被折叠?








