HDU-1506

最新推荐文章于 2019-05-23 22:42:30 发布

Dragon--C

最新推荐文章于 2019-05-23 22:42:30 发布

阅读量436

点赞数

分类专栏：随笔

本文链接：https://blog.csdn.net/u013769121/article/details/46129375

版权

随笔专栏收录该内容

6 篇文章 0 订阅

订阅专栏

Largest Rectangle in a Histogram

Time Limit: 2000/1000 MS (Java/Others) Memory Limit: 65536/32768 K (Java/Others)
Total Submission(s): 13452 Accepted Submission(s): 3804

Problem Description

A histogram is a polygon composed of a sequence of rectangles aligned at a common base line. The rectangles have equal widths but may have different heights. For example, the figure on the left shows the histogram that consists of rectangles with the heights 2, 1, 4, 5, 1, 3, 3, measured in units where 1 is the width of the rectangles:

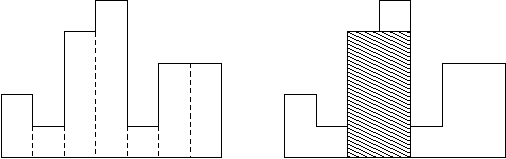
Usually, histograms are used to represent discrete distributions, e.g., the frequencies of characters in texts. Note that the order of the rectangles, i.e., their heights, is important. Calculate the area of the largest rectangle in a histogram that is aligned at the common base line, too. The figure on the right shows the largest aligned rectangle for the depicted histogram.

Input

The input contains several test cases. Each test case describes a histogram and starts with an integer n, denoting the number of rectangles it is composed of. You may assume that 1 <= n <= 100000. Then follow n integers h1, ..., hn, where 0 <= hi <= 1000000000. These numbers denote the heights of the rectangles of the histogram in left-to-right order. The width of each rectangle is 1. A zero follows the input for the last test case.

Output

For each test case output on a single line the area of the largest rectangle in the specified histogram. Remember that this rectangle must be aligned at the common base line.

Sample Input

  
  
   
   7 2 1 4 5 1 3 3
4 1000 1000 1000 1000
0

Sample Output

Source

University of Ulm Local Contest 2003

我在想这题为什么和dp有关啊? 暂时还不知道

我认为就是一道思维题吧

对于每个小长方形的高度我们需要求出以它为高的左右边界左边的比它本身高的话，就一直递归找下去。右边也是这样。

#include <stdio.h>
#include <iostream>
#include <algorithm>
#include <cstring>

using namespace std;
#define ll long long
#define Maxn 100010


int main()
{
	//freopen("in.in","r",stdin);
	int n;
	while(scanf("%d",&n)!=EOF&&n)
	{
        int l[Maxn],r[Maxn];
        ll a[Maxn];
        ll maxn,area;
		for(int i=1;i<=n;i++)
		{
			cin>>a[i];
			r[i]=l[i]=i;
		}
		a[0]=a[n+1]=-1;
		for(int i=1;i<=n;i++)
		{
			while(a[l[i]-1]>=a[i])
			{
				l[i]=l[l[i]-1];
			}
		}

		for(int i=n;i>=1;i--)
		{
			while(a[r[i]+1]>=a[i])
			{
				r[i]=r[r[i]+1];
			}
		}

		maxn=0;
		for(int i=1;i<=n;i++)
		{
			area=(r[i]-l[i]+1)*a[i];
			maxn= area>maxn?area:maxn;
		}
		printf("%lld\n",maxn);
	}
	return 0;
}