Kadj Squares - POJ 3347 几何

Kadj Squares

Time Limit: 2000MS		Memory Limit: 65536K
Total Submissions: 2801		Accepted: 1090

Description

In this problem, you are given a sequence S₁, S₂, ..., S_n of squares of different sizes. The sides of the squares are integer numbers. We locate the squares on the positive x-y quarter of the plane, such that their sides make 45 degrees with x and y axes, and one of their vertices are on y=0 line. Let b_i be the x coordinates of the bottom vertex of S_i. First, put S₁ such that its left vertex lies on x=0. Then, put S₁, (i > 1) at minimum b_i such that

• b_i > b_i-1 and
• the interior of S_i does not have intersection with the interior of S₁...S_i-1.

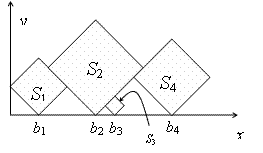
The goal is to find which squares are visible, either entirely or partially, when viewed from above. In the example above, the squares S₁, S₂, and S₄ have this property. More formally, S_i is visible from above if it contains a point p, such that no square other than S_i intersect the vertical half-line drawn from p upwards.

Input

The input consists of multiple test cases. The first line of each test case is n (1 ≤ n ≤ 50), the number of squares. The second line contains n integers between 1 to 30, where the ith number is the length of the sides of S_i. The input is terminated by a line containing a zero number.

Output

For each test case, output a single line containing the index of the visible squares in the input sequence, in ascending order, separated by blank characters.

Sample Input

4
3 5 1 4
3
2 1 2
0

Sample Output

1 2 4
1 3

题意：按照如图所示的方法放这些矩形，问有哪些矩形其正上面没有被完全覆盖。

思路：我们将矩形的边长乘上根号2，保证其端点都在整数坐标上。首先找到所有矩形最下方的那个点的位置。因为n比较小，一点点向右移动枚举其是否与前面的矩形有重叠即可。然后得到每个矩形的位置后，也可以得到其l-r的范围，按照s的大小排序，然后遍历找到这个矩形l-r是否已经完全被覆盖。

AC代码如下：

#include<cstdio>
#include<cstring>
#include<algorithm>
using namespace std;
struct Point
{
    int x,y;
    Point(int x=0,int y=0):x(x),y(y){}
};
struct node
{
    int id,l,r,h;
}line[55];
bool cmp(node a,node b)
{
    return a.h>b.h;
}
Point p[55][4];
int n,m,s[55],pos[55];
bool vis[100010];
int ans[55];
bool check(int i,int x)
{
    int j,k;
    for(j=1;j<i;j++)
       if(x-pos[j]<min(s[j],s[i])*2)
         return 0;
    return 1;
}
int main()
{
    int i,j,k;
    bool flag;
    while(~scanf("%d",&n) && n>0)
    {
        m=0;
        for(i=1;i<=n;i++)
        {
            scanf("%d",&s[i]);
            while(true)
            {
                m=max(m,s[i]);
                if(check(i,m))
                {
                    pos[i]=m;
                    m++;
                    break;
                }
                m++;
            }
        }

        for(i=1;i<=n;i++)
        {
            line[i].l=pos[i]-s[i]+1;
            line[i].r=pos[i]+s[i];
            line[i].h=s[i];
            line[i].id=i;
        }
        sort(line+1,line+1+n,cmp);
        ans[0]=0;
        memset(vis,0,sizeof(vis));
        for(i=1;i<=n;i++)
        {
            flag=0;
            for(j=line[i].l;j<=line[i].r;j++)
            {
                if(!vis[j])
                  flag=1;
                vis[j]=1;
            }
            if(flag)
              ans[++ans[0]]=line[i].id;
        }
        sort(ans+1,ans+1+ans[0]);
        printf("%d",ans[1]);
        for(i=2;i<=ans[0];i++)
           printf(" %d",ans[i]);
        printf("\n");
    }
}