codeforces 1015E1&&E2

E1. Stars Drawing (Easy Edition)

time limit per test

3 seconds

memory limit per test

256 megabytes

input

standard input

output

standard output

A star is a figure of the following type: an asterisk character '*' in the center of the figure and four rays (to the left, right, top, bottom) of the same positive length. The size of a star is the length of its rays. The size of a star must be a positive number (i.e. rays of length $\mathrm{00 are\; not\; allowed).}$

Let's consider empty cells are denoted by '.', then the following figures are stars:

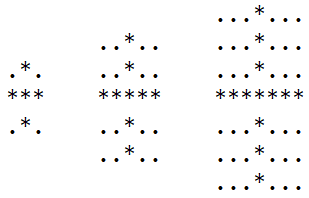The leftmost figure is a star of size $\mathrm{11,\; the\; middle\; figure\; is\; a star of\; size 22 and\; the\; rightmost\; figure\; is\; a star of\; size 33.}$

You are given a rectangular grid of size $\mathrm{n\times mn\times m consisting\; only\; of\; asterisks\; \text{'}*\text{'}\; and\; periods\; (dots)\; \text{'}.\text{'}.\; Rows\; are\; numbered\; from 11 to nn,\; columns\; are\; numbered\; from 11 to mm.\; Your\; task\; is\; to\; draw\; this\; grid\; using any number\; of stars or\; find\; out\; that\; it\; is\; impossible. Stars can\; intersect,\; overlap\; or\; even\; coincide\; with\; each\; other.\; The\; number\; of stars in\; the\; output\; can\text{'}t\; exceed n\cdot mn\cdot m.\; Each\; star\; should\; be\; completely\; inside\; the\; grid.\; You\; can\; use\; stars\; of\; same\; and\; arbitrary\; sizes.}$

In this problem, you do not need to minimize the number of stars. Just find any way to draw the given grid with at most $\mathrm{n\cdot mn\cdot m stars.}$

Input

The first line of the input contains two integers $\mathrm{nn and mm (3\le n,m\le 1003\le n,m\le 100)\; —\; the\; sizes\; of\; the\; given\; grid.}$

The next $\mathrm{nn lines\; contains mm characters\; each,\; the ii-th\; line\; describes\; the ii-th\; row\; of\; the\; grid.\; It\; is\; guaranteed\; that\; grid\; consists\; of\; characters\; \text{'}*\text{'}\; and\; \text{'}.\text{'}\; only.}$

Output

If it is impossible to draw the given grid using stars only, print "-1".

Otherwise in the first line print one integer $\mathrm{kk (0\le k\le n\cdot m0\le k\le n\cdot m)\; —\; the\; number\; of stars needed\; to\; draw\; the\; given\; grid.\; The\; next kk lines\; should\; contain\; three\; integers\; each\; — xjxj, yjyj and sjsj,\; where xjxj is\; the\; row\; index\; of\; the\; central star character, yjyj is\; the\; column\; index\; of\; the\; centralstar character\; and sjsj is\; the\; size\; of\; the star.\; Each star should\; be\; completely\; inside\; the\; grid.}$

Examples

input

Copy

6 8
....*...
...**...
..*****.
...**...
....*...
........

output

Copy

3
3 4 1
3 5 2
3 5 1

input

Copy

5 5
.*...
****.
.****
..**.
.....

output

Copy

3
2 2 1
3 3 1
3 4 1

input

Copy

5 5
.*...
***..
.*...
.*...
.....

output

Copy

-1

input

Copy

3 3
*.*
.*.
*.*

output

Copy

-1

Note

In the first example the output

2
3 4 1
3 5 2

is also correct.

 

题意：给你一个大小为n*m的图，求其中‘*’的十字架大小

题解：暴力，记得给走过的路打标记

代码如下:

#include <map>
#include <set>
#include <cmath>
#include <ctime>
#include <stack>
#include <queue>
#include <cstdio>
#include <cctype>
#include <bitset>
#include <string>
#include <vector>
#include <cstring>
#include <iostream>
#include <algorithm>
#include <functional>
#define fuck(x) cout<<"["<<x<<"]";
#define FIN freopen("input.txt","r",stdin);
#define FOUT freopen("output.txt","w+",stdout);
//#pragma comment(linker, "/STACK:102400000,102400000")
using namespace std;
typedef long long LL;
typedef pair<int, int> PII;
const int maxn = 1005;
char str[maxn][maxn];
bool vis[maxn][maxn];
int n,m;
struct node {
    int x,y,cnt;
};

std::vector<node> v;

bool check(int x,int y,int r){
    if(x+r>=n||x-r<0||y+r>=m||y-r<0) return 0;
    return 1;
}

int main(){
#ifndef ONLINE_JUDGE
    FIN
#endif

    scanf("%d%d",&n,&m);
    for(int i=0;i<n;i++){
        scanf("%s",str[i]);
    }

    for(int i=0;i<n;i++){
        for(int j=0;j<m;j++){
            int cnt=0;
            if(str[i][j]=='*'){
                for(int a=1; ;a++){
                    if(check(i,j,a)){
                        if(str[i+a][j]=='*'&&str[i-a][j]=='*'&&str[i][j+a]=='*'&&str[i][j-a]=='*'){
                            cnt++;
                            vis[i+a][j] = vis[i-a][j] = vis[i][j+a] = vis[i][j-a] = 1;  //求十字架的长度
                            vis[i][j] = 1;
                        }else break;
                    }else break;
                }
            }
            if (cnt != 0) 
                v.push_back(node{i+1, j+1, cnt});
        }
    }

    bool ok = 1;
    for (int i = 0; i < n; i++){
        for (int j = 0; j < m; j++){
            if (str[i][j] == '*' && !vis[i][j]){
                ok = 0;
                break;
            }
        }
    }

   if (!ok){
        printf("-1\n");
        
    }else{
        printf("%d\n", v.size());
        for (int i = 0; i < v.size(); i++){
            printf("%d %d %d\n", v[i].x, v[i].y, v[i].cnt);
        }
    }

}

View Code

 

转载于:https://www.cnblogs.com/buerdepepeqi/p/9416521.html