HDU1022--Train Problem I（栈的应用）

Problem Description

As the new term comes, the Ignatius Train Station is very busy nowadays. A lot of student want to get back to school by train(because the trains in the Ignatius Train Station is the fastest all over the world ^v^). But here comes a problem, there is only one railway where all the trains stop. So all the trains come in from one side and get out from the other side. For this problem, if train A gets into the railway first, and then train B gets into the railway before train A leaves, train A can\\\\\\\'t leave until train B leaves. The pictures below figure out the problem. Now the problem for you is, there are at most 9 trains in the station, all the trains has an ID(numbered from 1 to n), the trains get into the railway in an order O1, your task is to determine whether the trains can get out in an order O2.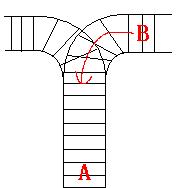

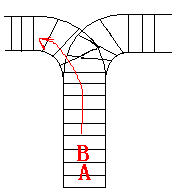
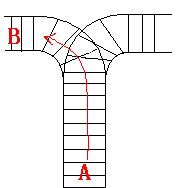
Input

The input contains several test cases. Each test case consists of an integer, the number of trains, and two strings, the order of the trains come in:O1, and the order of the trains leave:O2. The input is terminated by the end of file. More details in the Sample Input.

Output

            The output contains a string \\\\\\\"No.\\\\\\\" if you can\\\\\\\'t exchange O2 to O1, or you should output a line contains \\\\\\\"Yes.\\\\\\\", and then output your way in exchanging the order(you should output \\\\\\\"in\\\\\\\" for a train getting into the railway, and \\\\\\\"out\\\\\\\" for a train getting out of the railway). Print a line contains \\\\\\\"FINISH\\\\\\\" after each test case. More details in the Sample Output.

Sample Input

3 123 321
3 123 312

Sample Output

Yes.
in
in
in
out
out
out
FINISH
No.
FINISH

Hint

Hint For the first Sample Input, we let train 1 get in, then train 2 and train 3. So now train 3 is at the top of the railway, so train 3 can leave first, then train 2 and train 1. In the second Sample input, we should let train 3 leave first, so we have to let train 1 get in, then train 2 and train 3. Now we can let train 3 leave. But after that we can\\\\\\\'t let train 1 leave before train 2, because train 2 is at the top of the railway at the moment. So we output \\\\\\\"No.\\\\\\\".

Author

Ignatius.L

题意：

很有意思的一道题目

有一个站台C，只能FILO（先进后出），并且火车只能驶向B轨道，轨道A上的火车只能驶向C轨道

给出从A驶向C的顺序，以及从C驶向B的顺序，问这种顺序有可能吗？可能的话请输出方案

思路：

一开始想着在算法竞赛入门经典上看见过这道题目，只顾着回想思路了（尴尬的是忘记了。。。）

其实简单分析一下，题目其实就是问的在这种入栈顺序下，给出的出栈顺序有无可能，而每次出栈时，只能出栈顶元素。

设置标记f=0，出栈序列为O。模拟一下入栈顺序，当此时栈顶的元素等于等于O[f]时，出栈，f++，直至栈顶元素不等于O[f]，停止出栈

最后，若栈中还有元素或者f！=n  ，则此情况不成立

代码：

#include<bits/stdc++.h>
using namespace std;
const int MAXN=1000003;
int s[MAXN];
char a[MAXN],b[MAXN];
bool path[MAXN];
int main()
{
    int n;
    while(cin>>n){
        memset(path,-1,sizeof(path));
        scanf("%s",a);
        getchar();
        scanf("%s",b);
        int cou=-1;
        int coub=0;
        int coup=0;
        for(int i=0;i<n;i++){
            s[++cou]=a[i]-'0';
            path[coup++]=1;
            while(s[cou]==(b[coub]-'0')&&cou!=-1&&coub<n){
                //cout<<11111111<<endl;
                cou--;
                coub++;
                path[coup++]=0;
            }
        }
        if(cou!=-1)cout<<"NO."<<endl;
        else{
            cout<<"Yes."<<endl;
            for(int i=0;i<2*n;i++){
                if(path[i])cout<<"in"<<endl;
                else cout<<"out"<<endl;
            }
        }
        cout<<"FINISH"<<endl;
    }
}

转载于:https://www.cnblogs.com/liuzhanshan/p/6293938.html