Stochastic dominance 随机优势

最新推荐文章于 2023-02-03 11:56:34 发布

维博

最新推荐文章于 2023-02-03 11:56:34 发布

阅读量8.4k

点赞数 4

本文链接：https://blog.csdn.net/foreverdengwei/article/details/8220181

版权

随机优势

　　随机占优（Stochastic dominance），为风险资产选择提供了一个简单的工具（Whitmore和Findlay，1978）。我们用一个简单的例子解释随机占优关系：假设投资者想在两个风险资产X和Y之间做一个选择，如果在未来任何情况下X的收益总是超过Y的收益，只要投资者是永远不会满足的，那么投资者不会持有Y，因为持有X得到的回报一定会更好。因此，运用这种方法，不需要对投资者的效用函数、投资者需要规避的风险因子以及风险资产收益的分布做任何假设，我们就可以对风险资产进行排序。

　　上述例子仅仅是一阶随机占优（first-order stochastic dominance，FSD）的一个特例。更一般地，如果对任意x，资产Y的收益小于或等于x的概率大于资产X，那么资产X对资产Y是一阶随机占优的。只要投资者的目标是效用最大化，而且永远不会满足，那么投资者就不会选择Y。

编辑本段 主要的三种关系

　　随机占优关系主要有三种：一阶随机占优（FSD）；二阶随机占优（SSD）和三阶随机占优（TSD）。随机占优的严格定义是：假设X和Y的收益的累积概率密度函数（CDF）分别为F1和G1，X对Y是一阶随机占优的，当且仅当对任意的x有

因此如果X 的收益的概率密度函数在Y 的收益的概率密度函数的右边，那么X 对Y 是一阶随机占优的。一阶随机占优的条件很强，因此有了二阶随机占优和三阶随机占优。定义F2 和G2 分别为F1和G1 与横轴以及x=a（a 为任意实数）所围区域的面积，那么X 对Y 是二阶随机占优的，当且仅当对任意的x 有

二阶随机占优允许X 和Y 的收益的累积概率密度函数有交叉的可能。最后，定义F3 和G3 分别为F2 和G2 与横轴以及x=a（a 为任意实数）所围区域的面积，uX和uY 分别为X 和Y 的期望收益。那么X 对Y 是三阶随机占优的，当且仅当对任意的x 有

三种占优关系之间的联系是

因此，我们证明了一阶随机占优，就说明存在二阶和三阶的随机占优。随机占优的成立只需对投资者的效用函数做以下假设：一阶随机占优要求投资者的目标是效用最大化，而且永远不会满足；二阶随机占优要求投资者不但是不会满足的，而且是风险厌恶的；三阶随机占优要求投资者不但是不会满足和风险厌恶的，而且绝对风险厌恶系数是递减的。

　　如果存在随机占优，投资者持有占优资产预期效用总是更高的，因此理性投资者不会持有不占优的资产。另外，从直观意义上看，如果资产X对资产Y是一阶随机占优的，那说明无论在什么情况下，资产X的收益都不低于资产Y的收益，此时会有无风险的套利机会存在，即卖空资产Y买入资产X就可以获得无风险的收益。

一阶随机占优是指两种资产在大于某一个常数收益时，一种资产比另一种资产的收益概率高。 Stochastic dominance

Stochastic dominance^[1]^[2] is a form of stochastic ordering. The term is used in decision theory and decision analysis to refer to situations where one gamble (a probability distribution over possible outcomes, also known as prospects) can be ranked as superior to another gamble. It is based on preferences regarding outcomes. A preference might be a simple ranking of outcomes from favorite to least favored, or it might also employ a value measure (i.e., a number associated with each outcome that allows comparison of multiples of one outcome with another, such as two instances of winning a dollar vs. one instance of winning two dollars.) Only limited knowledge of preferences is required for determining dominance. Risk aversion is a factor only in second order stochastic dominance.

Stochastic dominance does not give a complete ordering: For some pairs of gambles, neither one stochastically dominates the other.

A related concept not included under stochastic dominance is deterministic dominance, which occurs when the least preferable outcome of gamble A is more valuable than the most highly preferred outcome of gamble B.

[hide]

[edit]Statewise dominance

The simplest case of stochastic dominance is statewise dominance (also known as state-by-state dominance), defined as follows: gamble A is statewise dominant over gamble B if A gives a better outcome than B in every possible future state (more precisely, at least as good an outcome in every state, with strict inequality in at least one state). For example, if a dollar is added to one or more prizes in a lottery, the new lottery statewise dominates the old one. Similarly, if a risk insurance policy has a lower premium and a better coverage than another policy, then with or without damage, the outcome is better. Anyone who prefers more to less (in the standard terminology, anyone who hasmonotonically increasing preferences) will always prefer a statewise dominant gamble.

[edit]First-order stochastic dominance

Statewise dominance is a special case of the canonical first-order stochastic dominance, defined as follows: gamble A has first-order stochastic dominance over gamble B if for any good outcome x, A gives at least as high a probability of receiving at least x as does B, and for some x, A gives a higher probability of receiving at least x. In notation form, $P [A \ge x]\ge P [B \ge x]$ for all x, and for some x, $P[A \ge x]>P[B \ge x]$ . In terms of the cumulative distribution functions of the two gambles, A dominating B means that $F_A(x) \le F_B(x)$ for all x, with strict inequality at some x. For example, consider a die-toss where 1 through 3 wins $1 and 4 through 6 wins $2 in gamble B. This is dominated by a gamble A that yields $3 for 1 through 3 and $1 for 4 through 6, and it is also dominated by a gamble C that gives $1 for 1 and 2 and $2 for 3 through 6. Gamble A would have statewise dominance over B if we re-ordered the die toss outcome by value won, but gamble C has first-order stochastic dominance over B without statewise dominance no matter how we order the prospects^{[clarification needed]} . Further, although when A dominates B, the expected value of the payoff under A will be greater than the expected value of the payoff under B, this is not a sufficient condition for dominance, and so one cannot order lotteries with regard to stochastic dominance simply by comparing the means of their probability distributions.

Every expected utility maximizer with an increasing utility function will prefer gamble A over gamble B if A first-order stochastically dominates B.

First-order stochastic dominance can also be expressed as follows: If and only if A first-order stochastically dominates B, there exists some gamble 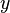$y$ such that $x_B \overset {d}{=} (x_A+y)$ where $y\le 0$ in all possible states (and strictly negative in at least one state); here $\overset{d}{=}$ means "is equal in distribution to" (that is, "has the same distribution as"). Thus, we can go from the graphed density function of A to that of B by, roughly speaking, pushing some of the probability mass to the left.

[edit]Second-order stochastic dominance

The other commonly used type of stochastic dominance is second-order stochastic dominance. Roughly speaking, for two gambles A and B, gamble A has second-order stochastic dominance over gamble B if the former is more predictable (i.e. involves less risk) and has at least as high a mean. All risk-averse expected-utility maximizers (that is, those with increasing and concave utility functions) prefer a second-order stochastically dominant gamble to a dominated gamble. The same is true for non-expected utility maximizers with utility functions that are locally concave.

In terms of cumulative distribution functions $F_A$ and $F_B$ , A is second-order stochastically dominant over B if and only if the area under $F_A$ from minus infinity to $x$ is less than or equal to that under $F_B$ from minus infinity to $x$ for all real numbers $x$ , with strict inequality at some $x$ ; that is, $\int_{-\infty}^x [F_B(t) - F_A(t)]dt \geq 0$ for all $x$ , with strict inequality at some $x$ . Equivalently, $A$ dominates $B$ in the second order if and only if $E_AU(x) \geq E_BU(x)$ for all nondecreasing and concave utility functions $U$ .

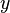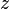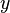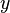Second-order stochastic dominance can also be expressed as follows: If and only if A second-order stochastically dominates B, there exist some gambles $y$ and $z$ such that $x_B \overset {d}{=} (x_A + y + z)$ , with $y$ always less than or equal to zero, and with $E(z|x_A+y)=0$ for all values of $x_A+y$ . Here the introduction of random variable $y$ makes B first-order stochastically dominated by A (making B disliked by those with an increasing utility function), and the introduction of random variable 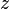$z$ introduces a mean-preserving spread in B which is disliked by those with concave utility. Note that if A and B have the same mean (so that the random variable 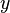$y$ degenerates to the fixed number 0), then B is a mean-preserving spread of A.

[edit]Second-order stochastic dominance in portfolio analysis

Portfolio analysis typically assumes that all investors are risk averse. Therefore, no investor would choose a portfolio that is second-order stochastically dominated by some other portfolio. See modern portfolio theory and marginal conditional stochastic dominance.

[edit]Sufficient conditions for second-order stochastic dominance

First-order stochastic dominance is a sufficient condition.

[edit]Necessary conditions for second-order stochastic dominance

$E_A(x) \geq E_B(x)$ is a necessary condition.
If $A$ dominates $B$ in the second order, then the geometric mean of $A$ must be greater than or equal to the geometric mean of $B$ .^{[clarification needed]}
$\min_A(x)\geq\min_B(x)$ is a necessary condition. The condition implies that the left tail of $F_B$ must be thicker than the left tail of $F_A$ .

[edit]Third-order stochastic dominance

Let $F_A$ and $F_B$ be the cumulative distribution functions of two distinct investments $A$ and $B$ . $A$ dominates $B$ in the third order if and only if

$\int_{-\infty}^x \int_{-\infty}^z [F_B(t) - F_A(t)] \, dt \, dz \geq 0$ for all $x$ ,

$E_A(x) \geq E_B(x), \,$

and there is at least one strict inequality. Equivalently, $A$ dominates $B$ in the third order if and only if $E_AU(x) \geq E_BU(x)$ for all nondecreasing, concave utility functions $U$ that are positively skewed (that is, have a positive third derivative throughout).

[edit]Sufficient condition for third-order stochastic dominance

Second-order stochastic dominance is a sufficient condition.

[edit]Necessary conditions for third-order stochastic dominance

$E_A(\log(x))\geq E_B(\log(x))$ is a necessary condition. The condition implies that the geometric mean of $A$ must be greater than or equal to the geometric mean of $B$ .
$\min_A(x)\geq\min_B(x)$ is a necessary condition. The condition implies that the left tail of $F_B$ must be thicker than the left tail of $F_A$ .

[edit]Higher-order stochastic dominance

Higher orders of stochastic dominance have also been analyzed, as have generalizations of the dual relationship between stochastic dominance orderings and classes of preference functions.

[edit]References

^ Hadar and Russell,"Rules for Ordering Uncertain Prospects", American Economic Review 59, March 1969, 25-34.
^ Bawa, Vijay S., "Optimal Rules for Ordering Uncertain Prospects," Journal of Financial Economics 2, 1975, 95-121.