第十二周项目2-操作用邻接表存储的图

最新推荐文章于 2017-11-16 10:42:38 发布

ly201458506115

最新推荐文章于 2017-11-16 10:42:38 发布

阅读量326

点赞数

本文链接：https://blog.csdn.net/ly201458506115/article/details/49997011

版权

问题：

/*
* Copyright (c)2015,烟台大学计算机与控制工程学院
* All rights reserved.
* 文件名称：项目2.cbp
* 作    者：李艺
* 完成日期：2015年11月23日
* 版 本 号：v1.0


* 问题描述：假设图G采用邻接表存储，分别设计实现以下要求的算法：


* 输入描述：无
* 程序输出：测试数据
*/

（1）输出出图G中每个顶点的出度

代码：

#include "graph.h"

//返回图G中编号为v的顶点的出度
int OutDegree(ALGraph *G,int v)
{
    ArcNode *p;
    int n=0;
    p=G->adjlist[v].firstarc;
    while (p!=NULL)
    {
        n++;
        p=p->nextarc;
    }
    return n;
}
//输出图G中每个顶点的出度
void OutDs(ALGraph *G)
{
    int i;
    for (i=0; i<G->n; i++)
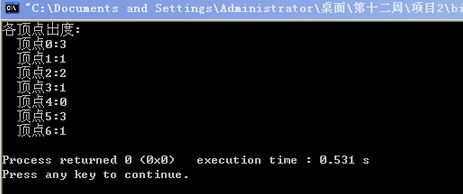
        printf("  顶点%d:%d\n",i,OutDegree(G,i));
}
int main()
{
    ALGraph *G;
    int A[7][7]=
    {
        {0,1,1,1,0,0,0},
        {0,0,0,0,1,0,0},
        {0,0,0,0,1,1,0},
        {0,0,0,0,0,0,1},
        {0,0,0,0,0,0,0},
        {0,0,0,1,1,0,1},
        {0,1,0,0,0,0,0}
    };
    ArrayToList(A[0], 7, G);
    printf("各顶点出度:\n");
    OutDs(G);
    return 0;
}

运行结果：

（２）求出图G中出度最大的一个顶点，输出该顶点编号

代码：

#include "graph.h"

//返回图G中编号为v的顶点的出度
int OutDegree(ALGraph *G,int v)
{
    ArcNode *p;
    int n=0;
    p=G->adjlist[v].firstarc;
    while (p!=NULL)
    {
        n++;
        p=p->nextarc;
    }
    return n;
}

//输出图G中每个顶点的出度
void OutDs(ALGraph *G)
{
    int i;
    for (i=0; i<G->n; i++)
        printf("  顶点%d:%d\n",i,OutDegree(G,i));
}

//输出图G中出度最大的一个顶点
void OutMaxDs(ALGraph *G)
{
    int maxv=0,maxds=0,i,x;
    for (i=0; i<G->n; i++)
    {
        x=OutDegree(G,i);
        if (x>maxds)
        {
            maxds=x;
            maxv=i;
        }
    }
    printf("顶点%d，出度=%d\n",maxv,maxds);
}

int main()
{
    ALGraph *G;
    int A[7][7]=
    {
        {0,1,1,1,0,0,0},
        {0,0,0,0,1,0,0},
        {0,0,0,0,1,1,0},
        {0,0,0,0,0,0,1},
        {0,0,0,0,0,0,0},
        {0,0,0,1,1,0,1},
        {0,1,0,0,0,0,0}
    };
    ArrayToList(A[0], 7, G);

    printf("最大出度的顶点信息:");
    OutMaxDs(G);

    return 0;
}

运行结果：

（3）计算图G中出度为0的顶点数；

代码：

#include "graph.h"

//返回图G中编号为v的顶点的出度
int OutDegree(ALGraph *G,int v)
{
    ArcNode *p;
    int n=0;
    p=G->adjlist[v].firstarc;
    while (p!=NULL)
    {
        n++;
        p=p->nextarc;
    }
    return n;
}

//输出图G中每个顶点的出度
void OutDs(ALGraph *G)
{
    int i;
    for (i=0; i<G->n; i++)
        printf("  顶点%d:%d\n",i,OutDegree(G,i));
}

//输出图G中出度为0的顶点数
void ZeroDs(ALGraph *G)
{
    int i,x;
    for (i=0; i<G->n; i++)
    {
        x=OutDegree(G,i);
        if (x==0)
            printf("%2d",i);
    }
    printf("\n");
}

int main()
{
    ALGraph *G;
    int A[7][7]=
    {
        {0,1,1,1,0,0,0},
        {0,0,0,0,1,0,0},
        {0,0,0,0,1,1,0},
        {0,0,0,0,0,0,1},
        {0,0,0,0,0,0,0},
        {0,0,0,1,1,0,1},
        {0,1,0,0,0,0,0}
    };
    ArrayToList(A[0], 7, G);

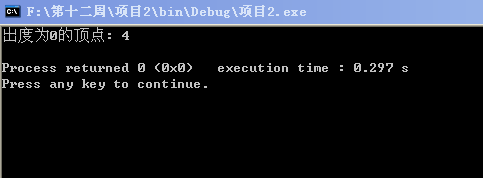
    printf("出度为0的顶点:");
    ZeroDs(G);

    return 0;
}

运行结果：

（4）判断图G中是否存在边 <i,j>

 
 
  
  #include "graph.h"

//返回图G中编号为v的顶点的出度
int OutDegree(ALGraph *G,int v)
{
    ArcNode *p;
    int n=0;
    p=G->adjlist[v].firstarc;
    while (p!=NULL)
    {
        n++;
        p=p->nextarc;
    }
    return n;
}

//输出图G中每个顶点的出度
void OutDs(ALGraph *G)
{
    int i;
    for (i=0; i<G->n; i++)
        printf("  顶点%d:%d\n",i,OutDegree(G,i));
}

//输出图G中出度最大的一个顶点
void OutMaxDs(ALGraph *G)
{
    int maxv=0,maxds=0,i,x;
    for (i=0; i<G->n; i++)
    {
        x=OutDegree(G,i);
        if (x>maxds)
        {
            maxds=x;
            maxv=i;
        }
    }
    printf("顶点%d，出度=%d\n",maxv,maxds);
}
//输出图G中出度为0的顶点数
void ZeroDs(ALGraph *G)
{
    int i,x;
    for (i=0; i<G->n; i++)
    {
        x=OutDegree(G,i);
        if (x==0)
            printf("%2d",i);
    }
    printf("\n");
}

//返回图G中是否存在边<i,j>
bool Arc(ALGraph *G, int i,int j)
{
    ArcNode *p;
    bool found = false;
    p=G->adjlist[i].firstarc;
    while (p!=NULL)
    {
        if(p->adjvex==j)
        {
            found = true;
            break;
        }
        p=p->nextarc;
    }
    return found;
}

int main()
{
    ALGraph *G;
    int A[7][7]=
    {
        {0,1,1,1,0,0,0},
        {0,0,0,0,1,0,0},
        {0,0,0,0,1,1,0},
        {0,0,0,0,0,0,1},
        {0,0,0,0,0,0,0},
        {0,0,0,1,1,0,1},
        {0,1,0,0,0,0,0}
    };
    ArrayToList(A[0], 7, G);

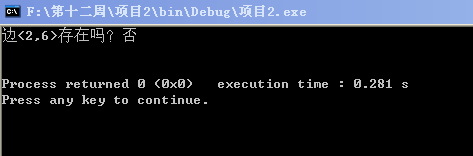
    printf("边<2,6>存在吗？");
    if(Arc(G,2,6))
        printf("是\n");
    else
        printf("否\n");
    printf("\n");
    
    return 0;
}

运行结果：