Polygon zkEVM 基本概念

1. 引言

前序博客有：

• ZK-Rollups工作原理
• Polygon zkEVM——Hermez 2.0简介
• Polygon zkEVM网络节点

Polygon zkEVM为zk-rollup layer 2扩容方案，其：

• execute smart contracts transparently
• publish zero-knowledge validity proof
• 与以太坊虚拟机opcode完全兼容——为此需重建所有EVM opcodes，从而支持transparent deployment of any existing Ethereum smart contract。

开源代码见：

• https://github.com/orgs/0xPolygonHermez

各代码库有：

Core Repos	Specific Tools & Libraries	Generic Tools & Libraries
zkevm-proverjs：以Javascript编写的zkEVM proof generator。	zkevm-commonjs：以Javascript实现的Polygon-Hermez zkEVM通用工具库。	pilcom：为Polynomial Identity Language（PIL）编译器。
zkevm-rom：包含了polygon-Hermez zkEVM的zkASM源码。	zkasmcom：zkASM Compiler。将zkASM文件编译为json文件，供zkExecutor使用。	pil-stark：Generate a stark from a SM written in pil。
zkevm-prover：以C++语言实现的zkEVM proof generator。	zkevm-testvectors：为与Ethereum test类似的，针对Polygon-Hermez zkEVM实现所提供的test vectors。
zkevm-node：Go语言实现的Polygon zkEVM节点软件。	zkevm-storagerom：为Storage状态机的zkASM实现，可将.zkasm storage编译为json文件。
zkevm-contracts：相关智能合约。	goldilocks：为一个素数域，用于实现Poseidon哈希函数。
zkevm-bridge-service：与bridge合约交互，提供Merkleproofs。Go语言。	zkevm-comms-protocol：模仿真实的zk-Prover接口和功能。
zkevm-bridge-ui：UI界面，用户用于在以太坊和Polygon zkEVM之间来回转移ETH和ERC-20资产。
zkevm-doc：相关文档。

2. EVM Arithmetization

证明某EVM交易执行正确的第一步是：

• 构建一个合适的execution trace。

所谓execution trace，是指满足EVM processing约束的一组值。将execution trace以矩阵表示，每列具有一个name。将每列插值为一个多项式，将execution的正确性 最终reduce为 验证多项式之间（列之间） 的一组identities。设计合适的列和identities的过程 称为 arithmetization。

Polygon zkEVM提供了an efficient arithmetization of the EVM。

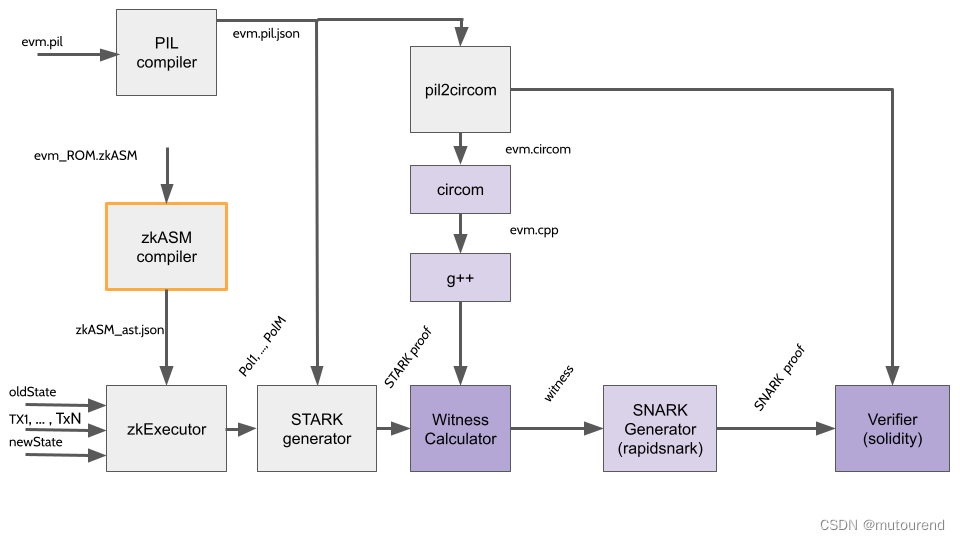
3. Executor, zkASM 和 zkProver

由Executor负责创建execution trace。
Executor的输入有：

• 1）a batch of 交易
• 2）a chain ID
• 3）代表该链zkEVM previous state的Merkle tree root
• 4）代表这些交易执行之后的该链zkEVM new state的Merkle tree root
• 5）values of the current state of the zkEVM，以构建proof

Executor本质上是一种名为zkASM的汇编语言解释器。
使用zkASM语言来构建名为zkROM的程序，Executor运行zkROM程序来提供合适的execution trace。

在zkROM程序中，每个EVM opcode都以一组zkASM指令集来实现。
每个zkASM指令利用了execution trace矩阵中的一行，又名zkEVM的一个“step”。

Executor为zkProver的一部分，zkProver为Polygon zkEVM的核心部件。

zkProver与节点和Database（DB）之间的交互流程为：

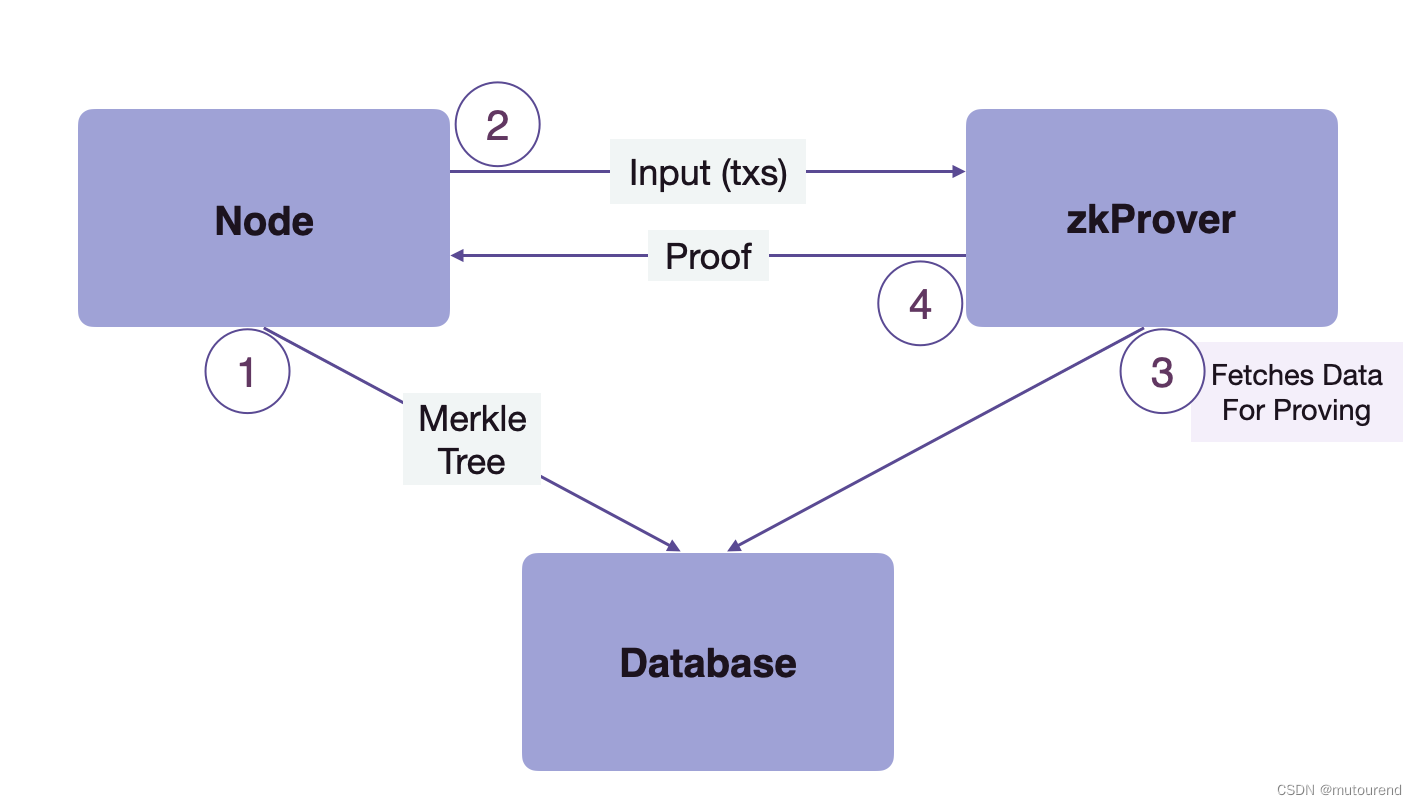
• 1）节点将Ethereum state content和EVM Merkle trees发送到DB并存储。
• 2）节点将input batch of transactions发送到zkProver。
• 3）zkProver访问DB，获取 为节点发送的transaction batch 生成verifiable proof 所需的信息。
• 4）zkProver为交易生成证明，将proof发回给节点。

3.1 zkProver中的状态机

根据zkEVM Audit Education Sessions 1/4 -Circuit Arithmetization for ZKP有：

zkProver采用模块化设计，主要由14种state machines组成：

• 1）1个Main State Machine
• 2）6个Secondary State Machine：Binary SM、Storage SM、Memory SM、Arithmetic SM、Keccak Function SM、Poseidon SM。
• 3）7个Auxiliary State Machine：Padding-PG SM、Padding-KK SM、Nine2One SM、Memory Align SM、Norm Gate SM、Byte4 SM、ROM SM。

zkProver这种模块化的设计，使得Main SM可将其职责委托给尽可能专业的state machine，通过委托实现效率的提升。

3.1.1 Secondary State Machine

Main SM Executor直接向secondary state machine发送名为Actions的合适指令 来传达命令。

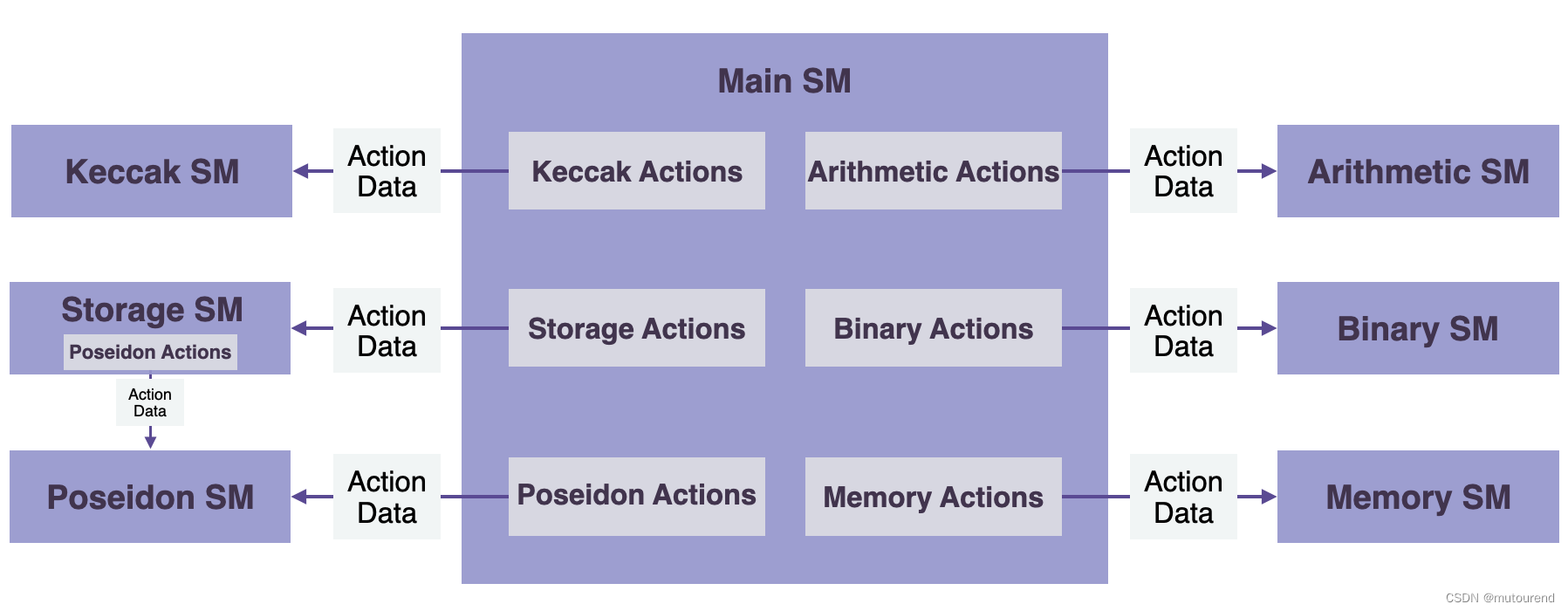
上图灰色方框即表示的是Actions。这些指令会命令 某state machine内的state如何transition。无论是来自Main SM还是来自特定SM的“Action”，每个Action都必须附加一个proof以证明其执行的正确性。
SM之间有一些相互依赖：

• 1）Storage SM会使用Merkle Trees和Poseidon SM，因其需要计算Storage Merkle Tree中所有节点的哈希值。
• 2）Keccak SM和Poseidon SM均为哈希状态机，对应的辅助状态机有Padding-KK SM和Padding-PG SM。

3.1.2 zkProver的2种编程语言

Polygon Hermez团队为zkProver创建了2种编程语言：

• 1）zkASM：Zero-Knowledge Assembly language。zkASM负责将zkProver Main SM的指令映射到其它SM。若SM有firmware，则zkASM为该firmware的解析器。
  zkASM代码的输入为 来自Main SM的指令，输出为 生成特定SM Executor如何运行计算的特定assembly codes。该Executor会严格遵循zkASM代码的规则和逻辑，从而可很容易地验证计算。

• 2）PIL：Polynomial Identity Language。因为几乎所有的状态机都将计算表示为多项式。状态机内状态变化必须满足特定计算相关的polynomial identities。
  Polygon zkEVM项目的主要目的是解决区块链不可能三角问题：隐私性、安全性、可扩展性，其本质是一种高效零知识承诺机制。而当前最安全和高效的承诺机制为多项式承诺机制，将计算转换成某种多项式语言是有利的，在这种语言中，验证归结为测试执行是否满足某些多项式恒等式（identities）。
  zkProver状态机内的所有PIL代码，构成了verifier code的DNA。
  Polynomial Identity Language（PIL）为定义state machine execution trace约束的领域特定语言。
  PIL用于：

  • 定义execution trace多项式name
  • 描述多项式identities
  • 描述多项式之间关系

  以满足execution是正确的。

3.1.3 Micro-Processor Context——Main SM和Storage SM

zkProver中有2种微处理器类型的状态机：

• Main SM
• Storage SM

这2种微处理器类型的状态机有：

• 1）firmware 固件部分：运行zkASM语言，以设置逻辑和规则，以JSON格式表示并存储在ROM中。特定的SM Executor将解析该JSON文件，然后根据JSON文件中的规则和逻辑执行Storage Actions。
• 2）hardware 硬件部分：运行PIL语言，以定义约束（或polynomial identities），以JSON格式表达并存储在相应的JSON文件内。与firmware类型，特定的SM Executor会解析这些约束，因为所有的计算都必须与polynomial identities一致。

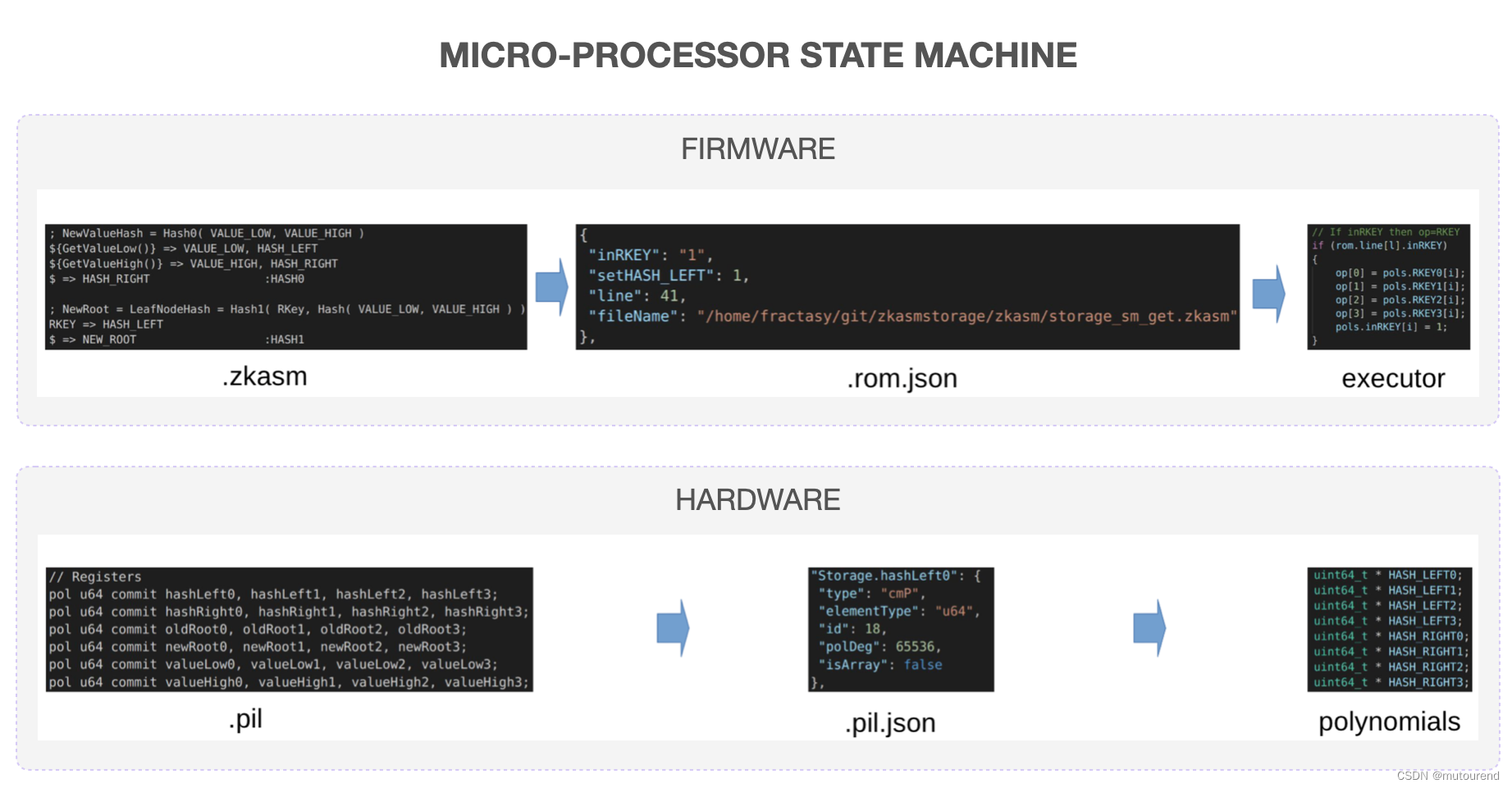
尽管Main SM和Storage SM看起来跟上图一样，但是二者区别很大：

• Storage SM擅长执行Storage Actions（又名SMT Actions），而Main SM负责更广范围的Actions。
• Main SM将大多数的Actions委托给专门的状态机，Storage SM是Secondary状态机——因其也从Main SM接收指令，但Storage SM无法给Main SM发送指令。

值得注意的是，每个微处理SM有各自的ROM。

3.1.4 zkProver中的哈希

有2个二级状态机负责哈希运算，二者均为标准密码学哈希函数的“automised”版本：

• 1）Keccak SM：为gates state machine，有一系列逻辑门（硬件）和一系列门（逻辑）之间的connections。由Keccak SM Hash Generator和Keccak PIL code组成，其中Keccak PIL code用于validation。
• 2）Poseidon SM：Poseidon哈希要比Keccak哈希算法更新，当前仍处于密码分析师的scritiny中，为zk-STARK-friendly hash function，其很适合于zkProver上下文。
  若熟悉原始Poseidon哈希函数的内部机制，Poseidon SM的实现非常直观。
  哈希函数的permutation过程很容易转化为Poseidon SM的状态转换。哈希函数的12个输入元素、非线性替换层（S-boxes）和线性扩散层（MDS矩阵）直接在状态机中实现。
  尽管Poseidon SM是二级状态机，它同时会从Main SM和Storage SM中接收指令。
  Poseidon SM有：
  • 2.1）executor部分
  • 2.2）internal PIL code：为验证规则集，以PIL语言编写。

3.2 证明每个状态机内计算正确执行的基本方法

zkProver的状态机设计为：

• 运行程序
• 并证明“程序执行正确”

每个二级状态机内包含了：

• 1）其自身的executor
• 2）一个PIL程序：用于检查其收到的源自Main SM的指令执行正确。

系统实现交易的证明和验证的基本流程为：

• 1）将某指定计算表示为状态机SM。
• 2）将该状态机的状态变化表示为多项式。
• 3）跟踪状态变化的trace，称为execution trace，作为lookup表的行。
• 4）形成该状态变化所满足的polynomial identities/constraints。
• 5）“Prover”采用特定的多项式承诺机制来承诺和证明其知道所commit的多项式。
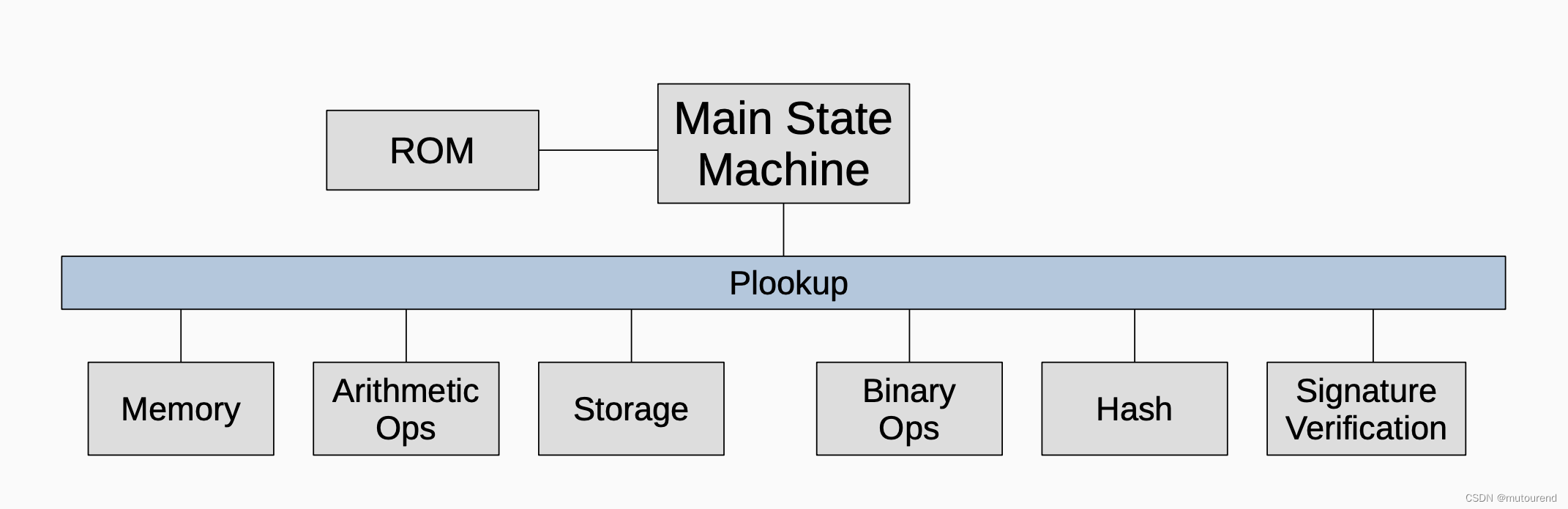
• 6）Plookup是一种检查Prover的committed polynomials 是否produce correct trace的方法。

多项式约束以PIL语言编写，而指令初始以zkASM语言编写，然后以JSON格式表达和存储。
尽管并不是所有的verification都包含了Plookup，但确实Plookup在zkProver中承担的了重要角色：

3.3 zkProver核心元素

zkProver主要有4大核心元素：

• 1）Executor，此处是指Main SM Executor：以交易、执行交易前的old states root、执行交易后的new states root、Sequencer的ChainID等，以及 PIL（为一组多项式、寄存器）和ROM（存储了需执行的指令集）为输入，Executor在PIL hardware层执行所有指令，并生成committed多项式——为state machine cycles 或 a list of all the states，同时还会生成某些public data——作为zk-SNARK Verifier的输入。【Main SM Executor负责将交易和相关数据转换为committed多项式】
• 2）STARK Recursion元素：输入有：committed多项式、constant多项式、scripts（指令集），输出为a zk-STARK proof。为了利用zk-STARK的快速证明，STARK Recursion中为每个zk-proof利用了FRI。之所以称为STARK Recursion，是因为：【即数百个zk-STARK proof会递归证明为一个zk-STARK proof】
  • a）其确实生成了多个zk-STARK proof
  • b）将这些zk-STARK proof整理捆绑为少量几个zk-STARK proof bundle
  • c）为每个bundle生成了zk-STARK proof
  • d）该bundle的zk-STARK proof会进一步整理并证明为一个zk-STARK proof。
• 3）CIRCOM库：输入为：STARK Recursion元素生成的单个zk-STARK proof、Verifier Data，输出为：Witness。zkProver使用CIRCOM circuit库 来生成witness for the zk-STARK proof produced by the STARK Recursion Component。【采用CIROM库，生成的witness本质就是以R1CS约束来表达的Arithmetic circuit。】
• 4）zk-SNARK Prover：为Rapid SNARK，以C++和Intel汇编语言编写，为CIRCOM输出快速生成证明。输入为：CIRCOM生成的witness、STARK verifier data（以表明Rapid SNARK如何处理该数据），输出为：a zk-SNARK proof。

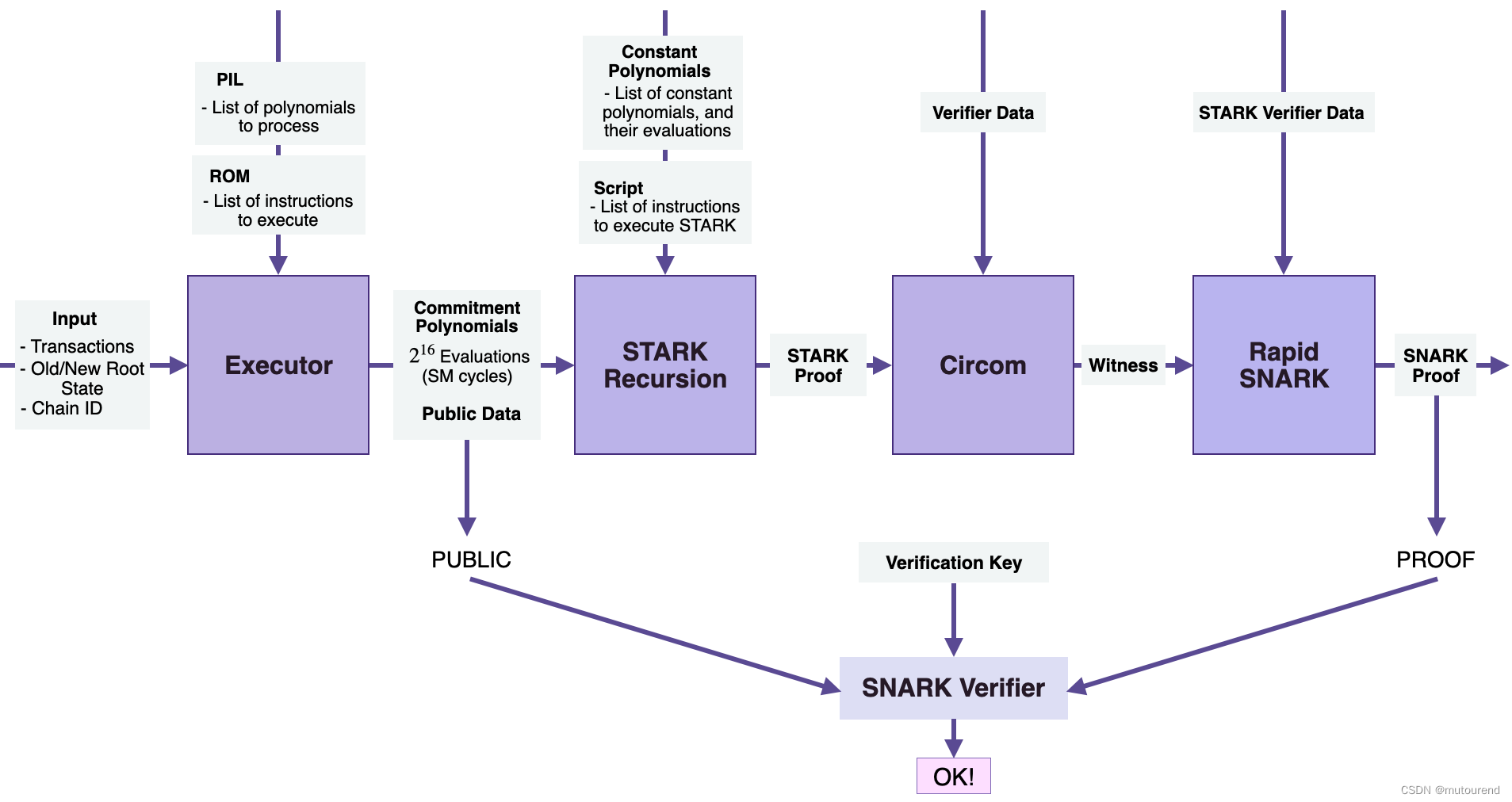
采用zk-STARK是因其证明速度很快，无需trusted setup，但是，zk-STARK的proof size很大，为此，引入zk-SNARK来证明zk-STARK proof的正确性，然后将zk-SNARK proof作为状态变化的validity proof发送到L1。借助zk-SNARK，验证validity proof的gas开销由500万降低为35万。

其中的rapidsnark为Iden3团队的：

• snarkjs工具

4. Modular Design

对于如EVM这样的复杂state machine，其execution trace对应的列数量和identities数量可达数千。而管理如此庞大的矩阵将是非常复杂且难处理的。

为简化，Polygon zkEVM使用divide and conquer技术，将execution trace切分为更小的矩阵。
使用名为plookup的proving技术，使得一个矩阵的行 可 关联到 另一矩阵的行 成为可能。

此外，还使用了：

• inclusion：检查某矩阵的行 被包含 在另一矩阵中。
• permutation：检查某矩阵中的行 与 另一矩阵中的行 相同，只是顺序不同。

PIL语言：

• 使用namespace关键字来命名execution trace中切分的每个矩阵的列。
• 使用in关键字来定义inclusion。
• 使用is关键字来定义permutation。

Polygon zkEVM中，会将execution trace切分为：

• 一个主矩阵：又名main state machine。
• 多个二级矩阵：又名secondary state machine。

借助divide and conquer技术：

• Polygon zkEVM中包含了不同的connected state machine。
• 每个state machine致力于证明某特定task的execution。
• 不同state machines之间的相关列（polynomials）使用inclusion proof（with plookup）来关联。

根据Polygon团队Jordi Baylina在2022年4月的分享Jordi Baylina - How we are building the zkEVM 可知：

5. 一个例子——Fibonacci state machine

Fibonacci序列：${\mathbf{a}}_1,{\mathbf{a}}_2,\dots ,{\mathbf{a}}_{\mathbf{n}}$，满足${\mathbf{a}}_{\mathbf{i}+1}={\mathbf{a}}_{\mathbf{i}-1}+{\mathbf{a}}_{\mathbf{i}}$。

如具有12个成员的序列：
$$0,1,1,2,3,5,8,13,21,34,55,89,\dots$$
很容易检查发现 $377$和$987$是Fibonacci 序列成员。但若要判断$12,586,269,025$是否为序列成员，则需要一个公式 或 计算机程序——本文以state machine密码学工具来实现。

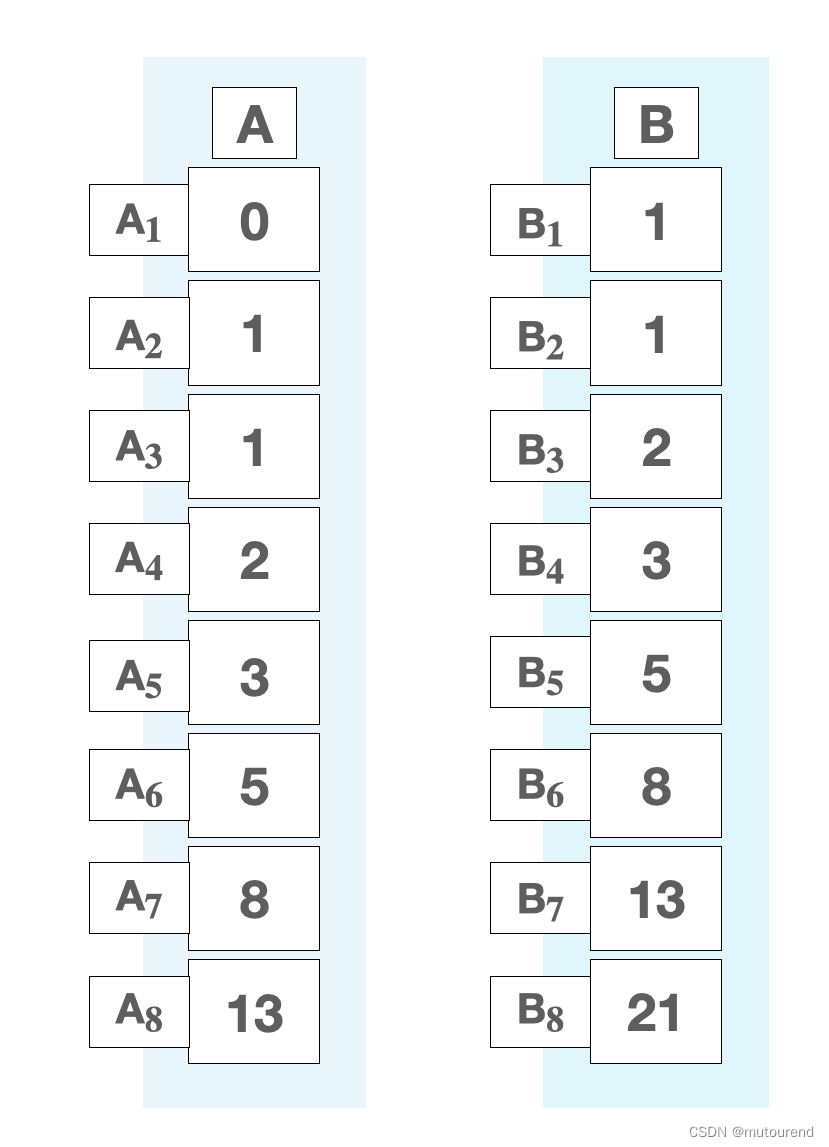
5.1 Fibonacci State Machine

具有寄存器$\mathbf{A}=(A_1,A_2,\dots ,A_l)$和$\mathbf{B}=(B_1,B_2,\dots ,B_l)$的状态机，第$i$个状态为$(A_i,B_i)$，初始状态为$A_1=0,B_1=1$。

$\mathbf{A}和\mathbf{B}$寄存器均包含了Fibonacci序列，只是$\mathbf{B}$中的序列为one step ahead of $\mathbf{A}$，二者具有如下关系：
$$\begin{array}{rl}{A}_{i+1}& =B_i,\\ B_{i+1}& =A_i+B_i.\end{array}$$
接下来，是将这些寄存器以多项式表示，并最终引入多项式承诺机制来构建该membership密码学工具。

5.1.1 polynomial identities

区块链传统中，将2个寄存器的多项式表示为${\mathbb{Z}}_p[x]$，其系数源自a prime field ${\mathbb{Z}}_p$。
将多项式evaluate over subgroup of H = { ω , ω 2 , ω 3 , … , ω 8 = 1 } = ⟨ ω ⟩ H = \{\omega,\omega^2,\omega^3,\dots,\omega^8 = 1\} = \langle\omega\rangle H={ω,ω2,ω3,…,ω8=1}=⟨ω⟩ of order $8$。
定义多项式$P(x)$和$Q(x)$为：
$$\begin{gathered} P({\omega }^i)=A_i, \\ Q({\omega }^i)=B_i. \end{gathered}$$

$H$中的每个$x$形式为$x={\omega }^i$ for some $i$，从而有：
$$\begin{array}{rl}P(x\omega )& =P({\omega }^{i+1})=A_{i+1},\\ Q(x\omega )& =Q({\omega }^{i+1})=B_{i+1}.\end{array}$$
将关系$A_{i+1}=B_i$ and $B_{i+1}=A_i+B_i$代入，获得如下polynomial identities：
$$\begin{array}{rl}P(x\omega )& =A_{i+1}=B_i=Q({\omega }^i)={|}_{H}Q(x),\\ Q(x\omega )& =B_{i+1}=A_i+B_i=P({\omega }^i)+Q({\omega }^i)={|}_{H}P(x)+Q(x).\end{array}$$
即：
$$\begin{array}{rl}P(x\omega )& ={|}_{H}Q(x),\\ Q(x\omega )& ={|}_{H}P(x)+Q(x).\end{array}$$

5.1.2 Non-cyclic Polynomial Identities Problem

若以上这些polynomial identities可准确表达2个寄存器，则Fibonacci序列中的每个成员均可满足该identities。

注意，$H$的定义并未限定$i\le 8$，若设置$i=27$，则${\omega }^{27}$ is in $H$，因为${\omega }^{27}=w^8\cdot {\omega }^8\cdot {\omega }^8\cdot {\omega }^3=1\cdot 1\cdot 1\cdot {\omega }^3={\omega }^3$。

但是不限定$i$值，以上polynomial identities会有问题。如令$x={\omega }^8$，有：

• 对于第一个identity有：
  $$\begin{array}{rl}& P(x\omega )=P({\omega }^8\cdot \omega )=P({\omega }^1)=A_1=0,\text{but}\\ & Q(x)=Q(w^8)=B_8=21\ne 0.\end{array}$$
• 对于第二个identity有：
  $$\begin{array}{rl}& Q(x\omega )=Q(\omega )=B_1=1\\ & P(x)=P({\omega }^8)+Q({\omega }^8)=21+13=34\ne 1.\end{array}$$

这意味着，尽管$H$是cyclic的，但是identities并不是cyclic的，polynomial identities 与 Fibonacci state machine寄存器 并不匹配。

为此，需要引入纠错多项式$R(x)$，使得polynomial identities也是cyclic的。纠错多项式也必须in ${\mathbb{Z}}_p[x]$。

5.1.3 Correcting Errors in Polynomial Identities

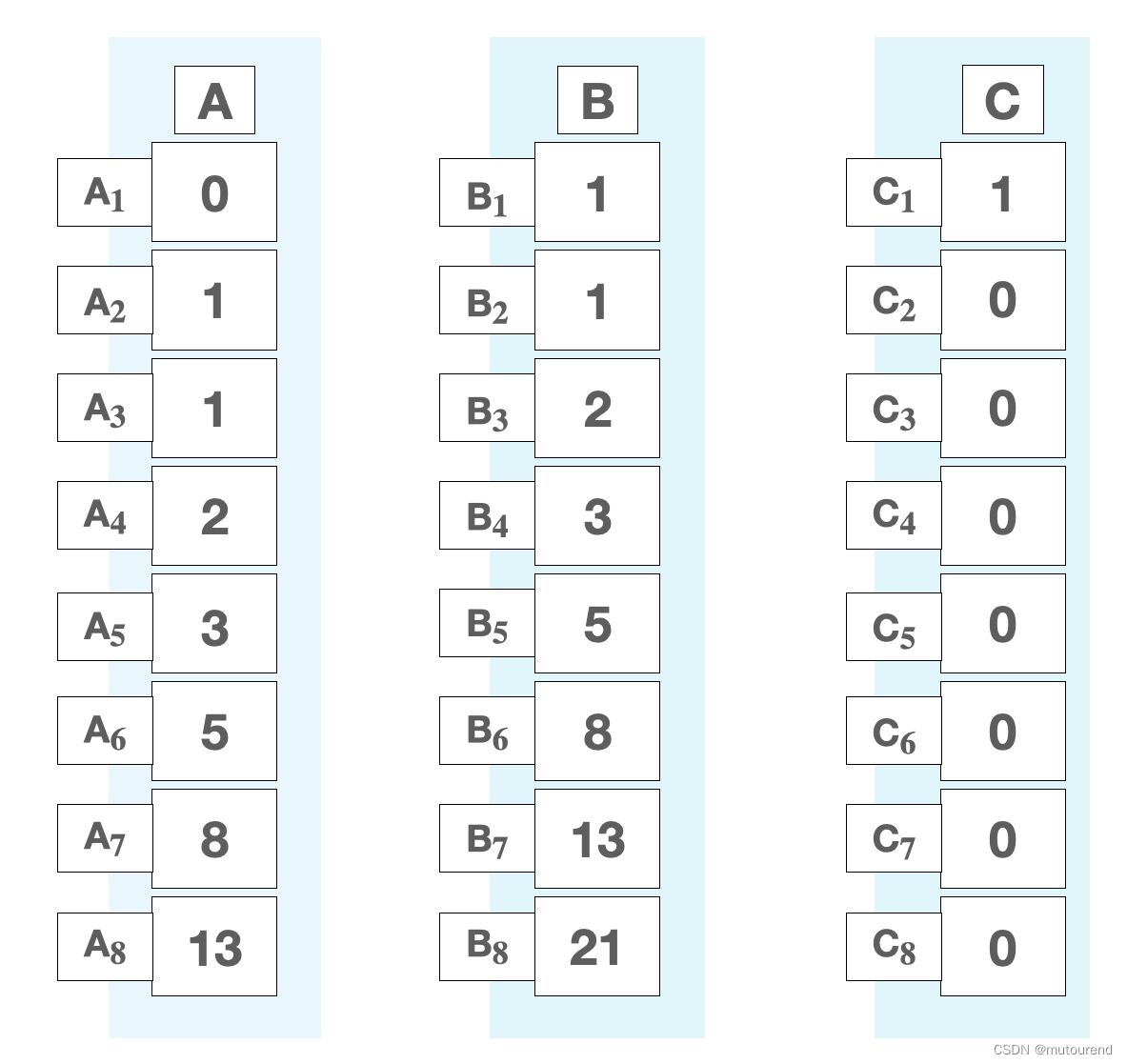
为Fibonacci state machine引入第三个寄存器 $\mathbf{C}=(C_1,C_2,\dots ,C_l)$，设置该寄存器的值为$\mathbf{C}=(1,0,0,\dots ,0)$。

将纠错多项式$R(x)$定义为：
$$R({\omega }^i)=C_i.$$
即：
$$\begin{array}{rl}R({\omega }^i)& =C_i=1,\text{ if }i\mathrm{m}\mathrm{o}\mathrm{d}8=1,\\ R({\omega }^i)& =C_i=0,\text{ otherwise}.\end{array}$$
将纠错多项式$R(x)$嵌入到之前的polynomial identities，有：
$$\begin{array}{rl}P(x\omega )& ={|}_{H}Q(x)(1-R(x\omega )),\\ Q(x\omega )& ={|}_H(P(x)+Q(x))(1-R(x\omega ))+R(x\omega )\end{array}$$

接下来，仍然设置$x={\omega }^8$，来检查新的polynomial identities是否是cyclic的：

• 对于第一个identity有：
  $$\begin{array}{rl}LHS& =P(x\omega )=P({\omega }^8\cdot \omega )=P({\omega }^1)=A_1=0,\\ RHS& =Q(x)(1-R(x\omega ))=Q({\omega }^8)(1-R({\omega }^8\cdot \omega ))=A_8(1-R(\omega ))=13(1-1)=0.\end{array}$$
  因此当$x={\omega }^8$时，第一个identity成立。很容易检查当$x$为$H$中的其它值时，也成立。
• 对于第二个identity有：
  $$\begin{array}{rl}LHS& =Q(x\omega )=Q({\omega }^8\cdot \omega )=Q({\omega }^1)=B_1=1,\\ RHS& =(P({\omega }^8)+Q({\omega }^8))(1-R({\omega }^8\cdot \omega ))+R({\omega }^8\cdot \omega )\\ & =(A_8+B_8)(1-R({\omega }^1))+R({\omega }^1)\\ & =(13+21)(1-1)+1=1.\end{array}$$
  因此当$x={\omega }^8$时，第二个identity成立。很容易检查当$x$为$H$中的其它值时，也成立。

5.1.4 Varied Initial Conditions

注意，此处不再限定初始条件为$(A_1,B_1)=(0,1)$。
将polynomial identities调整为适于任意初始条件$(A_1,B_1)$：
$$\begin{array}{rl}P(x\omega )& ={|}_{H}Q(x)(1-R(x\omega ))+A_{1}R(x\omega ),\\ Q(x\omega )& ={|}_H(P(x)+Q(x))(1-R(x\omega ))+B_{1}R(x\omega ).\end{array}$$

5.1.5 Proving Our State Machine（High level）

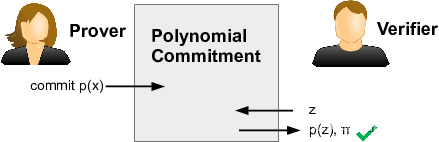
之前的多项式关系可通过如 KZG 和 基于FRI 的多项式承诺来证明。

承诺机制具有binding和hiding属性：

• 1）Binding：一旦commit之后，Prover无法修改多项式。
• 2）Hiding：仅知道承诺值，Verifier无法推导出Prover所commit的具体多项式。

6. 一个例子——Arithmetic State Machine

arithmetic state machine将检查32bit元素的加减乘除运算。
约束中有5个寄存器：
$${\mathcal{A}}_i\cdot {\mathcal{B}}_i+{\mathcal{C}}_i=2^{32}{\mathcal{D}}_i+{\mathcal{E}}_i.$$
注意，以上五个寄存器均为32bit的。

跟之前类似，将该关系表示为a cyclic polynomial identity at some subgroup $H$ of roots of unity of ${\mathbb{Z}}_p$：
$$\mathcal{A}(x)\cdot \mathcal{B}(x)+\mathcal{C}(x)=2^{32}\mathcal{D}(x)+\mathcal{E}(x).$$

注意，此处需要 enforce $\mathcal{A}(x)$, $\mathcal{B}(x)$, $\mathcal{C}(x)$, $\mathcal{D}(x)$ 和 $\mathcal{E}(x)$ 在 $H$ 的值均为32bit。

参考资料

[1] Polygon zkEVM Basic Concepts