
The Heaviside step function, H, also called the unit step function, is a discontinuous function whose value is zero for negative argument and one for positive argument. It seldom matters what value is used for H(0), since H is mostly used as a distribution. Some common choices can be seen below.
The function is used in the mathematics of control theory and signal processing to represent a signal that switches on at a specified time and stays switched on indefinitely. It was named after the English polymath Oliver Heaviside.
It is the cumulative distribution function of a random variable which is almost surely 0. (See constant random variable.)
The Heaviside function is the integral of the Dirac delta function: H′ = δ. This is sometimes written as
although this expansion may not hold (or even make sense) for x = 0, depending on which formalism one uses to give meaning to integrals involving δ.
Contents []- 1 Discrete form
- 2 Analytic approximations
- 3 Representations
- 4 H(0)
- 5 Antiderivative and derivative
- 6 Fourier transform
- 7 See also
- 8 References
We can also define an alternative form of the unit step as a function of a discrete variable n:
where n is an integer.
The discrete-time unit impulse is the first difference of the discrete-time step
This function is the cumulative summation of the Kronecker delta:
where
is the discrete unit impulse function.
[ edit] Analytic approximationsFor a smooth approximation to the step function, one can use the logistic function
-
 ,
,
where a larger k corresponds to a sharper transition at x = 0. If we take H(0) = ½, equality holds in the limit:
There are many other smooth, analytic approximations to the step function.[1] They include:
While these approximations converge pointwise towards the step function, the implied distributions do not strictly converge towards the delta distribution. In particular, the measurable set
has measure zero in the delta distribution, but its measure under each smooth approximation family becomes larger with increasing k.
[ edit] RepresentationsOften an integral representation of the Heaviside step function is useful:
The value of the function at 0 can be defined as H(0) = 0, H(0) = ½ or H(0) = 1. H(0) = ½ is the most consistent choice used, since it maximizes the symmetry of the function and becomes completely consistent with the sign function. This makes for a more general definition:
To remove the ambiguity of which value to use for H(0), a subscript specifying the value may be used:
The ramp function is the antiderivative of the Heaviside step function: 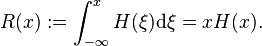
The derivative of the Heaviside step function is the Dirac delta function: dH(x) / dx = δ(x).
[ edit] Fourier transformThe Fourier transform of the Heaviside step function is a distribution. Using one choice of constants for the definition of the Fourier transform we have
Here the  term must be interpreted as a distribution that takes a test function φ to the Cauchy principal value of
term must be interpreted as a distribution that takes a test function φ to the Cauchy principal value of 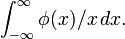
- Rectangular function
- Step response
- Sign function
- Negative and non-negative numbers
- Laplace transform
- Iverson bracket










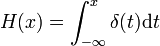
![H[n]=\begin{cases} 0, & n < 0 \\ 1, & n \ge 0 \end{cases}](http://upload.wikimedia.org/math/7/4/1/7410747ec7563eab51f608f2c80a9497.png)
![\delta\left[ n \right] = H[n] - H[n-1].](http://upload.wikimedia.org/math/1/8/b/18b1fdb556783d82836628433d71fa6d.png)
![H[n] = \sum_{k=-\infty}^{n} \delta[k] \,](http://upload.wikimedia.org/math/8/a/c/8ac2212bc01e69e22245f783f82146fd.png)
![\delta[k] = \delta_{k,0} \,](http://upload.wikimedia.org/math/4/3/0/430fc704633ce64f5d7aa81d9d45df7c.png)

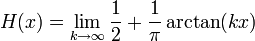

![\bigcup_{n=0}^{\infty}\left[ 2^{-2n};2^{-2n+1}\right]](http://upload.wikimedia.org/math/7/5/8/7580dfa7064d435433f04ba3e010557f.png)

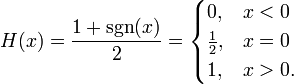

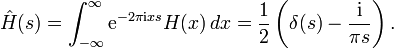














 8272
8272

 被折叠的 条评论
为什么被折叠?
被折叠的 条评论
为什么被折叠?








