nyist 148 fibonacci数列(二)

fibonacci数列(二)

时间限制： 1000 ms  |  内存限制： 65535 KB

难度： 3

描述

In the Fibonacci integer sequence, F₀ = 0, F₁ = 1, and F_n = F_{n − 1} + F_{n − 2} for n ≥ 2. For example, the first ten terms of the Fibonacci sequence are:

0, 1, 1, 2, 3, 5, 8, 13, 21, 34, …

An alternative formula for the Fibonacci sequence is

.

Given an integer n, your goal is to compute the last 4 digits of F_n.

 

Hint

As a reminder, matrix multiplication is associative, and the product of two 2 × 2 matrices is given by

.

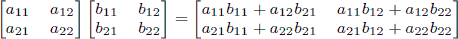Also, note that raising any 2 × 2 matrix to the 0th power gives the identity matrix:

.

输入

The input test file will contain multiple test cases. Each test case consists of a single line containing n (where 0 ≤ n ≤ 1,000,000,000). The end-of-file is denoted by a single line containing the number −1.

输出

For each test case, print the last four digits of Fn. If the last four digits of Fn are all zeros, print ‘0’; otherwise, omit any leading zeros (i.e., print Fn mod 10000).

样例输入

0
9
1000000000
-1

样例输出

0
34
6875

解题方法：
使用矩阵的来解决
1,1
1,0
的n次幂就能得到我们要的结果了
证明和具体的思路课参照网上的资料

下面是实现代码

 1 #include <cstdio>
 2 #include <cstring>
 3 #include <iostream>
 4 
 5 using namespace std;
 6 
 7 #define N 10000
 8 
 9 typedef struct matrix
10 {
11     long long a[2][2];
12     matrix()
13     {
14         memset(a,0,sizeof(a));
15     }
16 }matrix;
17 
18 void  chenfa(matrix x,matrix y,matrix &z)
19 {
20 
21         z.a[0][0] = (x.a[0][0]*y.a[0][0] + x.a[0][1]*y.a[1][0]) % N;
22         z.a[0][1] = (x.a[0][0]*y.a[0][1] + x.a[0][1]*y.a[1][1]) % N;
23         z.a[1][0] = (x.a[1][0]*y.a[0][0] + x.a[1][1]*y.a[1][0]) % N;
24         z.a[1][1] = (x.a[1][0]*y.a[0][1] + x.a[1][1]*y.a[1][1]) % N;
25 }
26 
27 void kuaishu(matrix x,matrix &z,long long n)
28 {
29     z.a[0][0] = 1;
30     z.a[0][1] = 0;
31     z.a[1][0] = 0;
32     z.a[1][1] = 1;
33 
34     while(n)
35     {
36         if(n % 2 == 1)
37             chenfa(z,x,z);
38         n /= 2;
39         chenfa(x,x,x);
40     }
41 
42 }
43 
44 /*void print(matrix z)
45 {
46     int i = 0;
47     int j = 0;
48     for(i = 0; i< 2; i++)
49         {
50             for(j = 0; j< 2;j++)
51                 printf("%d ",z.a[i][j]);
52                 printf("\n");
53         }
54 }
55 */
56 int main()
57 {
58     long long n;
59     while(1)
60     {
61         scanf("%lld",&n);
62         if(n == -1)
63             break;
64 
65         if(n < 3)
66         {
67             if(n == 0)
68                 printf("0\n");
69             else
70             printf("1\n");
71         }
72         else
73         {
74             matrix z;
75             matrix x;
76             x.a[0][0] = 1;
77             x.a[0][1] = 1;
78             x.a[1][0] = 1;
79             x.a[1][1] = 0;
80             kuaishu(x,z,n);
81             //print(z);
82             printf("%lld\n",z.a[0][1]);
83         }
84     }
85     return 0;
86 }

 

转载于:https://www.cnblogs.com/yyroom/archive/2013/04/14/3019874.html