贪心（百练1328）：安放雷达（区间问题）

总时间限制:

1000ms

内存限制:

65536kB

描述

Assume the coasting is an infinite straight line. Land is in one side of coasting, sea in the other. Each small island is a point locating in the sea side. And any radar installation, locating on the coasting, can only cover d distance, so an island in the sea can be covered by a radius installation, if the distance between them is at most d.

We use Cartesian coordinate system, defining the coasting is the x-axis. The sea side is above x-axis, and the land side below. Given the position of each island in the sea, and given the distance of the coverage of the radar installation, your task is to write a program to find the minimal number of radar installations to cover all the islands. Note that the position of an island is represented by its x-y coordinates.

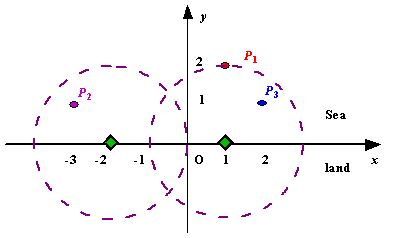
Figure A Sample Input of Radar Installations

输入

The input consists of several test cases. The first line of each case contains two integers n (1<=n<=1000) and d, where n is the number of islands in the sea and d is the distance of coverage of the radar installation. This is followed by n lines each containing two integers representing the coordinate of the position of each island. Then a blank line follows to separate the cases.

The input is terminated by a line containing pair of zeros

输出

For each test case output one line consisting of the test case number followed by the minimal number of radar installations needed. "-1" installation means no solution for that case.

样例输入

3 2
1 2
-3 1
2 1

1 2
0 2

0 0

样例输出

Case 1: 2
Case 2: 1

题目大致意思是，x轴为海岸线，上方有坐标为(x,y)的岛屿x,y为整数,已知雷达的探测范围为d(整数),问至少需要多少个雷达能他覆盖到所有的岛屿。如果有岛屿不能被探测到，则输出-1，否则输出最少雷达个数

思路：把雷达能探测到的位置转化为以，岛屿为圆心，d为半径的圆在x轴上截的长度区间（在此区间内可以探测到该岛屿，

把所有的区间按照升序排列，然后选择某个区间左端点，并且与其之前的没有被确定为已经被探测的区间的右端点进行比较，如果小于之前的点的右端点，则说明这个位置安放雷达可以探测到这些岛屿，继续这个过程，直到某个岛屿不能被探测，说明之前几个区间需要安放一个雷达，记录此时位置，并且下一轮从该位置记录比较。

此题巧妙之处就是，按照区间左端点升序排列，此时，后面的区间起始处已经默认小于之前的区间的左端点，此时只需要比较其起始点，是否也比右端点小就可以判断是否可以同时探测。

按照这个思想，我觉得，也可以按照右端点降序排列，此时之前的区间的右端点已经默认小于该点右端点，可以按照类似方法进行判断。

学习到了：数据读入时即使不满足条件，也要把此次数据读完再结束本次，否则直接影响之后的数据读取

#include<stdio.h>
#include<iostream>
#include<cmath> 
#include<algorithm>
#include<cstring>
using namespace std;

struct Node{
	double s,e;
	bool operator<(const Node o)const{
		return s < o.s; //按照 区间初始距离升序 
	}
};
int n,d;
struct Node nodes[1000+5];
int main()
	{
		int x,y;
		int kcase = 0;
		//freopen("C:/Users/zhangwei/Desktop/input.txt","r",stdin);
		while(cin >> n >> d && n!=0 && d != 0){
			bool flag = true; 
			for(int i = 0; i < n; ++i){
				scanf("%d%d",&x,&y);
				if(y > d){
					flag = false; //注意 不要在这里直接break， 否则本次数据没有读取完 
				}				 //影响下一组数据的读取 导致RuntimeError 
				double t = sqrt(d*d-y*y);
				nodes[i].s = x-t;//记录区间
				nodes[i].e = x+t;
			} 
		
			printf("Case %d:",++kcase); 
			if(!flag){
				printf(" -1\n");
				continue; //一旦有不可探测点结束 
			}
			sort(nodes,nodes+n);
			int firNoVis = 0;
			int ans = 1;//初始为1
			
			for(int i = 1; i < n; ++i){
				for(int j = firNoVis; j < i; ++j){
					if(nodes[i].s <= nodes[j].e){
						continue;
					}
					else{
						firNoVis = i;
						++ans;
						break;
					}
				}
			} 
			printf(" %d\n",ans);
			
		}
		
		
		return 0;
	}