Positive-definite matrix

最新推荐文章于 2023-02-13 21:41:40 发布

技术笔记本

最新推荐文章于 2023-02-13 21:41:40 发布

阅读量1.4k

点赞数

分类专栏：机器学习/深度学习

机器学习/深度学习专栏收录该内容

12 篇文章 1 订阅

订阅专栏

In linear algebra, a symmetric n × n real matrix M is said to be positive definite if z^TMz is positive for every non-zero columnvector z of n real numbers. Here z^T denotes thetranspose of z.

The real symmetric matrix

M = \begin{bmatrix} 2&-1&0\\-1&2&-1\\0&-1&2 \end{bmatrix}

is positive definite since for any non-zero column vector z with entries a, b and c, we have

\begin{align} z^{\mathrm{T}}M z = (z^{\mathrm{T}}M) z &= \begin{bmatrix} (2a-b)&(-a+2b-c)&(-b+2c) \end{bmatrix} \begin{bmatrix} a\\b\\c \end{bmatrix} \\&= 2{a}^2 - 2ab + 2{b}^2 - 2bc + 2{c}^2 \\&= {a}^2+(a - b)^{2} + (b - c)^{2}+{c}^2\end{align}

This result is a sum of squares, and therefore non-negative; and is zero only if a = b = c = 0, that is, when z is zero.

The real symmetric matrix

N = \begin{bmatrix} 1 & 2 \\ 2 & 1\end{bmatrix}

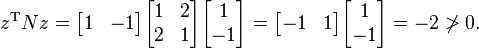
is not positive definite. If z is the vector $\begin{bmatrix} 1\\ -1\end{bmatrix}$ , one has $z^{\mathrm{T}}N z = \begin{bmatrix} 1 & -1\end{bmatrix} \begin{bmatrix} 1 & 2 \\ 2 & 1\end{bmatrix} \begin{bmatrix} 1 \\ -1\end{bmatrix}=\begin{bmatrix} -1 & 1\end{bmatrix} \begin{bmatrix} 1 \\ -1\end{bmatrix}=-2 \not > 0.$

More generally, an n × n Hermitian matrix M is said to be positive definite if z*Mz is real and positive for all non-zero complex vectors z. Here z* denotes the conjugate transpose of z.

摘自：https://en.wikipedia.org/wiki/Positive_semidefinite_matrix

技术笔记本

关注

0
点赞
踩
0

收藏

觉得还不错? 一键收藏
0
评论
Positive-definite matrix

In linear algebra, a symmetric n × n real matrix M is said to be positive definite if zTMz is positive for every non-zero columnvector z of n real numbers. Here zT denotes thetranspose
复制链接

扫一扫