POJ 2492 (树状数组统计数据)

Astronomers often examine star maps where stars are represented by points on a plane and each star has Cartesian coordinates. Let the level of a star be an amount of the stars that are not higher and not to the right of the given star. Astronomers want to know the distribution of the levels of the stars. 



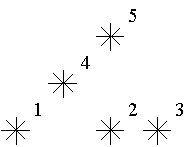
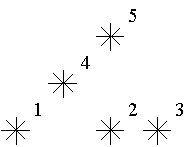
For example, look at the map shown on the figure above. Level of the star number 5 is equal to 3 (it's formed by three stars with a numbers 1, 2 and 4). And the levels of the stars numbered by 2 and 4 are 1. At this map there are only one star of the level 0, two stars of the level 1, one star of the level 2, and one star of the level 3. 

You are to write a program that will count the amounts of the stars of each level on a given map.

Input

The first line of the input file contains a number of stars N (1<=N<=15000). The following N lines describe coordinates of stars (two integers X and Y per line separated by a space, 0<=X,Y<=32000). There can be only one star at one point of the plane. Stars are listed in ascending order of Y coordinate. Stars with equal Y coordinates are listed in ascending order of X coordinate.

Output

The output should contain N lines, one number per line. The first line contains amount of stars of the level 0, the second does amount of stars of the level 1 and so on, the last line contains amount of stars of the level N-1.

Sample Input

5
1 1
5 1
7 1
3 3
5 5

Sample Output

1
2
1
1
0

题意：统计一个星星左下方的星星数量（包括在同一条水平线和竖直线上）

很经典的一道用树状数组解决的数据统计的问题，树状数组支持两个功能：1。向a［］表x位置既a［x］加上价值v 2.获得从a[1]到a[x]的的和

那么，就在a［x］位置上加一，sum（x）就可以了，注意本题中x可能为0，所以要加上1

讲解树状数组很好的两篇帖子：点击打开链接

点击打开链接

#include <iostream>
#include <cstring>
#include <cmath>
#include <cstdio>
using namespace std;
const int maxn=32010;
int c[maxn],a[maxn],ans[maxn];

inline int lowbit(int x)
{
    return (x&-x);
}

void add(int x,int value)
{
    for(int i=x;i<=maxn;i+=lowbit(i))
        c[i]+=value;
}

int sum(int x)
{
    int temp=0;
    for(int i=x;i;i-=lowbit(i))
        temp+=c[i];
    return temp;
}
int main()
{
    //freopen("/Users/zhangjiatao/Documents/暑期训练/input.txt","r",stdin);
    int n;
    while(scanf("%d",&n)!=EOF)
    {
        memset(c,0,sizeof(c));
        memset(a,0,sizeof(a));
        memset(ans,0,sizeof(ans));
        for(int t=1;t<=n;t++)
        {
            int x,y,temp;
            scanf("%d%d",&x,&y);
            temp=sum(x+1);
            ans[temp]++;
            add(x+1,1);
        }
        for(int i=0;i<n;i++) cout<<ans[i]<<endl;
    }
    return 0;
}