Description
We can change the matrix in the following way. Given a rectangle whose upper-left corner is (x1, y1) and lower-right corner is (x2, y2), we change all the elements in the rectangle by using "not" operation (if it is a '0' then change it into '1' otherwise change it into '0'). To maintain the information of the matrix, you are asked to write a program to receive and execute two kinds of instructions.
1. C x1 y1 x2 y2 (1 <= x1 <= x2 <= n, 1 <= y1 <= y2 <= n) changes the matrix by using the rectangle whose upper-left corner is (x1, y1) and lower-right corner is (x2, y2).
2. Q x y (1 <= x, y <= n) querys A[x, y].
Input
The first line of each block contains two numbers N and T (2 <= N <= 1000, 1 <= T <= 50000) representing the size of the matrix and the number of the instructions. The following T lines each represents an instruction having the format "Q x y" or "C x1 y1 x2 y2", which has been described above.
Output
There is a blank line between every two continuous test cases.
Sample Input
1 2 10 C 2 1 2 2 Q 2 2 C 2 1 2 1 Q 1 1 C 1 1 2 1 C 1 2 1 2 C 1 1 2 2 Q 1 1 C 1 1 2 1 Q 2 1
Sample Output
1 0 0 1
Source
#include<cstdio>
#include<string>
#include<iostream>
#include<string.h>
using namespace std;
int c[1005][1005],n;
int lowbit(int x)
{
return x&(-x);
}
void update(int x,int y,int k )
{
for(int i=x; i<=n; i+=lowbit(i))
for(int j=y; j<=n; j+=lowbit(j))
{
c[i][j]+=k;
}
}
int sum(int x,int y)
{
int ans=0;
for(int i=x; i>0; i-=lowbit(i))
for(int j=y; j>0; j-=lowbit(j))
ans+=c[i][j];
return ans;
}
int main()
{
int t,m,x1,x2,y1,y2;
char g[100];
scanf("%d",&t);
while(t--)
{
memset(c,0,sizeof(c));
scanf("%d %d",&n,&m);
for(int i=1; i<=m; i++)
{
scanf("%s",g);
if(g[0]=='C')
{
scanf("%d %d %d %d",&x1,&y1,&x2,&y2);
getchar();
update(x1,y1,1);
update(x1,y2+1,1);
update(x2+1,y1,1);
update(x2+1,y2+1,1);
}
else
{
scanf("%d %d",&x1,&y1);
getchar();
printf("%d\n",sum(x1,y1)%2);
}
}
printf("\n");
}
return 0;
}今天初步学习树状数组,虽然还有很多不懂,但这也是一个进步吧
下面是转载的一位大牛的博客
楼教主出的二维树状数组。
给出矩阵左上角和右下角坐标,矩阵里的元素 1变0 ,0 变1,然后给出询问,问某个点是多少。
纠结好久了,一直没什么好思路,看discuss说四个角神马的,我搜了下,理解了,树状数组里记录该点的变幻次数,或者直接%2也行。
查询的时候Getsum得到的是该点在所有区间的总变幻次数,最后%2就是结果。
建图的时候死活想不通,杂四个点的坐标是那个 = =。。。刚才协会开会了,在路上想通了,我想的0,0坐标是类似坐标轴的那种,在左下角。。。而矩阵的0 0 应该是在左上角。。这样,什么都通了 = =。。。
还有,更新的时候有的减1了,死循环了,发现错误了,树状数组里是不能使用下标为1的,所以更新的时候把下标都加一即可。
实验了下,%2和&1速度一样。。。
提供坐标的图。。
我见另一种做法,见 http://3214668848.blog.163.com/blog/static/48764919201052484413539/
就是原来的Updata改成Getsum。。。Getsum改成Updata。只不过每个坐标不用++了。
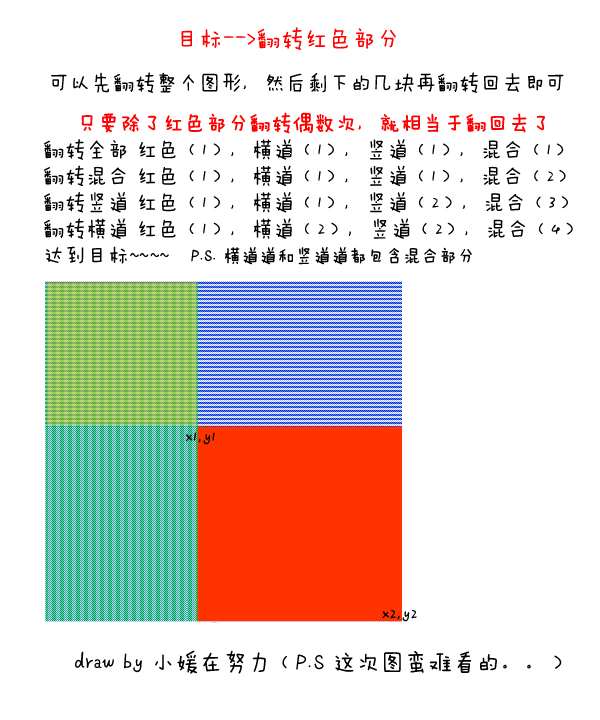
这题我还是有点迷茫的。刚才上机组课想了下,经过我画图以及认真分析(//害羞)代码,终于理解了。。。继续画图先。。

#include <queue>
#include <stack>
#include <math.h>
#include <stdio.h>
#include <stdlib.h>
#include <iostream>
#include <limits.h>
#include <string.h>
#include <algorithm>
using namespace std;
const int MAX = 1010;
int c[MAX][MAX];
int n;
int Lowbit(int x)
{
return x & (-x);
}
void Updata(int x,int y)
{
int i,k;
for(i=x; i<=n; i+=Lowbit(i))
for(k=y; k<=n; k+=Lowbit(k))
c[i][k]++;
}
int Get(int x,int y)
{
int i,k,sum = 0;
for(i=x; i>0; i-=Lowbit(i))
for(k=y; k>0; k-=Lowbit(k))
sum += c[i][k];
return sum;
}
int main()
{
int ncases,m;
int x1,y1,x2,y2;
char ch[2];
scanf("%d",&ncases);
while( ncases-- )
{
memset(c,0,sizeof(c));
scanf("%d%d",&n,&m);
while( m-- )
{
scanf("%s",ch);
if( ch[0] == 'C' )
{
scanf("%d%d%d%d",&x1,&y1,&x2,&y2);
x1++; y1++; x2++; y2++;
Updata(x2,y2);
Updata(x1-1,y1-1);
Updata(x1-1,y2);
Updata(x2,y1-1);
}
else
{
scanf("%d%d",&x1,&y1);
printf("%d/n",Get(x1,y1)%2);
}
}
printf("/n");
}
return 0;
}








 本文通过一个具体的编程问题,介绍了如何使用二维树状数组来高效处理矩阵上的操作和查询。文章详细解释了更新和查询的操作,并附上了实现代码。
本文通过一个具体的编程问题,介绍了如何使用二维树状数组来高效处理矩阵上的操作和查询。文章详细解释了更新和查询的操作,并附上了实现代码。
















 539
539

 被折叠的 条评论
为什么被折叠?
被折叠的 条评论
为什么被折叠?








