Perron–Frobenius theorem

Perron–Frobenius theorem:

转自： http://www.cnblogs.com/ZhangShuo/articles/1866748.html

证明方阵，如果其行列的元素为正值，则存在最大的特征值，并且特征向量的每个元素是正的。这个原理应用在统计推断，经济，人口统计学，搜索引擎的基础。

PF原理：说明了最大特征值的作用和实对称矩阵的作用。

如果临界矩阵A是个实对称矩阵，则具有下列性质：

1.There is a positive real number  r, called the  Perron root or the  Perron–Frobenius eigenvalue, such that  r is an eigenvalue of  A and any other eigenvalue  λ (possibly, complex) is strictly smaller than  r in  absolute value, | λ| <  r. Thus, the  spectral radius ρ( A) is equal to  r.

2.The Perron–Frobenius eigenvalue is simple:  r is a simple root of the  characteristic polynomial of  A. Consequently,  eigenspace associated to  r is one-dimensional. (Same is true for the left eigenspace i.e. eigenspace for  A^T.)

3.There exists an eigenvector  v = ( v ₁,…, v _n) of  A with eigenvalue  r such that all components of v are positive:  A v =  r v,  v _i > 0 for 1 ≤  i ≤  n. (Respectively exists positive left eigenvector  w :  w^T A =  r w^T,  w _i > 0.)转置就可以了。

4.There are no other positive (moreover non-negative) eigenvectors except  v (respectively the left eigenvectors except  w). I.e. all other eigenvectors must have at least one negative or complex component.

5. , where the left and right eigenvectors for  A are normalized so that w^Tv = 1. Moreover the matrix  v w^T is the  spectral projection corresponding to  r, such projection is called the  Perron projection.

6. Collatz–Wielandt formula: for all non-negative non-zero vectors  x let  f( x) be the minimum value of [ Ax] _i /  x _i taken over all those  i such that  x_i ≠ 0. Then  f is a real valued function whose  maximum is the Perron–Frobenius eigenvalue.

7.Min-max Collatz–Wielandt formula is similar to the one above: for all strictly positive vectors  x (pay attention that one above required only non-negativity here) let  g( x) be the maximum value of [ Ax] _i /  x _i taken over  i. Then  g is a real valued function whose  minimum is the Perron–Frobenius eigenvalue.

8.The Perron–Frobenius eigenvalue satisfies the inequalities：

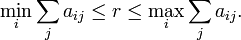
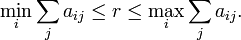
因为在科研中，比如计算pagerank,希望向量的每个值都是正的，在这里我着重介绍定理1,4的证明：

定理四启发式证明：由于不同特征值对应的特征向量是正交的，所以如果有一个特征值对应的特征向量的每个元素大于零，则其余的特征向量的分量要不然是负数要不然就是复数。

数学证明：设  (λ, y)为A的一个特征值和特征向量，(r, x)为A的另一个特征值和特征向量。则r x^t y = (x^t A) y= x^t (A y)=λ x^t y。所以r=λ。

定理一证明：矩阵除以矩阵的普半径，最大特征值在半径为1的单位园上。假设有不止一个特征值在这个单位圆上，最后得出矛盾来证明。