这篇博文是自己看文章顺手做的笔记 只是简单翻译和整理 仅做个人参考学习和分享
如果作者看到觉得内容不妥请联系我 我会及时处理
本人非文章作者,文献的引用格式如下,原文更有价值

摘要——
收集和讨论小型多旋翼无人机受地面效应的影响,尤其是从建模和控制的角度。
For a rotorcraft, an accident is likely to happen when the vehicle leaves or enters the ground effect region, which may cause crashes and property damages.
讨论关于地效的建模 控制和应用,以及其他一些类似的效应比如顶吸和墙吸
总结:
实验现象:
地面效应距离z<2R(有些多旋翼在z<5R都有观测到地效的影响),提供额外升力,或者说 保持总升力不变时降低了旋翼功率。利用近地面飞行可以节能约10%
顶吸效应距离z<2R
侧吸没有给生效的距离,但是,研究发现,对于靠近墙壁的叶片,升力会随着叶片方位角的变化而周期性波动。这些波动的升力在转子盘上产生力矩,力矩随转子-壁间距和姿态而变化。
方法上的讨论:
目前还是需要更好的感知和算法 - > 传感器数据融合和on-board实时数据处理
主要建模方法:实验数据建模 ,参数化建模,动力学建模
参数化建模可以更好的拓展在不同机型,因为可以定义旋翼直径,旋翼间隔,旋翼数等。
主要控制方法归为四类: 自适应,基于观测器,鲁棒,容错。控制器需要处理的包括 传感器问题,气动交互时的新模型。
应用方面,临近气动效应主要三个应用:提升控制效果,被动临近估计,节能导航。
应用方面的一个挑战在于:未知局限环境下的自动导航
未完成的工作:
包括对新模型的广泛验证、新模型在控制架构中的应用,以及在未知封闭环境中的自主导航,我们认为基于计算机视觉和机器人技术的人工智能技术可以利用新解决方案的出现
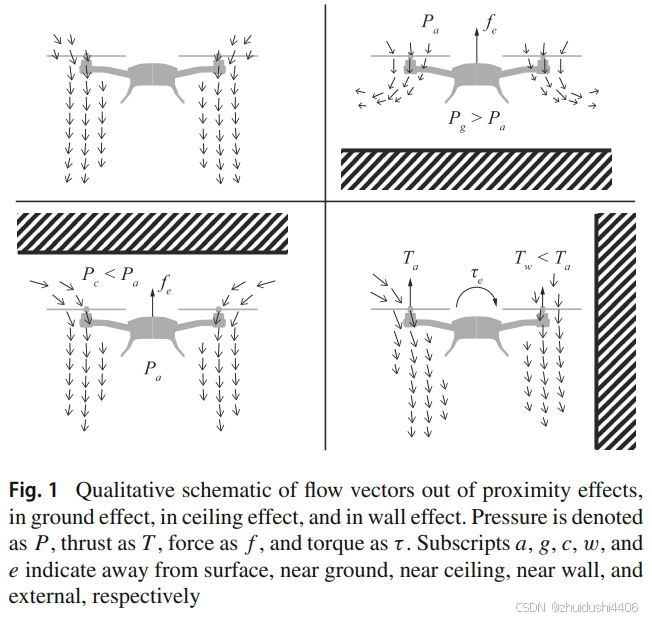
1 Introduction
. At the control level, a model of the GE can be incorporated into the control loop, allowing to track trajectories more accurately [11,12].
回顾了从1930至今关于地效研究的趋势,以及本文是第一次综述小型旋翼机的地效。
2 Background
悬停下的地效:
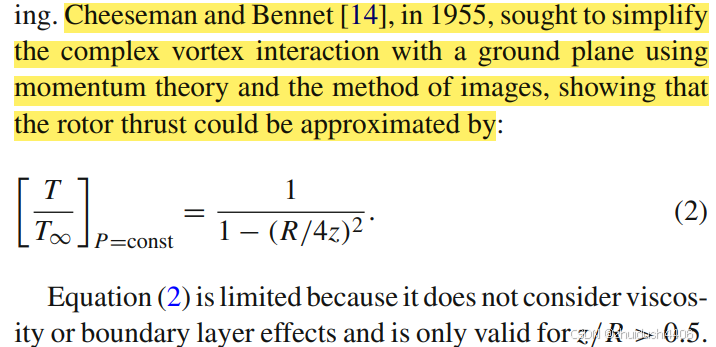
In all cases, it is apparent that the GE becomes negligible for rotors hovering greater than two rotor radii (one diameter) above the ground.
前飞时的地效:
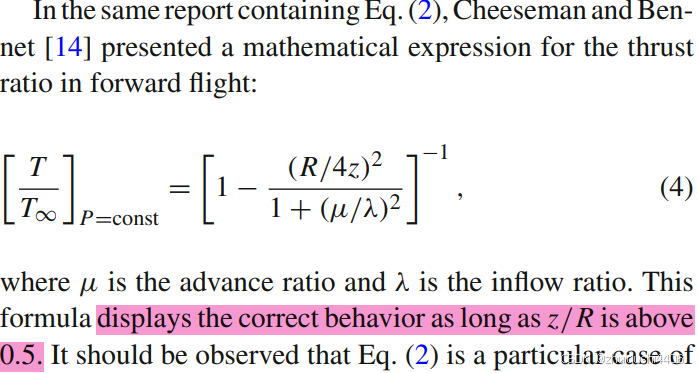
observed that the ground reduces the power required, but the effect diminishes with height more rapidly in forward flight. On the other hand, for small heights and speed near hover, the net power required can actually increase because of the formation and influence of flow recirculation at the leading edge of the rotor [42].
环境影响:
Bludau等人[44]承认任意环境的影响,提出了一种实时模型来预测物体附近的旋翼机运动。
2.1 Control
包含模型的方法中,a particular model of the rotor thrust is used, in which the thrust depends on the reduction of the induced velocity due to the GE.
height estimation and control is possible with only flow sensing and modeling.
2.2 Flow Studies
Research has been carried out about proximity flow effects on small rotors.
This claim motivated a work that used CFD to investigate the fluid interactions between a hovering small rotor and planar surfaces [54]. The computational method was validated by comparison against experimental results, a two-dimensional free vortex model, and results from existing literature. This study supposed that the variation in thrust generates a vertical force disturbance, Z¯, acting on the helicopter. Then, the relationship between Z¯ and ground proximity was characterized as:

Flight tests showed that the scheme can provide fair in-flight estimates of disturbances that are induced by the ground plane using only an onboard inertial measurement unit (IMU)
2.3 Modeling
The modeling of the GE for small rotors has attracted the attention of the UAV community.
3 Multirotor
Even though akin, helicopters and multirotors differ greatly on their mechanical structure and operation.
3.1 Control
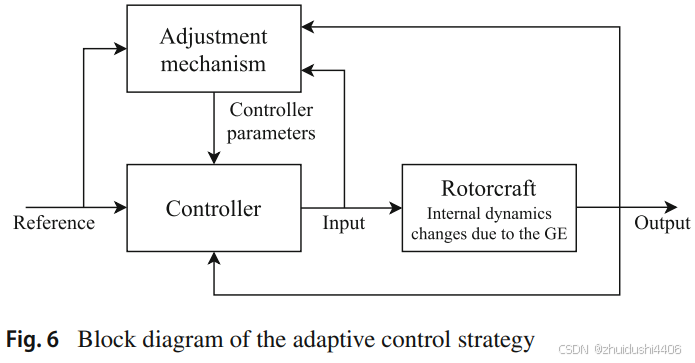
用的最多的方法是自适应控制
Guenard et al. [9] proposed an adaptive altitude controller that estimates online the global thrust coefficient of a quadcopter (assuming that the thrust coefficient is the same for all the rotors).
It was noted that using the adaptive controller and the estimator worked well in experiments with a quadrotor prototype (only results for heights above 0.5 m were reported, situation in which the GE might not be significant given the size of the prototype),‘
用观测器结合高度传感器 修改旋翼力效系数
Lee et al. [60] compared in simulations two nonlinear control methods for quadcopters: feedback linearization and adaptive sliding mode.
They considered an unknown acceleration term, representing the GE, while designing of the adaptive controller.
模型中加了一个函数来表示地效引起的加速度
发现传统反馈线性化应对非确定性和传感器噪声时不够鲁棒,自适应滑膜控制器相对较好。
同一作者,后来滑膜自适应的部分改成了神经网络,调整其权重来估计地效在z轴加速度,roll,pitch引起的干扰。在一个0.8kg无人机测试。结果说明效果提升了。
Hu et al. [61] proposed a control structure to achieve the landing of a quadrotor onto a vertically oscillating platform.
降落在高度垂直摆动的平台上,控制器包括动作估计,轨迹生成,自适应鲁棒跟踪。
The controller was executed in an offboard computer using measurements of vertical position from a MoCap system, and vertical acceleration from an IMU.
A pair of authors have explored model reference adaptive control (MRAC) for quadcopters operating close to the ground.
When the observer or estimator is used to estimate internal or external disturbances online in the control loop, the control strategy is often referred to as an active disturbance rejection control
Besides the increment in thrust efficiency, the GE causes variations in the pressure altitude measured by barometers [75] and distance measurements of ultrasonic sensors [9].
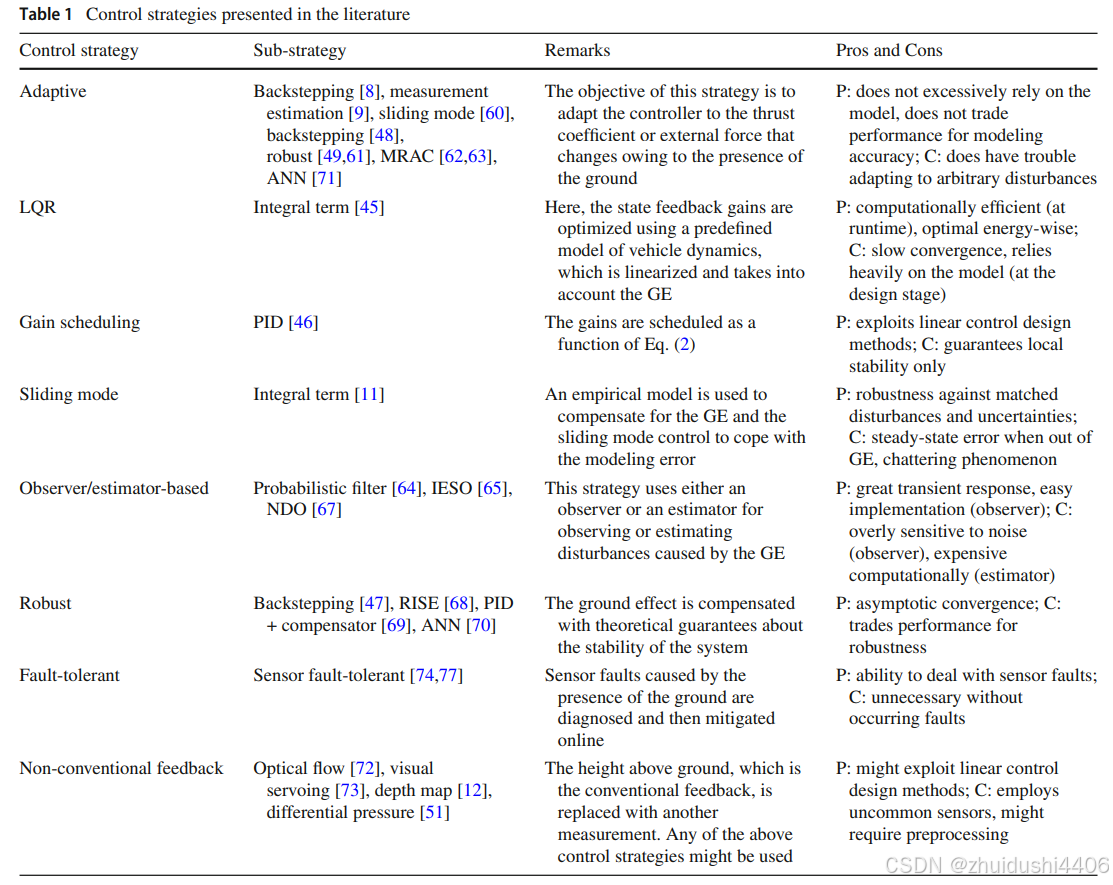
3.2 Modeling
A number of models of the GE for multirotors have been presented already in the previous subsection. However, the conception of those models has not been the primary concern and little or no details have been given about their validity. In this subsection, we will discuss the research that revolves around the modeling of the effect of the ground on multiro tors.
上一小节已经介绍了地面效应的多电机模型。然而,这些模型的概念并不是主要关注的问题,关于其有效性的细节很少或根本没有给出。在本小节中,我们将讨论围绕地面对多任务机影响建模的研究。
The earliest studies on GE modeling for multirotors were focused on applying or evaluating the classical mod els of thrust change obtained for helicopters. Bangura and Mahony [78] proposed a hybrid dynamic model to account for different flight states of the vehicle, including GE. The idea is to switch between states (normal, vortex ring, turbu lent wake state, windmill brake state, and GE) given some predefined conditions. Equation (4) (Cheeseman–Bennet model for forward flight) was used to represent the GE. In a different report [79], the4 inEq. (2) (Cheeseman Bennet model for hovering) was replaced with a constant that was later identified with empirical data collected for the kQuadNano. A similar procedure with the same quadro tor was reported by Powers et al. [80]. In their data, the GE can be observed up to z/R = 5, which is signifi cantly higher than predicted by Eq. (2). Sharf et al. [81] assessed the applicability of this model for the Draganflyer X8, which is a small rotary-wing UAV with eight propellers arranged in four coaxial pairs. Again, it was found that the GE was apparent at higher altitudes than predicted by the model.
对多旋翼地面效应建模的最早研究集中在应用或评估直升机推力变化的经典模型上。Bangura和Mahony[78]提出了一种混合动力模型,以考虑车辆的不同飞行状态,包括地面效应。其想法是在给定一些预定条件的状态(正常、涡流环、湍流尾流状态、风车制动状态和地面效应)之间切换。方程(4)(正向飞行的Cheeseman–Bennet模型)用于表示地面效应。在另一份报告[79]中,4 inEq。(2) (Cheeseman-Bennet悬停模型)被替换为一个常数,该常数后来被kQuadNano收集的经验数据所识别。Powers等人[80]报道了使用相同四分体的类似程序。在他们的数据中,可以观察到高达z/R=5的地面效应,这显著高于方程(2)的预测。Sharf等人[81]评估了该模型对Draganflyer X8的适用性,这是一种小型旋翼无人机,有八个螺旋桨,排列在四个同轴对中。再次,人们发现地面效应在比模型预测的更高的高度上是明显的。
The rotors that are used in model helicopters are dif ferent from the ones used in small multirotors. This has motivated the study of the GE in the hovering perfor mance of the latter. The work presented in [83] focused on developing a numerical method for predicting the thrust and power consumption in GE. The authors developed a model with blade element momentum theory which is com bined with Cheeseman–Bennet predictions. The resulting model provides a purely analytical method for model ing the GE performance of a specific propeller geometry. However, the authors do not explore potential interactions between multiple adjacent rotors. An experimental study [84] assessed the applicability of Eq. (2) for a small hover ing rotor (between 6 and 20 in). The results showed that this expression is a good approximation for small iso lated propellers too. In a follow-up publication [82], a method was developed which allows the generation of a map of aerodynamic effects in an environment with mul tiple objects. First, the aerodynamic effects close to surfaces were experimentally tested along a two-dimensional grid (Fig. 13a). Then, the data are combined to account for different objects in the aerodynamic map (Fig. 13b). On the same line, Bernard et al. [85] performed an exten sive experimental campaign aimed at characterizing the GE for a small quadcopter. In particular, both an isolated rotor and the complete platform were studied. The col lected results were compared against the Cheeseman–Bennet model and the model of Hayden, showing that the effect of the ground is extended up to almost z/R = 4. Cony ers et al. [86] went a step further by reporting empirical data for T/T∞ from two different spacings between pro pellers on a typical x-shaped quadrotor frame. The results suggest that Eq. (2) cannot accurately predict GE perfor mance for quadrotors. In his thesis, Conyers [87] presented more empirical data for different propeller sizes and propeller spacings. These data were used to fit a rational polynomial for T/T∞ as a function of normalized height x = z/R, propeller spacing L, and propeller radius. A compromise was made to use an equation with the lowest order while still producing a quality fit. The final equation form is defined by:
模型直升机中使用的旋翼与小型多旋翼中使用的不同。这推动了对地面效应悬停性能的研究。[83]中的工作重点是开发一种数值方法来预测地面效应的推力和功率消耗。作者开发了一个与Cheeseman–Bennet预测相结合的叶片单元动量理论模型。所得模型为特定螺旋桨几何形状的地面效应性能建模提供了一种纯粹的分析方法。然而,作者没有探索多个相邻转子之间的潜在相互作用。一项实验研究[84]评估了方程式(2)对小型悬停转子(6至20英寸)的适用性。结果表明,该表达式对小型孤立螺旋桨也是一个很好的近似值。在后续出版物[82]中,开发了一种方法,可以生成多个物体环境中的空气动力学效应图。首先,沿着二维网格对靠近表面的空气动力学效应进行了实验测试(图13a)。然后,将数据组合在一起,以解释空气动力学图中的不同对象(图13b)。在同一条线上,Bernard等人[85]进行了一项广泛的实验活动,旨在表征小型四旋翼飞机的地面效应特性。特别是,对独立转子和完整平台进行了研究。将收集的结果与Cheeseman-Bennet模型和Hayden模型进行了比较,结果表明,地面的影响几乎扩展到z/R=4。Cony-ers等人[86]更进一步,报告了来自典型x形四旋翼框架上两个不同推进器间距的T/T∞的经验数据。结果表明,方程(2)不能准确预测四旋翼机的地面效应性能。Conyers[87]在他的论文中提供了不同螺旋桨尺寸和螺旋桨间距的更多经验数据。这些数据用于拟合T/T∞的有理多项式,作为归一化高度x=z/R、螺旋桨间距L和螺旋桨半径的函数。折衷方案是使用最低阶的方程,同时仍能产生质量拟合。最终的方程形式由下式定义:


To prevent potential issues such as selection bias or over f itting, all coefficients in Eq. (19) were obtained from a cross-validation procedure, from which the mean accuracy of the model was found to be within the generally adequate level of ±5%. However, the author did not provide compar isons between the model output and measurements obtained underdifferent conditions (radius and spacing) from theones used in the cross-validation procedure.
为了防止选择偏差或过度拟合等潜在问题,方程式(19)中的所有系数都是通过交叉验证程序获得的,从中发现模型的平均精度在±5%的一般适当水平内。然而,作者没有提供模型输出与在不同条件下(半径和间距)从交叉验证程序中使用的理论获得的测量值之间的比较。
Experience-based models have been developed for capturing the GE. Ryan and Kim [88] demonstrated how a vision system can be used to provide information about the GE using environmental cues. The main idea is that streamers attached to the ground will move around faster due to the pressurized airflow causing the GE. Then, the ground cushion force was learned with an-support vector regression model using empirical data. Another study demonstrated that the modular recurrent neural network framework is capable of modeling a multi-input-multi-output model of a quadrotor in the presence of GE [89]. The GE can also be acquired through data-driven or low-dimensional stochastic models [90]. Karydis and Hsieh [91] derived a data-driven model based on principal orthogonal decomposition to cap ture the GE for a hovering quadcopter. This model closely predicts the sample mean of the testing dataset. Karydis et al. [92] proposed a systematic approach that extends a deterministic model to a stochastic regime. With this, they developed a reduced stochastic model for a quadrotor which captures the mean and variance of the empirical data at all setpoints in steady state.
已经开发了基于经验的模型来捕捉地面效应。Ryan和Kim[88]演示了如何使用视觉系统来使用环境线索提供有关地面效应的信息。其主要思想是,由于加压气流导致地面效应,附着在地面上的拖缆将更快地移动。然后,使用经验数据,通过支持向量回归模型学习地面缓冲力。另一项研究表明,模块化递归神经网络框架能够在地面效应存在的情况下对四转子的多输入多输出模型进行建模[89]。地面效应也可以通过数据驱动或低维随机模型获取[90]。Karydis和Hsieh[91]导出了一个基于主正交分解的数据驱动模型,以覆盖悬停四旋翼机的地面效应。该模型精确地预测了测试数据集的样本均值。Karydis等人[92]提出了一种系统方法,将确定性模型扩展到随机状态。有了这个,他们为四旋翼开发了一个简化的随机模型,该模型在稳态下捕获了所有设定点的经验数据的均值和方差。
In the classical rotorcraft literature, the GE is studied in static conditions, in essence, for a fixed rotor. Even when a non-fixed rotorcraft is analyzed, the data are collected once the vehicle is at a stable hovering state. The effect of distance from the ground on the dynamics of the attitude motion is ignored. This was the main concern of Aich et al. [93]who reported a frequency-domain analysis of the roll dynamics as a function of height for a non-fixed quadcopter. A slow down of attitude dynamics as the distance from the ground decreases is registered. Closely related, Del Cont Bernard et al. [94] identified second-order models of the pitch dynam ics of a quadrotor (operated on a single degree of freedom testbed, representative of actual near hovering condition). These models show a dependence with respect to the height from the ground, justifying the degradation of the attitude control effectiveness in near-ground flight.
在经典旋翼飞机文献中,地面效应是在静态条件下研究的,本质上是针对固定旋翼。即使在分析非固定旋翼飞行器时,一旦飞行器处于稳定的悬停状态,数据也会被收集。忽略了离地距离对姿态运动动力学的影响。这是Aich等人[93]的主要关注点,他们报告了非固定四轴飞行器滚转动力学随高度变化的频域分析。随着离地面距离的减小,姿态动力学减慢。密切相关的是,Del Cont-Bernard等人[94]确定了四旋翼桨距动力学的二阶模型(在单自由度试验台上运行,代表实际的近悬停状态)。这些模型显示了对离地高度的依赖性,证明了近地飞行中姿态控制效果的下降。
When a rotorcraft flies past over an object, it is disturbed by the GE. Bartholomew et al. [95] proposed a system for predicting the acceleration expected when a quadcopter flies over anobject. They use Gaussian process regression to learn a function that receives a depth image and yields a vertical acceleration. The qualitative results show that the predictor output is similar to the ground truth for several seen objects and just one unseen object. Also,the authors present quantita tive results (average error), which they used only to compare the error for the same obstacle in different data-sets. The quantitative results provide the error mean for four unseen objects. Since the approach does not consider the velocity of the drone, it is limited to predict accelerations which are valid for a small vicinity around the training flight veloc ity. In a later publication [12], the same authors incorporated the prediction system into the flight control loop. To target the undisrupted level (forward) flight, they use the predic tions to adjust the throttle command when the drone passes over an object. The results display significant improvements for the box and step objects. On the other hand, the system was not able to compensate for the disturbance resulting from one already seen object and over compensated for two unseen objects, even though they were similar to the box obstacle.
当旋翼飞机飞过物体时,它会受到地面效应的干扰。Bartholomew等人[95]提出了一种预测四旋翼飞机飞越物体时预期加速度的系统。他们使用高斯过程回归来学习一个函数,该函数接收深度图像并产生垂直加速度。定性结果表明,对于几个可见物体和一个不可见物体,预测器输出与地面真实值相似。此外,作者还提供了定量结果(平均误差),他们仅用于比较不同数据集中同一障碍物的误差。定量结果提供了四个看不见物体的误差均值。由于该方法没有考虑无人机的速度,因此仅限于预测在训练飞行速度附近的小范围内有效的加速度。在后来的出版物[12]中,同样的作者将预测系统纳入了飞行控制回路。为了瞄准无中断的水平(向前)飞行,他们使用预测来调整无人机飞越物体时的油门指令。结果显示,框和步骤对象有了显著改进。另一方面,该系统无法补偿一个已经看到的物体造成的干扰,也无法过度补偿两个看不见的物体,即使它们与盒子障碍物相似。
As discussed before, experimental results with small quadcopters suggest that the GE may be larger than predicted by Eq.(2). However, the issue had not been further analyzed. Sanchez-Cuevas et al. [96] provided an expression of the thrust increment due to the GE for coplanar quadrotors, which has been modeled similar to full-scale helicopters (Cheese manand Bennet).To derive this expression, they use potential flow and the method of images, so that the model considers one source located at the geometric center of each rotor and its corresponding image source. Also, they include an addi tional term with an empirical coefficient, kb, to account for flow recirculation and the central body lift it generates. The expression has the following form:
如前所述,小型四旋翼机的实验结果表明,地面效应可能比公式(2)预测的要大。然而,该问题尚未得到进一步分析。Sanchez-Cuevas等人[96]提供了由于地面效应对共平面四旋翼机的推力增量的表达式,该四旋翼机已被建模为类似于全尺寸直升机(Cheesemanand Bennet)。为了推导这个表达式,他们使用了势流和图像方法,因此模型考虑了位于每个转子几何中心的一个源及其相应的图像源。此外,它们还包括一个带有经验系数kb的附加项,用于解释流量再循环及其产生的中心体升力。表达式具有以下形式:

where d is the separation from each rotor axis to its adja cent rotor axes and b is the distance between two opposite rotor axes (diagonal). The authors simulated two flight situ ations involving an object with a flat upmost surface, refer to Fig. 14. In both cases, the lateral axis (y-coordinate) of the drone remained constant, and the setpoint changed along the frontal axis (x-coordinate). Furthermore, they compared the response of three control alternatives: a conventional scheme with cascaded PID controllers, a scheme with a torque dis turbance observer in the attitude loop, and a scheme that uses a measurement of the relative distance from each rotor to the planar surface for implementing a feedforward control term in the attitude loop. Little details are provided about the sim ulation of the GE, but it seems that the effect is accounted for each rotor as a discontinuous event that is triggered when a rotor’s center lies over the planar object. By examining the x coordinate andpitchangleofthedrone,theauthorsfoundthe best response for the feedforward scheme, followed by the one with the disturbance observer. Then, all control alterna tives were assessed in a test bench, in which a quadrotor was restricted to only rotate around the lateral axis (pitch angle). In this setup, the partial GE was introduced by placing a large plate under one of the rotors. Again, the results showcased the superior performance of the feedforward scheme over the one with the disturbance observer, and of the latter over the conventional one. Lastly, the same (partial GE) test was reproduced during normal flight only with the standard PID control scheme, showing that the disturbance in the pitch angle was similar to the one observed in the test bench. Nev ertheless, the magnitude of the disturbance was greater in normal flight than in the test bench when the plate is retired. Equation (20) was acknowledged in a book chapter about aerodynamic effects in aerial manipulation [97].
其中d是从每个转子轴到其相邻转子轴的间距,b是两个相对转子轴(对角线)之间的距离。作者模拟了两个飞行位置,涉及一个最上面表面平坦的物体,见图14。在这两种情况下,无人机的横轴(y坐标)保持不变,设定点沿纵轴(x坐标)变化。此外,他们比较了三种控制方案的响应:一种具有级联PID控制器的传统方案,一种在姿态回路中具有扭矩扰动观测器的方案,以及一种使用每个转子到平面的相对距离的测量值在姿态回路中实现前馈控制项的方案。关于地面效应的模拟,几乎没有提供任何细节,但似乎每个转子的影响都被视为一个不连续事件,当转子的中心位于平面物体上时触发。通过检查锥体的x坐标和倾斜角,作者找到了前馈方案的最佳响应,其次是带有扰动观测器的方案。然后,在试验台上对所有控制替代品进行了评估,其中四旋翼仅限于围绕横轴(俯仰角)旋转。在这种设置中,部分地面效应是通过在其中一个转子下放置一块大板来引入的。结果再次表明,前馈方案的性能优于带有干扰观测器的方案,后者也优于传统方案。最后,在正常飞行期间,仅使用标准PID控制方案再现了相同的(部分地面效应)试验,表明俯仰角的扰动与在试验台上观察到的扰动相似。尽管如此,当板退役时,正常飞行中的干扰幅度大于试验台上的干扰幅度。方程式(20)在一本关于空中操纵气动效应的书中得到了认可[97]。
Evidence has been reported about how the GE varies for different types of surfaces. Keshavarzian and Danesjou [98] presented a modified model of the GE for all flight types near the ground that considers a surface quality coefficient. The authors claimed that the ground surface plays a significant role in the GE. The maximum value of GE occurs at flat and hard surfaces. On the other hand, it is less over uneven sur faces. The previous claim was confirmed for two cases: flat concrete and grass. They took measurements of differential pressure below a hovering quadrotor, over each surface and at different heights. It was observed that the values measured over flat concrete were higher than the ones over grass. More pressure means less induced velocity, which translates to a greater thrust ratio in GE. Therefore, the GE is greater over f lat concrete than over grass. Then, by simplifying the rela tionship between induced velocity and aircraft speed, and considering Eq. (4), the proposed model is
关于地面效应如何因不同类型的表面而变化的证据已经报道。Keshavarzian和Danesjou[98]针对地面附近的所有飞行类型提出了一个修正的地面效应模型,该模型考虑了表面质量系数。作者认为,地面在地面效应中起着重要作用,地面效应的最大值出现在平坦和坚硬的地面上。另一方面,表面不平整的情况较少。之前的索赔得到了两起案件的证实:平地混凝土和草地。他们测量了悬停四旋翼机下方、每个表面和不同高度的压差。观察到在平坦混凝土上测量的值高于在草地上测量的数值。压力越大,诱导速度越小,这意味着地面效应的推力比越大。因此,地面效应在混凝土上比在草地上更大。然后,通过简化诱导速度和飞机速度之间的关系,并考虑方程(4),提出的模型为

where ks,x,and y are the surface quality coefficient,position of the drone along the inertial x-axis, and position along the inertial y-axis, respectively.Relying on this model, the authors designed a backstepping control scheme for trajectory tracking that considers Eq.(21) in the computations of the control inputs.The control scheme was evaluated in simulations with noise,but no further validation of Eq.(21)was presented.
其中ks、x和y分别是表面质量系数、无人机沿惯性x轴的位置和沿惯性y轴的位置。基于该模型,作者设计了一种用于轨迹跟踪的反推控制方案,在计算控制输入时考虑了方程(21)。在有噪声的模拟中对控制方案进行了评估,但没有对方程(21)进行进一步验证。
Shortly after,Kanet al. [99] investigated thebehavior of small-scale rotorcrafts in close proximity to the ground both at hover and in forward flight. Performing an extensive experimental study, the authors found that existing models for helicopters cannot fully describe the forward motion of a quadcopter when it operates close to the ground. Consequently, they introduced two new data-driven models for quadrotors operating in GE, both at hover and in forward flight. Two variants were provided for each model: low/medium speed and high speed. The twomodels are described in Eqs.(22)–(23),in which the low-/medium-speed variants are denoted as “a,” and the high-speed variants as “b.” There quired thrust to hover is denoted as Th (under ideal conditions it is equal to mg/4 for a quadrotor), and the aircraft speed as V.
不久之后,Kanet等人[99]研究了小型旋翼机在悬停和向前飞行时靠近地面的行为。作者进行了广泛的实验研究,发现现有的直升机模型无法完全描述四轴飞行器在接近地面时的前进运动。因此,他们为在地面效应运行的四旋翼机引入了两种新的数据驱动模型,包括悬停和向前飞行。每种型号都提供了两种变体:低速/中速和高速。这两个模型在方程式(22)-(23)中进行了描述,其中低速/中速变体表示为“a”,高速变量表示为“b”。悬停所需的推力表示为Th(在理想条件下,四旋翼等于mg/4),飞机速度表示为V。

It should be mentioned that the models above,which were obtained by fitting data from an AscTec Hummingbird quadrotor (54cmmotor-to-motor),were validated with data collected from a Bitcraze Crazyflie2.0 (9.2cmmotor-to motor). The results show that the proposed models are able to predict T/T∞ for another quadrotor of differentsize,mass, controller,and trajectories.
应该提到的是,上述模型是通过拟合AscTec蜂鸟四旋翼(54cm电机到电机)的数据获得的,并用从Bitcraze Crazyflie2.0(9.2cm电机到发动机)收集的数据进行了验证。结果表明,所提出的模型能够预测另一个不同尺寸、质量、控制器和轨迹的四旋翼的T/T∞。
Considering the models discussed throughout the paper, we classified the GE models as shown in Table2.
考虑到本文中讨论的模型,我们对地面效应模型进行了分类,如表2所示。

3.3 Applications
The authors in [80] took advantage of the GE to perform a blind terrain mapping.
The idea is to fly a quadrotor at a constant height and slow forward velocity over the terrain to be mapped while recording the average rotors speed and x-y position.
Using an equivalent approach, a sensorless surface detection and estimation procedure is proposed in [10].
Using an equivalent approach, a sensorless surface detection and estimation procedure is proposed in [10]. Here, the estimated height from the ground is computed as a function of the throttle command of a quadcopter (previously obtained from experimental data). This estimation is used first to detect the ground, and later to land the drone. Two experiments were reported in which a quadcopter is able to land autonomously using this strategy. Nevertheless, the estimation is shown to be reliable only when the height from the ground is below 10 cm, which could lead to a crash. In the same work, ground and ceiling surfaces are considered to achieve energy-efficient path planning. From a heuristic energy relation, the authors demonstrated that it is possible to save about 10% when flying in proximity to horizontal surfaces.
利用近地面飞行可以节能10%
4 Other proximity effects
The second most mentioned proximity interaction is the Ceiling Effect (CE) [54,79,82,87, 97], which emerges when a rotorcraft is approaching from below to a horizontal surface.

























 被折叠的 条评论
为什么被折叠?
被折叠的 条评论
为什么被折叠?








