【图像处理】利用种子填充法对二值图像进行连通域标记-计算目标中心位置方法2

种子填充法原理

关于种子填充法的详细原理可以参考OpenCV_连通区域分析（Connected Component Analysis/Labeling）

大致算法如下： 设二值化图像A中，像素值为255的点是前景，为0的点是背景。A(x, y)为坐标(x, y)处的像素值，遍历图像的每个像素： 1、 如果像素值不等于255，则继续访问下一个元素。 2、 如果像素值为A(x, y) = 255，则新建一个新的label，当前值A(x, y) = label，并且

a. 检查其4个邻域，如果有属于前景的像素也给它赋予label值，并将它的坐标压栈。

b. 弹出栈顶坐标，重复a的过程，知道堆栈为空。 
此时，便找到了一个连通区域，该区域内的像素值被标记为label。

3、 重复1、2的过程，检测出所有的区域。

废话少说，上代码！

实现程序

该程序基于OpenCV2.4.9 和VS2010平台：

#include <opencv2\opencv.hpp>
#include <iostream>
#include <vector>
#include <stack>
using namespace std;
using namespace cv;

typedef struct _Feather
{
    int label;              // 连通域的label值
    int area;               // 连通域的面积
    Rect boundingbox;       // 连通域的外接矩形框
} Feather;

/* 
Input: 
    src: 待检测连通域的二值化图像
Output:
    dst: 标记后的图像
    featherList: 连通域特征的清单
return： 
    连通域数量。
*/
int bwLabel(Mat & src, Mat & dst, vector<Feather> & featherList)
{
    int rows = src.rows;
    int cols = src.cols;

    int labelValue = 0;
    Point seed, neighbor;
    stack<Point> pointStack;    // 堆栈

    int area = 0;               // 用于计算连通域的面积
    int leftBoundary = 0;       // 连通域的左边界，即外接最小矩形的左边框，横坐标值，依此类推
    int rightBoundary = 0;
    int topBoundary = 0;
    int bottomBoundary = 0;
    Rect box;                   // 外接矩形框
    Feather feather;

    featherList.clear();    // 清除数组

    dst.release();
    dst = src.clone();
    for( int i = 0; i < rows; i++)
    {
        uchar *pRow = dst.ptr<uchar>(i);
        for( int j = 0; j < cols; j++)
        {
            if(pRow[j] == 255)
            {
                area = 0;
                labelValue++;           // labelValue最大为254，最小为1.
                seed = Point(j, i);     // Point（横坐标，纵坐标）
                dst.at<uchar>(seed) = labelValue;
                pointStack.push(seed);

                area++;
                leftBoundary = seed.x;
                rightBoundary = seed.x;
                topBoundary = seed.y;
                bottomBoundary = seed.y;

                while(!pointStack.empty())
                {
                    neighbor = Point(seed.x+1, seed.y);
                    if((seed.x != (cols-1)) && (dst.at<uchar>(neighbor) == 255))
                    {
                        dst.at<uchar>(neighbor) = labelValue;
                        pointStack.push(neighbor);

                        area++;
                        if(rightBoundary < neighbor.x)
                            rightBoundary = neighbor.x;
                    }

                    neighbor = Point(seed.x, seed.y+1);
                    if((seed.y != (rows-1)) && (dst.at<uchar>(neighbor) == 255))
                    {
                        dst.at<uchar>(neighbor) = labelValue;
                        pointStack.push(neighbor);

                        area++;
                        if(bottomBoundary < neighbor.y)
                            bottomBoundary = neighbor.y;

                    }

                    neighbor = Point(seed.x-1, seed.y);
                    if((seed.x != 0) && (dst.at<uchar>(neighbor) == 255))
                    {
                        dst.at<uchar>(neighbor) = labelValue;
                        pointStack.push(neighbor);

                        area++;
                        if(leftBoundary > neighbor.x)
                            leftBoundary = neighbor.x;
                    }

                    neighbor = Point(seed.x, seed.y-1);
                    if((seed.y != 0) && (dst.at<uchar>(neighbor) == 255))
                    {
                        dst.at<uchar>(neighbor) = labelValue;
                        pointStack.push(neighbor);

                        area++;
                        if(topBoundary > neighbor.y)
                            topBoundary = neighbor.y;
                    }

                    seed = pointStack.top();
                    pointStack.pop();
                }
                box = Rect(leftBoundary, topBoundary, rightBoundary-leftBoundary, bottomBoundary-topBoundary);
                rectangle(src, box, 255);
                feather.area = area;
                feather.boundingbox = box;
                feather.label = labelValue;
                featherList.push_back(feather);
            }
        }
    }
    return labelValue;
}

int main(int argc, char *argv[])
{
    Mat src(imread("shape.jpg", 0));
    if(src.empty())
        exit(-1);
    threshold(src, src, 127, 255, THRESH_BINARY);   // 二值化图像
    vector<Feather> featherList;                    // 存放连通域特征
    Mat dst;
    cout << "连通域数量： " << bwLabel(src, dst, featherList) << endl;

    // 为了方便观察，可以将label“放大”
    for( int i = 0; i < dst.rows; i++)
    {
        uchar *p = dst.ptr<uchar>(i);
        for( int j = 0; j < dst.cols; j++)
        {
            p[j] = 30*p[j];
        }
    }

    cout << "标号" << "\t" << "面积" << endl;
    for(vector<Feather>::iterator it = featherList.begin(); it < featherList.end(); it++)
    {
        cout << it->label << "\t" << it->area << endl;
        rectangle(dst, it->boundingbox, 255);
    }

    imshow("src", src);
    imshow("dst", dst);

    waitKey();
    destroyAllWindows();

    system("pause");
    return 0;
}

 
 
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57
58
59
60
61
62
63
64
65
66
67
68
69
70
71
72
73
74
75
76
77
78
79
80
81
82
83
84
85
86
87
88
89
90
91
92
93
94
95
96
97
98
99
100
101
102
103
104
105
106
107
108
109
110
111
112
113
114
115
116
117
118
119
120
121
122
123
124
125
126
127
128
129
130
131
132
133
134
135
136
137
138
139
140
141
142
143
144
145
146
147
148
149
150
151
152
153
154
155
156
157
158
159
160
161
162
163

运行结果：

原图： 

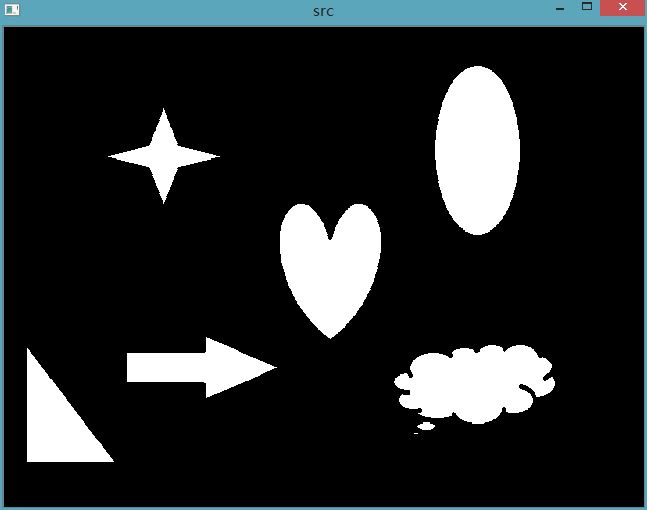
检测结果： 

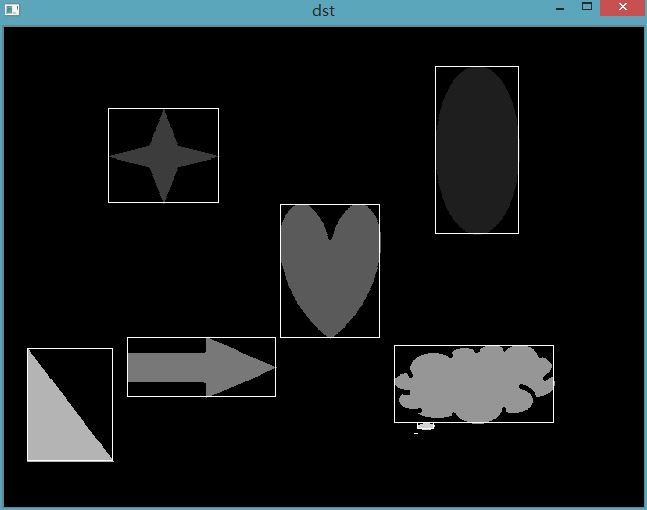
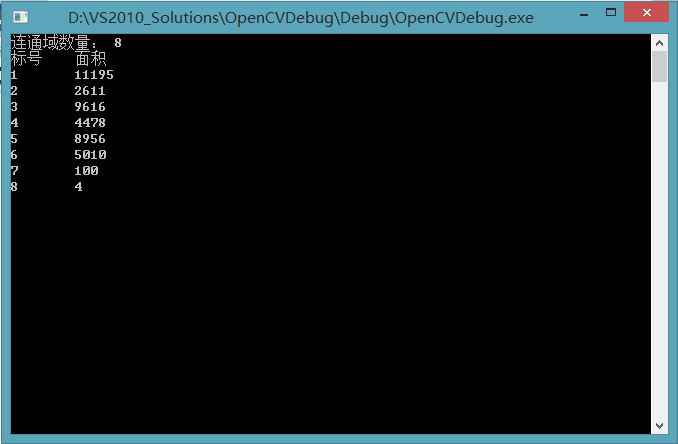
特征清单：